Definition, Formula, Characteristics, Solved Example Problems - Rectangular or Uniform Distribution | 11th Statistics : Chapter 10 : Probability Distributions
Chapter: 11th Statistics : Chapter 10 : Probability Distributions
Rectangular or Uniform Distribution
Continuous distributions:
Rectangular
or Uniform Distribution
Definition
A random variable X is said to have a
continuous Uniform distribution over the interval (a, b) if its probability
density function is

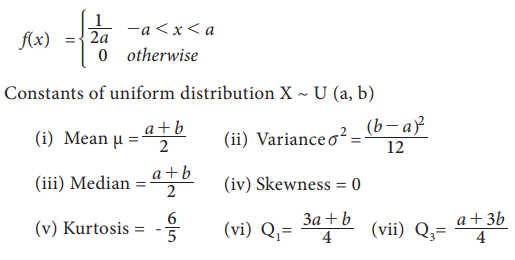
Characteristics of Uniform Distribution
i. a and b are the parameters of the Uniform
distribution and we write X ~ U (a, b)
ii.
The distribution is also known as Rectangular distribution, as the curve
iii. y = f(x) describes a rectangle over the
x-axis and between ordinates at x = a and x= b.
(iv)
f X ~ U (-a, a) then its p.d.f. is
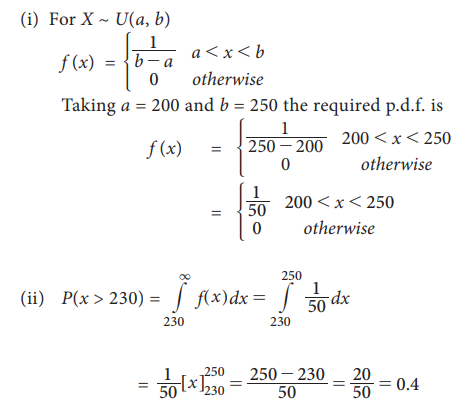
Example 10.17
If
X ~ U (200, 250) find its p.d.f and P
(X > 230)
Solution:
Example 10.18
If
X is a Uniform variate with the parameter 50 and100, find
the mean, median and standard deviation.
Solution:
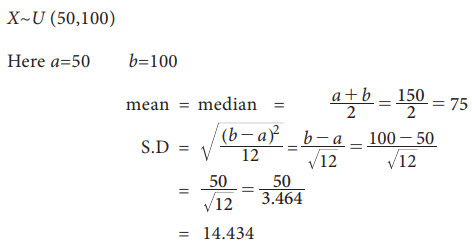
Example 10.19
If
X is a random variable having a uniform distribution U
(a,b) such that P(20<X<40)=0.2
and mean = 150, find a and b.
Solution:
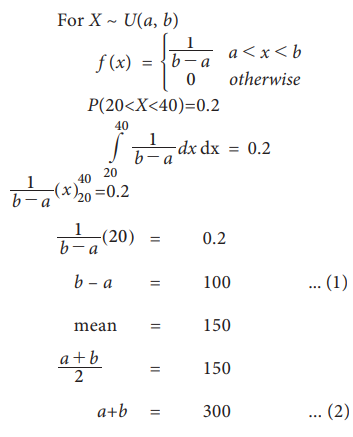
(1)+(2)
implies 2b = 400 and b = 200.
Substituting
b in (2) we have a + 200 = 300 and that a =100.
a=100,
b=200
X~U(100,200)
Example 10.20
If
X is a Uniform variable U(a,b) with first and third quartiles
100 and 200, find the p.d.f of X.
Solution:
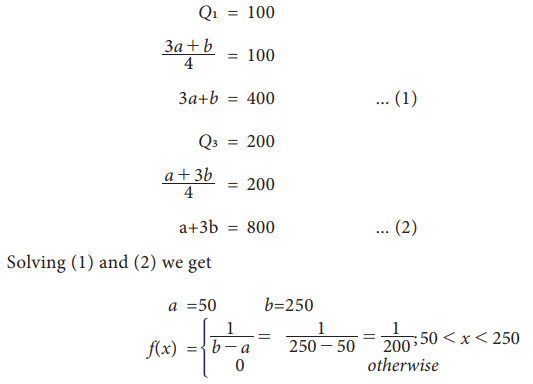
Example 10.21
Electric trains on a certain line run every 15 minutes between
mid- night and six in the morning. What is the probability that a man entering
the station at a random time during this period will have to wait at least ten
minutes?
Let the random variable X denote the waiting time (in minutes).
The given assumption indicates that X is distributed Uniformly
on (0,15).
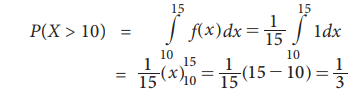
Related Topics