Prandtl’s Stress Function

Prandtl’s Stress Function

An alternative solution procedure that leads to a much simpler boundary condition, but a somewhat more difficult field equation, involves the intro-duction of a stress function Ï•(x,y) defined as:

By this definition, the equilibrium Equation (9.10) is automatically solved and the boundary condition Equation (9.15) becomes:

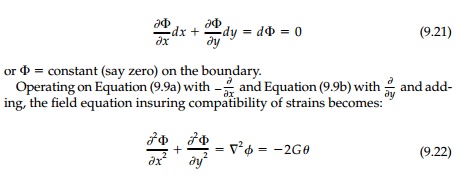
As noted in previews, this is a Poisson equation for which a solution can always be found and the boundary condition

is particularly nice.

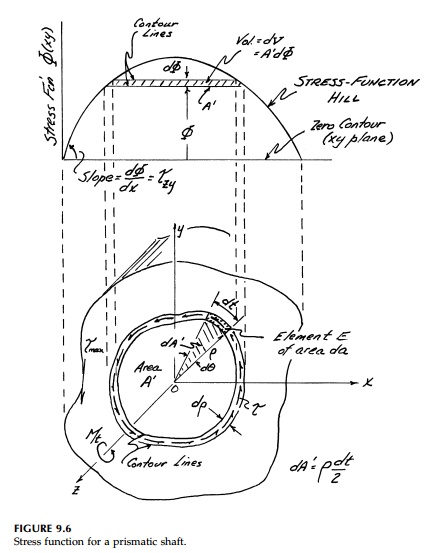
However, the power of the Prandtl stress function approach is in visualiz-ing torsional shear fields. It is easy to show that the stress function Ï• will transform in any direction as an invariant scalar function, and therefore in a new coordinate orientation x', y'

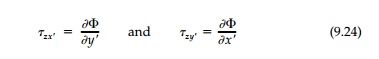
Thus at any point the shear stress in one direction is equal in magnitude to the slope of the  Ï• surface in the perpendicular direction, and the maximum shear is the maximum slope of the stress function curve and acts tangent (along a contour) to that curve. If we visualize the stress function plotted in the z direction above the cross-section, then contours (of equal Ï•) can be plot-ted and:

a.  The shear Ï„ acts along these contours and is proportional to the shortest distance (slope normal) to the next contour;

b. Ï„_max occurs where the stress-hill contours become closest togeth-er, which will be on the boundary of the cross-section (generally where r = Rt(x²+y²) is a minimum.

From Figure 9.6 it is easy to show that the twisting moment M_t is propor-tional to the volume of this stress-function hill. The shear force dS on a differ ential element E will be

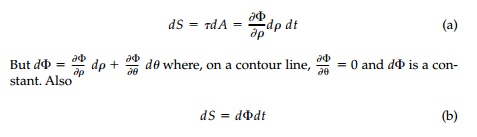
Therefore, between two contour lines the resisting moment

which is twice the volume of the stress-function hill.

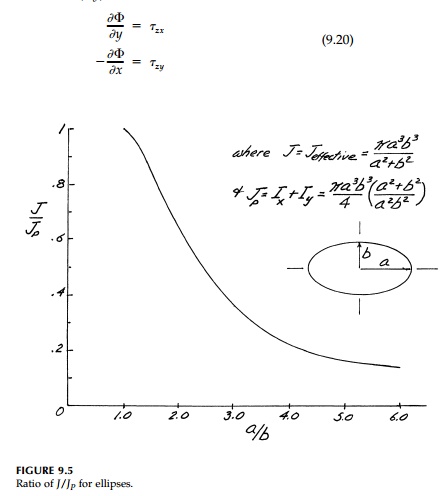
For example, consider the elliptic cross-section discussed previously. Assume a stress function

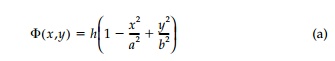
which must satisfy Equation (9.22), and the boundary condition Ï•_edge = 0. The volume of the stress-function hill (a paraboloid) is 0.5 Ï€ abh and therefore:

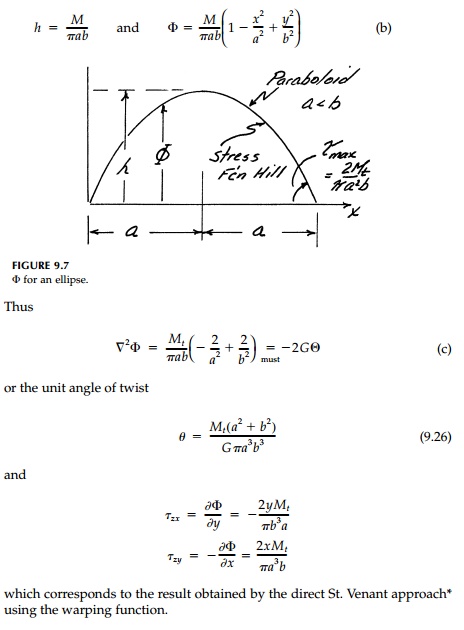
which corresponds to the result obtained by the direct St. Venant approach* using the warping function.