Chapter: Mechanical : Engineering Thermodynamics : Gas Mixtures and Psychrometry
P-v-T Behavior Of Gas Mixtures: Ideal And Real Gases
P-v-T BEHAVIOR OF GAS MIXTURES: IDEAL AND REAL GASES
An ideal gas is defined as a gas whose molecules are spaced far apart so that the behavior of a molecule is not influenced by the presence of other molecules—a situation encountered at low densities. We also mentioned that real gases approximate this behavior closely when they are at a low pressure or high temperature relative to their critical-point values. The P-v-T behavior of an ideal gas is expressed by the simple relation Pv _ RT, which is called the ideal-gas equation of state. The P-v-T behavior of real gases is expressed by more complex equations of state or by Pv _ ZRT, where Z is the compressibility factor.
When two or more ideal gases are mixed, the behavior of a molecule normally is not influenced by the presence of other similar or dissimilar molecules, and therefore a nonreacting mixture of ideal gases also behaves as an ideal gas. Air, for example, is conveniently treated as an ideal gas in the range where nitrogen and oxygen behave as ideal gases. When a gas mixture consists of real (nonideal) gases, however, the prediction of the P-v-T behavior of the mixture becomes rather involved.
The prediction of the P-v-T behavior of gas mixtures is usually based on two
models: Dalton’s law of additive pressures
Both models are described and discussed below.
Dalton’s law of additive pressures:
Dalton's law (also called Dalton's law of partial pressures) states that the total pressure exerted by a gaseous mixture is equal to the sum of the partial pressures of each individual component in a gas mixture. This empirical law was observed by John Dalton in 1801 and is related to the ideal gas laws. Mathematically, the pressure of a mixture of gases can be defined as the summation
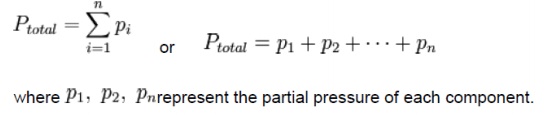
Amagat’s law of additive volumes:
Amagat's law or the Law of Partial Volumes of 1880 describes the behavior and properties of mixtures of ideal (as well as some cases of non-ideal) gases. Of use in chemistry and thermodynamics, Amagat's law states that the volume Vm of a gas mixture is equal to the sum of volumes Vi of the K component gases, if the temperature T and the pressure p remain the same:
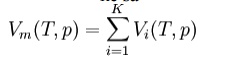
This is the experimental expression of volume as an extensive quantity. It is named after Emile Amagat. Both Amagat's and Dalton's Law predict the properties of gas mixtures. Their predictions are the same for ideal gases. However, for real (non-ideal) gases, the results differ. Dalton's Law of Partial Pressures assumes that the gases in the mixture are non-interacting (with each other) and each gas independently applies its own pressure, the sum of which is the total pressure. Amagat's Law assumes that the volumes of each component gas (same temperature and pressure) are additive; the interactions of the different gases are the same as the average interactions of the components.
Heat capacity
The heat capacity at constant volume of an ideal gas is:
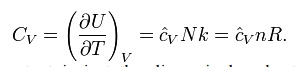
It is seen that the constant is just the dimensionless heat capacity at constant volume. It is equal to half the number of degrees of freedom per particle. For
moderate temperatures, the constant for a monoatomic gas is Cv = 3/2 while for
a diatomic gas it Is Cv = 5/2 . It is seen that macroscopic measurements on heat capacity provide information on the microscopic structure of the molecules.
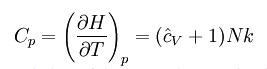
where H = U + pV is the enthalpy of the gas. It is seen that is also a constant and that the dimensionless heat capacities are related by:
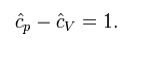
Related Topics