Measurements of Components

(i) Secular trend

It refers to the long term tendency of the data to move in an upward or downward direction. For example, changes in productivity, increase in the rate of capital formation, growth of population, etc ., follow secular trend which has upward direction, while deaths due to improved medical facilities and sanitations show downward trend. All these forces occur in slow process and influence the time series variable in a gradual manner.

Methods of Measuring Trend

Trend is measured using by the following methods:

1. Graphical method

2. Semi averages method

3. Moving averages method

4. Method of least squares

(ii) Seasonal variation

Seasonal variations are fluctuations within a year over different seasons.

Estimation of seasonal variations requires that the time series data are recorded at even intervals such as quarterly, monthly, weekly or daily, depending on the nature of the time series. Changes due to seasons, weather conditions and social customs are the primary causes of seasonal variations. The main objective of the measurement of seasonal variation is to study their effect and isolate them from the trend.

Methods of constructing seasonal indices

There are four methods of constructing seasonal indices.

1. Simple averages method

2. Ratio to trend method

3. Percentage moving average method

4. Link relatives method

Among these, we shall discuss the construction of seasonal index by the first method only.

(iii) Cyclical variation

Cyclical variations refer to periodic movements in the time series about the trend line, described by upswings and downswings. They occur in a cyclical fashion over an extended period of time (more than a year). For example, the business cycle may be described as follows.

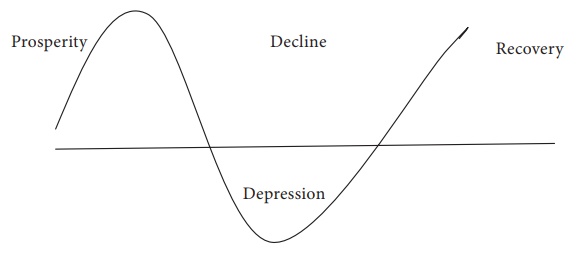
The cyclical pattern of any time series tells about the prosperity and recession, ups and downs, booms and depression of a business. In most of the businesses there are upward trend for some time followed by a downfall, touching its lowest level. Again a rise starts which touches its peak. This process of prosperity and recession continues and may be considered as a natural phenomenon.

(iv) Irregular variation

In practice, the changes in a time series that cannot be attributed to the influence of cyclic fluctuations or seasonal variations or those of the secular trend are classified as irregular variations.

In the words of Patterson, “Irregular variation in a time series is composed of non-recurring sporadic (rare) form which is not attributed to trend, cyclical or seasonal factors”.

Nothing can be predicted about the occurrence of irregular influences and the magnitude of such effects. Hence, no standard method has been evolved to estimate the same. It is taken as the residual left in the time series, after accounting for the trend, seasonal and cyclic variations.