Exercise 2.4

Exercise 2.4

Miscellaneous Practice problems

1. The base of the parallelogram is 16 cm and the height is 7 cm less than its base. Find the area of the parallelogram.

The base of the parallelogram b = 16 cm

                                    Height h = 7 cm less than its base

                                                 = (16 – 7) cm = 9 cm

Area of the parallelogram = b × h sq.unit

                                        = 16 × 9 sq.cm

                                       = 144 sq.cm

Area of the parallelogram = 144 sq.cm

2. An agricultural field is in the form of a parallelogram, whose area is 68.75 sq. hm. The distance between the parallel sides is 6.25 hm. Find the length of the base.

The area of the parallelogram = 68.75 sq.hm

 Height h = 6.25 h.m

 base b = ?

Area of the parallelogram b × h = 68.75

 b × 6.25 = 68.75

 b = 68.75 / 6.25 h.m

 b = 6875 / 625 h.m = 11 hm

The length of the base = 11 hm

3. A square and a parallelogram have the same area. If the side of the square is 48 m and the height of the parallelogram is 18 m, find the length of the base of the parallelogram.

Side of the square a = 48 m

Area of the square = a² = 48 × 48 sq.m 

                            = 2304 sq.m

Area of the parallelogram b × h = 2304 sq.m

height h = 18 m

base = Area / height = 2304 / 18 = 128 m

The length of the base = 128 m

4. The height of the parallelogram is one fourth of its base. If the area of the parallelogram is 676 sq. cm, find the height and the base.

Let base be b cm

The height of the parallelogram = 1/4 of it base = 1/4 × b = b/4 cm

Area of the parallelogram = b  × h sq. units

= b × b/4 = 676

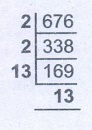
b × b = 676 × 4

= 2 × 2 × 13 × 13 × 2 × 2

b = 2 × 2 × 13 = 52 cm

 h = 1/4 × b =  1/4 × 52 = 13 cm

The height h = 13 cm

The base b = 52 cm

5. The area of the rhombus is 576 sq. cm and the length of one of its diagonal is half of the length of the other diagonal then find the length of the diagonals.

Length of one diagonal d₁ = d cm

Length of the other diagonal d₂ = 1/2 of the length of the first diagonal

                                                = 1/2 × d cm

Area of the rhombus = 1/2 d₁ × d₂ sq. units

 = 576 sq.cm

=1/2 × d × 1/2 × d = 576

d × d = 576 × 2 × 2

 = 2 × 2 × 12 × 12 × 2 × 2

 d = 2 × 2 × 12 = 48 cm

One diagonal d₁ = 48 cm

Other diagonal d₂ = 1/2 × 48 = 24 cm

The length of the diagonals d₁ = 48 cm

                                           d₂ = 24 cm

6. A ground is in the form of isosceles trapezium with parallel sides measuring 42 m and 36 m long. The distance between the parallel sides is 30 m. Find the cost of levelling it at the rate of ₹ 135 per sq.m.

The parallel sides a = 42 m

 b = 36 m

 height h = 30 m

Area of the isosceles trapezium = 1/2 h (a + b) sq. units

 = 1/2 × 30 (42 + 36) sq.m

= 15 × 78 sq.m

Area of the Trapezium = 1170 sq. m

The cost of levelling per sq.m = ₹ 135

The cost of levelling 1170 sq.m = ₹ 1170 × 135

                                                   = ₹ 1,57,950

The cost of levelling the ground = ₹ 1,57,950

Challenge Problems

7. In a parallelogram PQRS (see the diagram) PM and PN are the heights corresponding to the sides QR and RS respectively. If the area of the parallelogram is 900 sq. cm and the length of PM and PN are 20 cm and 36 cm respectively, find the length of the sides QR and SR.

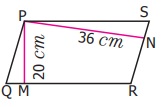
The area of the parallelogram b × h = 900 sq. cm

                                          height h = 20 cm

 base b = QR = Area / height = 900 / 20 = 45 cm

                                                        QR = 45 cm

Height h = 36 cm

base b = SR = Area / height = 900 / 36 = 25 cm

                                                       SR = 25 cm

The length of the side QR = 45 cm

The length of the side SR = 25 cm

8. If the base and height of a parallelogram are in the ratio 7:3 and the height is 45 cm then, find the area of the parallelogram.

The base and the height of a parallelogram are in the ratio = 7:3

Let the base b = 7x

The height h = 3x = 45 cm

 x = 45 / 3 = 15 cm

base b = 7x = 7 × 15 = 105 cm

The area of the parallelogram = b × h sq. units 

 = 105 × 45 sq.cm

 = 4725 sq.cm

The area of the parallelogram = 4725 sq.cm

9. Find the area of the parallelogram ABCD, if AC is 24 cm and BE = DF= 8 cm.

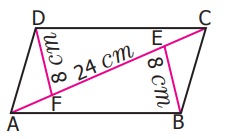
The area of the parallelogram ABCD = Area of Δ ABC + Aea of Δ ACD

1/2 × AC × DF + 1/2 × AC × BE sq. units

1/2 × AC (DF + BE) sq.units

1/2 × 24 (8+8) sq.cm

12 × 6 sq.cm = 192 sq.cm

Area of the parallelogram ABCD = 192 sq.cm

10. The area of the parallelogram ABCD is 1470 sq cm. If AB = 49 cm and AD = 35 cm then, find the heights DF and BE.

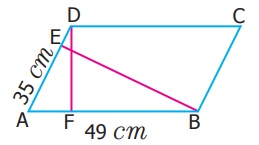
Area of the parallelogram ABCD

                                          = 1470 sq. cm

 Base AB = 49 cm

 Base × height = 1470

 49 × height =1470

 height = 1470 / 49 = 30 cm

 Height DF = 30 cm

 Base AD = 35 cm

 Base × height = 1470

 35 × height = 1470

  Height = 1470 / 35= 42 cm

 Height BE = 42 cm

Height DF = 30 cm

Height BE = 42 cm

11. One of the diagonals of a rhombus is thrice as the other. If the sum of the length of the diagonals is 24 cm, then find the area of the rhombus.

Let the diagonals be d₁ and d₂

d₁ = d cm

d₂ = thrice of the other diagonals

d₂ = 3 d cm

Sum of the length of the diagonals = d₁ + d₂

= d + 3d = 4d cm

 4d = 24

  d = 24 / 4 = 6 cm

 d₁ = 6 cm

 d₂ = 3d = 3 × 6 = 18 cm

The area of the rhombus = 1/2 d₁ × d₂ sq.cm

                                       = 1/2 × 6 × 18 = 54 sq. cm

Area of the rhombus = 54 sq.cm

12. A man has to build a rhombus shaped swimming pool. One of the diagonal is 13 m and the other is twice the first one. Then find the area of the swimming pool and also find the cost of cementing the floor at the rate of ₹ 15 per sq.cm.

Let the diagonals be d₁ and d₂

d₁ = 13 m

d₂ = 2d₁ = 2 × l3 m = 26 m

Area of the swimming pool = 1/2 d₁ × d₂ sq.units

= 1/2 × 13 × 26 = 169 sq.m

The cost of cementing the floor per sq.m = ₹ 15

The cost of cementing the floor 169 sq.m = 169 × 15 = ₹ 2535

The cost of cementing the floor = ₹ 2535

13. Find the height of the parallelogram whose base is four times the height and whose area is 576 sq. cm.

Let the height be h cm

base b = 4 times the height

 = 4 h cm

Area of the parallelogram = b  × h = 576 sq. cm

                              h × 4 h = 576

 h × h = 576 / 4 = 144 = 12 × 12

h = 12 cm

Height h = 12 cm

14. The table top is in the shape of trapezium with measurements given in the figure. Find the cost of the glass used to cover the table at the rate of ₹ 6 per 10 sq. cm.

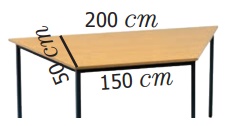
Parallel sides of the trapezium a = 150 cm

 b = 200 cm

 height h = 50 cm

Area of the trapezium = 1/2 h (a+b) sq units

 = 1/2 × 50 (150 + 200) sq.cm

= 25 × 350 sq.cm

= 8750 sq.cm

The scost of the glass per 10 sq.cm = ₹ 6

The scost of the glass per 8750 sq.cm = ₹ 6/10 × 8750

                                                             = ₹5250

The cost of the glass used to cover the table=₹5250

15. Arivu has a land ABCD with the measurements given in the figure. If a portion ABED is used for cultivation (where E is the mid-point of DC), find the cultivated area.

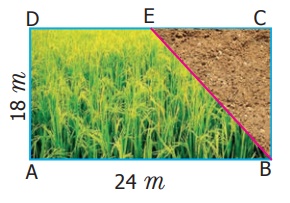
Answer:

Parallel sides AB = a = 24cm

DE = b = 12m

 height AD = h = 18 m

The cultivated area ABCD = 1/2 h(a+b) sq.units

                                         = 1/2 × 18 (24+12) sq.m

                                         = 9 × 36 =324 sq.m

        The cultivated area = 324 sq.m

ANSWERS

Exercise 2.4

1. 144 sq.cm

2. 11 hm

3. 128 m

4. h = 13cm b = 52cm

5. d₁ = 48 cm d₂ = 24cm

6. ₹ 1,57,950

 Challenge Problems

7. 45 cm; 25 cm

8. 4725 sq.cm

 9. 192 sq.cm

10. DF = 30cm BE = 42cm

11. 54 sq.cm

12. 169 sq.cm; ₹ 2535

13. 12 cm

14. ₹ 5250

15. 324 sq.m