Chapter: Mechanical : Finite Element Analysis : Two Dimensional Finite Element Analysis
Strain - Stress Relation
STRAIN – STRESS RELATION
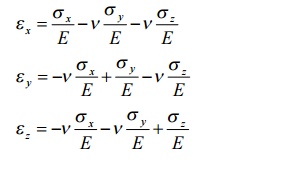
1 Plane stress conditions
since s z =0, from equations 1 and 2

2 Plane strain conditions
s x ,s y and t xy are present.
εz =γxz =γyz =0.
s z is not zero.
since εz =0, we get from equation 3
s z =n (sx +s x )
substituting s z in equations 1 and 2

It is difficult to represent the curved boundaries by straight edges element a large number of element may be used to obtain reasonable resembalance between original body and the assemblage
Two-Dimensional Problems
Review of the Basic Theory
In general, the stresses and strains in a structure consist of six components:
sx , sy , sz , txy , tyz , tzx for stresses,
ex , e y , ez , g,xy, g,yz , g,zx, for strains.
Under contain conditions, the state of stresses and strains can be simplified. A general 3-D structure analysis can, therefore, be reduced to a 2-D analysis.
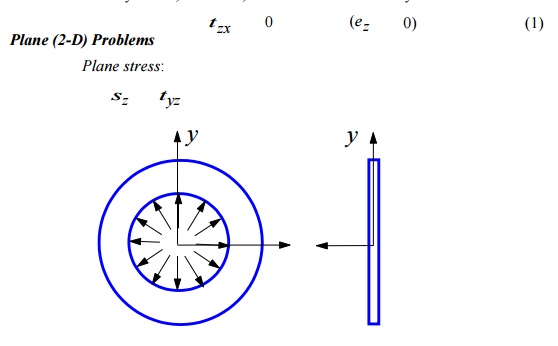
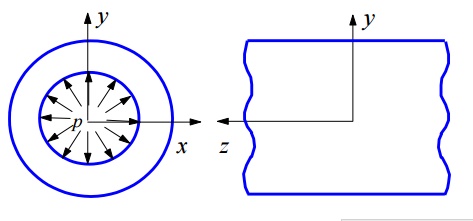
Plane strain:
A long structure with a uniform cross section and transverse loading along its length (z-direction).
Stress-Strain-Temperature (Constitutive) Relations
For elastic and isotropic materials, we have,

where e0 is the initial strain E the Young’s modulus, n the Poisson’s ratio and G the shear modulus. Note that,
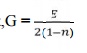
which means that there are only two independent materials constants for homogeneous and isotropic materials.
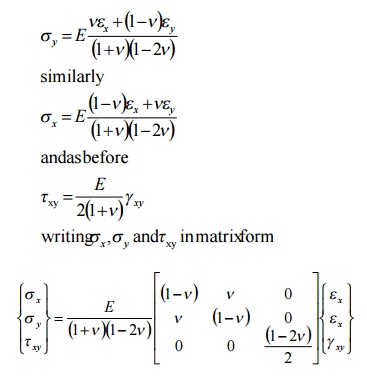
We can also express stresses in terms of strains by solving the above equation,
The above relations are valid for plane stress case. For plane strain case, we need to replace the material constants in the above equations in the following fashion,
For example, the stress is related to strain by
Initial strains due to temperature change (thermal loading) is given by,
where a is the coefficient of thermal expansion, T the change of temperature. Note that if the structure is free to deform under thermal loading, there will be no (elastic) stresses in the structure.
GENERALIZED COORDINATES APPROACH TO NODEL APPROXIMATIONS
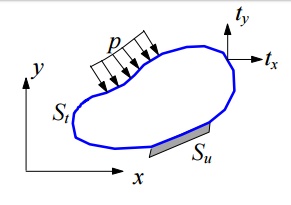
The boundary S of the body can be divided into two parts, Su and St. The boundary conditions (BC’s) are described as, in which tx and ty are traction forces (stresses on the boundary) and the barred quantities are those with known values.
In FEM, all types of loads (distributed surface loads, body forces, concentrated forces and moments, etc.) are converted to point forces acting at the nodes.
Exact Elasticity Solution
The exact solution (displacements, strains and stresses) of a given problem must satisfy the equilibrium equations, the given boundary conditions and compatibility conditions (structures should deform in a continuous manner, no cracks and overlaps in the obtained displacement field)
Related Topics