Chapter: 9th Science : Light
Speed of light
Speed of light
In early seventeenth century, the Italian scientist
Galileo Galilee (1564‒1642) tried to measure the speed of light as it travelled
from a lantern on a hill top about a mile (1.6 km) away from where he stood.
His attempt was bound to fail, because he had no accurate clocks or timing
instruments.
In 1665 the Danish astronomer Ole Roemer first
estimated the speed of light by observing one of the twelve moons of the planet
Jupiter. As these moons travel around the planet, at a set speed, it would take
42 hours to revolve around Jupiter. Roemer made a time schedule of the eclipses
for the whole year. He made first observation in June and second observation in
December. Roemer estimated the speed of light to be about 220,000 km per
second.
In 1849 the first land based estimate was made by
Armand Fizeau. Today the speed of light in vacuum is known to be almost exactly
300,000 km per second.
1. Refraction of light

2. Cause of refraction
Light rays get deviated from their original path
while entering from one transparent medium to another medium of different
optical density. This deviation (change in direction) in the path of light is
due to the change in velocity of light in the different medium. The velocity of
light depends on the nature of the medium in which it travels. Velocity of
light in a rarer medium (low optical density) is more than in a denser medium
(high optical density).
3. Refraction of light
from a plane transparent surface
When a ray of light travels from optically rarer
medium to optically denser medium, it bends towards the normal. (Figure 22)
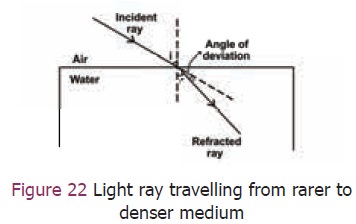
When a ray of light travels from an optically
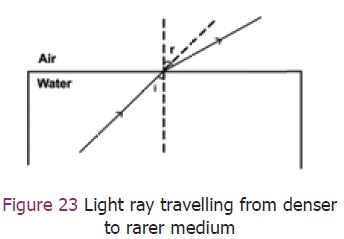
denser medium to an optically rarer medium it bends away from the normal.
(Figure 23)
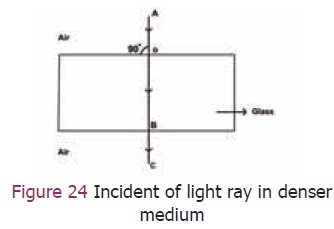
A ray of light incident normally on a denser
medium, goes without any deviation. (Figure 24).
4. The laws of refraction of light
The incident ray, the refracted ray and the normal
to the interface of two transparent media at the point of incidence, all lie in
the same plane.
The ratio of the sine of the angle of incidence to
the sine of the angle of refraction is a constant for a light of a given colour
and for the given pair of media. is law is also known as Snell’s law of
refraction.
If i is
the angle of incidence and r is the
angle of refraction, then
Sin i / sin r = constant
This constant is called the refractive index of the
second medium with respect to the first medium. It is generally represented by
the Greek letter, 1µ2 (mew).
Note: The refractive index has no unit as it is the
ratio of two similar quantities.
5. Verification of la s of refraction
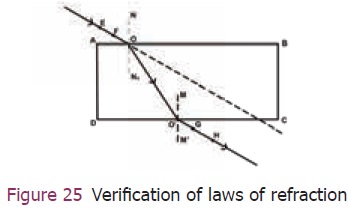


6. Speed of light in different media
Light has the maximum speed in vacuum and it
travels with different speeds in different media. The speed of light in some
media is given below.
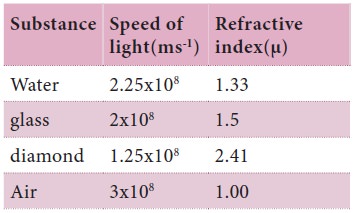
Note: The refractive index of a medium is also
defined in terms of speed of light in different media

Sample problem 4
The speed of light in air is 3 × 108 ms-1
and in glass it is 2 × 108 ms-1 what is the refractive
index of glass.
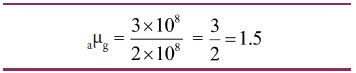
Sample problem 5
Light travels from a rarer medium to a denser
medium. The angles of incidence and refraction are respectively 45° and 30°.
Calculate the refractive index of the second medium with respect to the rst
medium.
Angle of incidence i = 45°
Angle of refraction r = 30°
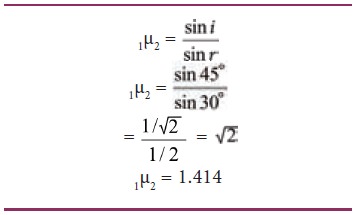
Related Topics