Finite Element Method (FEM) or Finite Element Analysis (FEA)

FINITE ELEMENT FORMULATION OF BOUNDARY VALUE PROBLEMS

INTRODUCTION

The finite element method constitutes a general tool for the numerical solution of partial differential equations in engineering and applied science.

The finite element method (FEM), or finite element analysis (FEA), is based on the idea of building a complicated object with simple blocks, or, dividing a complicated object into small and manageable pieces. Application of this simple idea can be found everywhere in everyday life as well as in engineering.

Examples:

Lego (kids’play) Buildings

Approximation of the area of a circle:

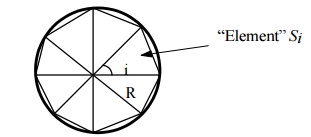
Why Finite Element Method?

·        Design analysis:  hand calculations, experiments, and computer simulations

·        FEM/FEA is the most widely applied computer simulation method in engineering

·        Closelyintegrated with CAD/CAM applications

1.A Brief History of the FEM

·        1943 --- Courant (variational method)

·        1956 --- Turner, clough, martin and top(stiffness)

·        1960 --- Clough (finite element plan problems)

·        1970 --- Applications on mainframe computer

·        1980 --- Microcomputers, pre and post processors

·        1990 --- Analysis of large structural systems

2.General Methods of the Finite Element Analysis

2. Force Method – Internal forces are considered as the unknowns of the problem.

3.                Displacement or stiffness method – Displacements of the nodes are considered as the unknowns of the problem.

3.General Steps of the Finite Element Analysis

·          Discretization of structure

·            Numbering of Nodes and Elements

·          Selection of Displacement function or interpolation function

·          Define the material behavior by using Strain – Displacement and Stress – Strain relationships

·          Derivation of element stiffness matrix and equations

·          Assemble the element equations to obtain the global or total equations

·          Applying boundary conditions

·          Solution for the unknown displacements computation of the element strains and stresses from the nodal displacements

·          Interpret the results (post processing).

4.Objectives of This FEM

·        Understand the fundamental ideas of the FEM

·        Know the behavior and usage of each type of elements covered in this course

·        Be able to prepare a suitable FE model for given problems

·        Can interpret and evaluate the quality of the results (know the physics of the problems)

·        Be aware of the limitations of the FEM (don’t misuse the

·        FEM - a numerical tool)

5.Applications of FEM in Engineering

·        Mechanical/Aerospace/Civil/Automobile Engineering Structure analysis (static/dynamic, linear/nonlinear) Thermal/fluid flows

·        Electromagnetics

·        Geomechanics

·        Biomechanics