Exercise 7.1: Binomial distribution

Exercise 7.1

1. Define Binomial distribution.

2. Define Bernoulli trials.

3. Dedrive the mean and variance of binomial distribution.

4. Write down the conditions for which the binomial distribution can be used.

5. Mention the properties of binomial distribution.

6. If 5% of the items produced turn out to be defective, then find out the probability that out of 10 items selected at random there are

(i) exactly three defectives

(ii) atleast two defectives

(iii) exactly 4 defectives

(iv) find the mean and variance

7. In a particular university 40% of the students are having news paper reading habit. Nine university students are selected to find their views on reading habit. Find the probability that

(i) none of those selected have news paper reading habit

(ii) all those selected have news paper reading habit

(iii) atleast two third have news paper reading habit.

8. In a family of 3 children, what is the probability that there will be exactly 2 girls?

9. Defects in yarn manufactured by a local mill can be approximated by a distribution with a mean of 1.2 defects for every 6 metres of length. If lengths of 6 metres are to be inspected, find the probability of less than 2 defects.

10. If 18% of the bolts produced by a machine are defective, determine the probability that out of the 4 bolts chosen at random

(i) exactly one will be defective

(ii) none will be defective

(iii) atmost 2 will be defective

11. If the probability of success is 0.09, how many trials are needed to have a probability of atleast one success as 1/3 or more ?

12. Among 28 professors of a certain department, 18 drive foreign cars and 10 drive local made cars. If 5 of these professors are selected at random, what is the probability that atleast 3 of them drive foreign cars?

13. Out of 750 families with 4 children each, how many families would be expected to have (i) atleast one boy (ii) atmost 2 girls (iii) and children of both sexes? Assume equal probabilities for boys and girls.

14. Forty percent of business travellers carry a laptop. In a sample of 15 business travelers,

(i) what is the probability that 3 will have a laptop?

(ii) what is the probability that 12 of the travelers will not have a laptop?

(iii) what is the probability that atleast three of the travelers have a laptop?

15. A pair of dice is thrown 4 times. If getting a doublet is considered a success, find the probability of 2 successes.

16. The mean of a binomial distribution is 5 and standard deviation is 2. Determine the distribution.

17. Determine the binomial distribution for which the mean is 4 and variance 3. Also find P(X=15)

18. Assume that a drug causes a serious side effect at a rate of three patients per one hundred. What is the probability that atleast one person will have side effects in a random sample of ten patients taking the drug?

19. Consider five mice from the same litter, all suffering from Vitamin A deficiency. They are fed a certain dose of carrots. The positive reaction means recovery from the disease. Assume that the probability of recovery is 0.73. What is the probability that atleast 3 of the 5 mice recover.

20. An experiment succeeds twice as often as it fails, what is the probability that in next five trials there will be (i) three successes and (ii) at least three successes

Answers:

6. (a) 0.059 (b) 0.2642 (c) 0.0133 (d) mean = 1 and variance = 0.95

7. (i) 0.01008 (ii) 0.00262 (iii) 0.09935

8. 0.375

9. 0.5767

10. (i) 0.3969 (ii) 0.45212 (iii) 0.9797 11. 5 or more trials

12. 0.7530

13. (i) 703 (ii) 516 (iii) 656

14. (i) 0.0634 (ii) 0.0634 (iii) 0.9729

15. 25/216 16.

16. 

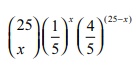
17. 3/4¹⁴

18. 0.2626

19. 0.8743

20. (i) 80/243 (ii) 192/243