Design of Gear Boxes

DESIGN OF GEAR BOXES

Standard progression

When the spindle speed are arranged in geometric progression then the ratio between the two adjacent speeds is known as step ratio or progression ratio.

Step ratio

Basic series : Step ratio (φ)

R5    : 1.58

R10  : 1.26

R20  : 1.12

R40  : 1.06

R80  : 1.03

Preferred basic series

Basic series          Preferred number

R5    (φ=1.6)       1.00, 1.60, 2.50, 4.00, 6.30,10.00

R10  (φ=1.26)     1.00, 1.25, 1.60, 2.00, 2.50, 3.15, 4.00, 5.00, 6.30, 8.00, 10.00

R20  (φ=1.12)     1.00, 1.06, 1.25, 1.18, 1.60, 1.25, 2.00, 2.24, 2.50, 2.80, 3.15, 3.55, 4.00, 4.50, 5.00, 5.60, 6.30, 7.10, 8.00, 9.00, 10.00

R40  (φ=1.06)     1.00, 1.06, 1.18, 1.25, 1.32, 1.18, 1.40,1.60, 1.70, 1.25,1.80, 1.90, 2.00, 2.10, 2.24, 2.36, 2.50, 2.65, 2.80, 3.00, 3.15, 3.35, 3.55, 4.00, 4.25, 4.50, 4.75, 5.00, 5.30, 5.60, 6.00, 6.30, 6.70, 7.10, 7.50, 8.00, 8.50, 9.00, 9.50, 10.00

Design of gear box

1.     Selection of Spindle speed:

Find the standard step ratio by using the relations,

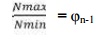
2.      Structural formula

It can be selected based on the number of speed:

Number of speed Structural formula

         3(1)   2(3)

6       2(1)   3(2)

         2(1)   2(2) 2(4)

8       4(1)   2(4)

         3(1)   3(3)

9               

         3(1)   2(3) 2(6)

12     2(1)   3(2) 2(6)

         2(1)   2(2) 3(4)

         3(1)   3(3) 2(5)

14     4(1)   2(4) 2(6)

15     3(1)   3(3) 2(6)

         4(1)   2(4) 2(8)

16     2(1)   4(2) 2(8)

         2(1)   2(2) 4(4)

         3(1)   3(3) 2(9)

18     3(1)   2(3) 3(6)

         2(1)   3(2) 3(6)

3.  Ray Diagram

The ray diagram is the graphical representation of the drive arrangement in general from. In other words, The ray diagram is the graphical representation of the structural formula.

The basic rules to be followed while designing the gear box as

ü Transmission ration (i):

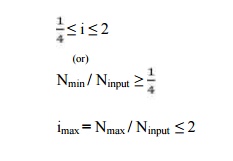
ü For stable operation the speed ratio at any stage should not be greater than 8.

Nmax / Nmin  ≤ 8

4.  Kinematic Layout:

The kinematic arrangement shows the arrangement of gears in a gear box.

Formula for kinematic arrangement,

n = p₁(X₁) . p₂(X₂)

5.     Calculation of number of teeth.

In each stage first pair,

Assume, driver Z_min  ≥ 17,

Assume Z = 20 (driver)

SOLVED PROBLEMS

1.     Design a 4- speed gear box for a machine. The speed vary approximately from 200 to 400 rpm. The input shaft speed is 600 rpm.

Sol:

ɸ  = ( Rn) ^1/z-1

Rn= 450/200 =2.25; Z=4

ɸ =2.25 ^0.334 =1.31

the nearest standard value of ɸ is 1.25

the speed of shafts are 180,224,280 and 355 rpm or 224,280,355,450 rpm T_a + T_b = T_c + T_d

N _a / N_b = T_a / T_b  =  355/600

N_d / N_c = T_c / T_d  =  450/600

Assuming minimum number of teeth on the smaller gear as 18( Ta =18)

18/ T_b  =  355/600

T_b = 30

T_a + T_b = 48 = T_c + T_d

T_c / T_d  =  450/600

T_c  = 20 and T_d = 28

Considering the transmission between the intermediate and output shafts

T_e+ T_f = T_g + T_h

N_f / N_e = T_e/ T_f  = 224/355

N _a / N_b = T_a / T_b  = 355/355

Assuming minimum number of teeth on the smaller gear as 20( Te =20)

20/ T_f  =  224/355

T_b = 32

T_e+ T_f  = 52 =  T_g + T_h

T_g / T_h  =  450/600

T_g = T_h  = 26

2. Design a gear box for a drilling machine to give speed variation between 100 and 560 rpm in six steps. The input shaft speed is 560 rpm. The intermediate shaft is to have three speeds.

Sol:

The progression ratio,

ɸ = ( Rn) ^1/z-1

Rn= 560/100 =5.6; Z=6

ɸ =5.6 ^0.2 =1.411

the nearest standard value of ɸ is 1.4

the speed of shafts are 100,140,200,280,400 and 560 rpm.

T_a + T_b = T_c + T_d = T_e+ T_f

N_b/ N_a = T_a/ T_b = 280/560

N_d / N_c= T_c/ T_d= 400/560

N_f / N_e = T_e/ T_f  = 560/560

Assuming minimum number of teeth on the smaller gear as 20( Ta =20)

20/ T_b = 280/560

T_b = 40

T_c/ T_d= 400/560

T_a + T_b = 60 = T_c + T_d

T_c  = 25 and T_d = 35

T_e/ T_f= 560/560 and  T_e + T_f = 60

T_e = T_f =30

Considering the transmission between the intermediate and output shafts

T_g + T_h= T_i+ T_j

N_h/ N_g = T_g/ T_h = 100/280

N_j / N_i= T_i/ T_j= 280/280

Assuming minimum number of teeth on the smaller gear as 20( Tg =20) 20/ T_h = 100/280

T_h = 56

T_i/ T_j= 280/280

T_i + T_j = 76 = T_g + T_h

T_i = T_j=38

2.     Design a nine speed gear box for a grinding machine with a minimum speed of 100 rpm and a maximum speed of 700 rpm. The motor speed is 1400 rpm. Determine the speed at which the input shaft is to be driven.

Sol:

The progression ratio,

ɸ = ( Rn) ^1/z-1

Rn= 700/100 =7; Z=9

ɸ =7 ^0.125 =1.275

The nearest standard value of ɸ is 1.25

The speeds of shafts are 112,140,180,224,280,355,450,560 and 710 rpm

T_a + T_b = T_c + T_d = T_e+ T_f

N_b/ N_a = T_a/ T_b = 224/560

N_d / N_c= T_c/ T_d= 280/560

N_f / N_e = T_e/ T_f  = 355/560

Assuming minimum number of teeth on the smaller gear as 24( Ta =24)

24/ T_b = 224/560

T_b = 60

T_c/ T_d= 280/560

T_a + T_b = 84 = T_c + T_d

T_c  = 28 and T_d = 56

T_e/ T_f= 355/560 and  T_e + T_f = 84

T_e = 33 and T_f =51

Considering the transmission between the intermediate and output shafts

T_g + T_h= T_i+ T_j= T_k+ T_l

N_h/ N_g = T_g/ T_h =112/224

N_j / N_i= T_i/ T_j= 224/224

N_l/ N_k= T_k/ T_l= 450/224

Assuming minimum number of teeth on the smaller gear as 20( Tg =20) 20/ T_h = 112/224

T_h = 40

T_i/ T_j= 224/224

T_i + T_j = 60 = T_g + T_h

T_i = T_j=30

T_k/ T_l= 450/224

T_k+ T_j = 60

T_k=40 and T_l=20

4. Design an all geared speed gear box for a radial machine, with the following specifications:

Maximum size of the drill to be used = 50 mm

Minimum size of the drill to be used = 10mm

Maximum cutting speed = 40m/mt

Minimum cutting speed = 6 m /mt

Number of speeds = 12

Sol:

Max. Speed N _max = 100 * V_max / π D_min = 1000*40 / π * 10= 1280 rpm = n_z

Min. Speed N _min = 100 * V_min/ π D_max = 1000*6 / π * 50= 38 rpm = n₁

The progression ratio,

ɸ = ( Rn)

Rn= 1280/38 =33.68; Z=12

ɸ =33.68 ^{1/12-1 =}1.38

The nearest standard value of ɸ is 1.4

The speeds of shafts are 31.5,45,63,90,125,180,250,355,500,710,100 and 1400 rpm

T_a + T_b = T_c + T_d = T_e+ T_f

N_b/ N_a = T_a/ T_b = 500/1400

N_d / N_c= T_c/ T_d= 710/1400

N_f / N_e = T_e/ T_f  = 1000/1400

Assuming minimum number of teeth on the smaller gear as18( Ta =18)

18/ T_b = 500/1400

T_b = 50

T_c/ T_d= 710/1400

T_a + T_b = 68 = T_c + T_d

T_c  = 23 and T_d = 45

T_e/ T_f= 1000/1400 and  T_e + T_f = 68

T_e = 28 and T_f =40

Considering the transmission between the second and third shafts

T_g + T_h= T_i+ T_j

N_h/ N_g = T_g/ T_h =125/500

N_j / N_i= T_i/ T_j= 355/500

Assuming minimum number of teeth on the smaller gear as 18( Tg =18) 18/ T_h = 125/500

T_h = 72

T_i/ T_j= 355/500

T_i + T_j = 90= T_g + T_h

T_i = 37and  T_j=53

Considering the transmission between the third and output shafts

T_k + T_l= T_m+ T_n

N_l/ N_k = T_k/ T_l=31.5/125

N_n/ N_m= T_m/ T_n= 250/125

Assuming minimum number of teeth on the smaller gear as 18( Tk =18) 18/ T_l = 31.5/125

T_l = 72

T_m/ T_n= 250/125

T_m + T_n = 90= T_k+ T_l

T_m= 60 and  T_n=30