Continuous Time Fourier Transform

Continuous Time Fourier Transform:

The Fourier expansion coefficient x[k] ( in a_k  OWN) of a periodic signal is  

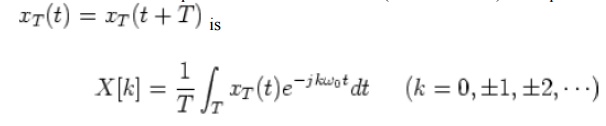
and the Fourier expansion of the signal is:

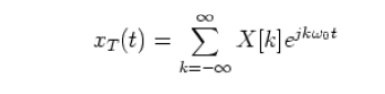
which can also be written as:

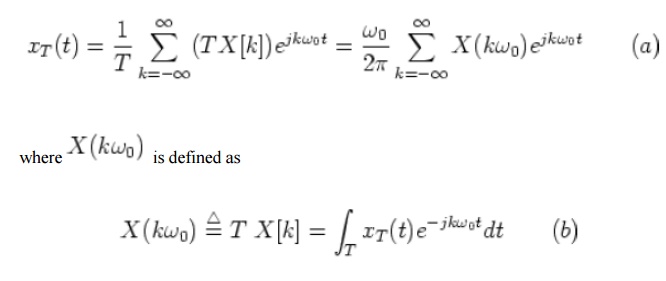
When the period of x_T(t) approaches infinity, T->(infinity) the periodic signal x_T(t) becomes a non-periodic signal and the following will result:

Interval between two neighboring frequency components becomes zero:

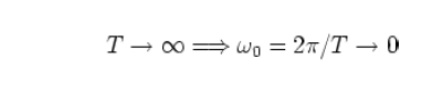
Discrete frequency becomes continuous frequency:

Summation of the Fourier expansion in equation (a) becomes an integral:

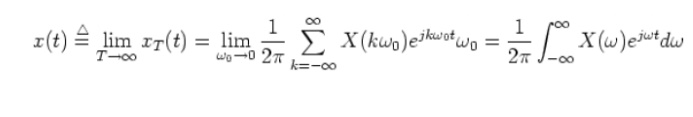
the second equal sign is due to the general fact:

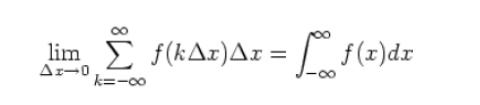
Time integral over in equation (b) becomes over the entire time axis:

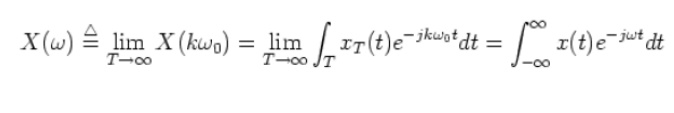
In summary, when the signal is non-periodic x(t)=lim_T->infx_T(t)  the Fourier expansion becomes Fourier transform. The forward transform (analysis) is:

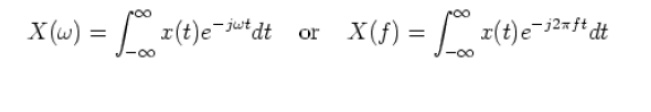
and the inverse transform (synthesis) is:

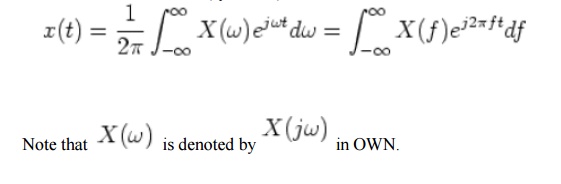
Comparing Fourier coefficient of a periodic signal  x_T(t) withwith Fourier spectrum of a non-periodic signal x(t)

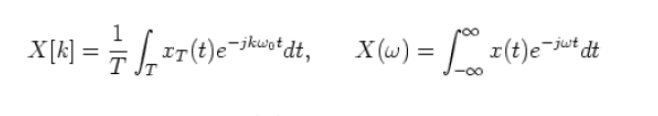
we see that the dimension of X(ω) is different from that of     X[k]:

x(t) distributed along the frequency axis. We can only speak of the energy contained in a particular frequency band ω₁< ω< ω₂