Chapter: Civil : Strength of Materials : Indeterminate Beams
Civil - Strength of Materials - Indeterminate Beams
INDETERMINATE BEAMS
1 STATICALLY INDETERMINATE BEAMS
If the numbers of reaction components are more
than the conditions equations, the structure is
defined as statically indeterminate beams.
E = R -r
E = Degree of
external redundancy
R = Total
number of reaction components
r = Total
number of condition equations available.
A continuous
beam is a typical example of externally indeterminate structure.
2 STATE THE DEGREE OF INDETERMINACY IN
PROPPED CANTILEVER
For a general
loading, the total reaction components (R) are equal to (3+2) =5,
While the total number of condition equations
(r) are equal to 3. The beam is statically
indeterminate, externally to second degree. For vertical loading, the
beam is statically determinate to single
degree.
E = R -r
5
-3 = 2
3 STATE THE DEGREE OF
INDETERMINACY IN A FIXED BEAM
For a general system of loading, a fixed beam
is statically indeterminate to third degree. For vertical loading, a fixed beam is statically
indeterminate to second degree.
E
= R -r
For general system of loading:
R = 3 + 3 and r = 3
E
= 6-3 = 3
For vertical loading:
R
= 2+2 and r = 2
E = 4 -2 = 2
4 STATE
THE DEGREE OF INDETERMINACY IN THE GIVEN BEAM
The beam is statically indeterminate to third
degree of general system of loading.
R
= 3+1+1+1 = 6
E = R-r
6-3 = 3
5 STATE THE DEGREE OF INDETERMINACY IN
THE GIVEN BEAM
The beam is statically determinate. The total
numbers of condition equations are equal to 3+2 = 5. Since, there is a link at B. The two
additional condition equations are at link.
E
=
R-r
=
2+1+2-5
=
5-5
E = 0
6 STATE THE METHODS
AVAILABLE FOR ANALYZING STATICALLY
INDETERMINATE STRUCTURES
i.
Compatibility method
ii.
Equilibrium method
7
WRITE THE EXPRESSION FIXED END MOMENTS AND DEFLECTION FOR A FIXED BEAM CARRYING POINT LOAD AT CENTRE
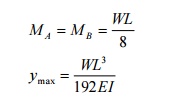
8 WRITE THE EXPRESSION FIXED END MOMENTS
AND DEFLECTION FOR A FIXED BEAM CARRYING
ECCENTRIC POINT LOAD
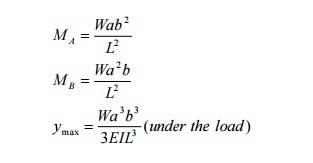
9 WRITE THE EXPRESSION FIXED END MOMENTS
FOR A FIXED DUE TO SINKING OF SUPPORT
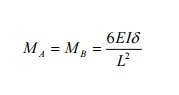
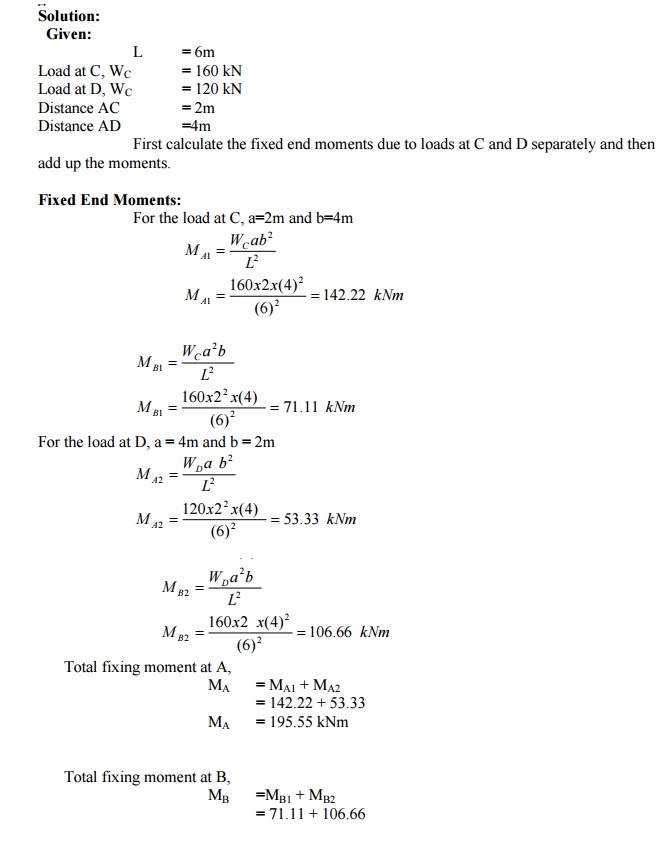
A
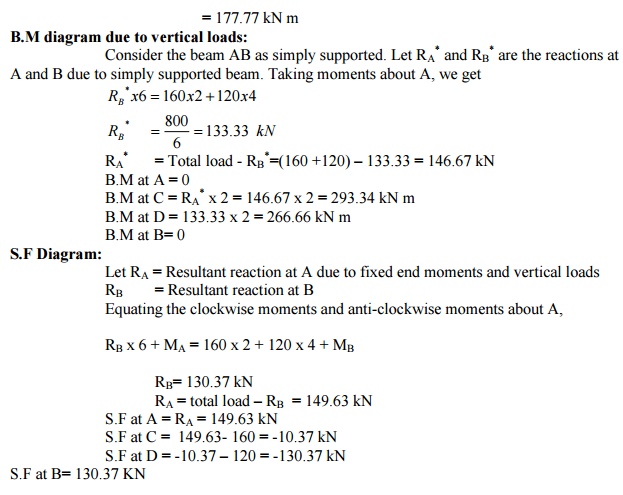
fixed beam AB of length 6m carries point load of 160 kN and 120 kN at a
distance of 2m and 4m from the left end
A. Find the fixed end moments and the reactions at the supports. Draw B.M
and S.F diagrams.
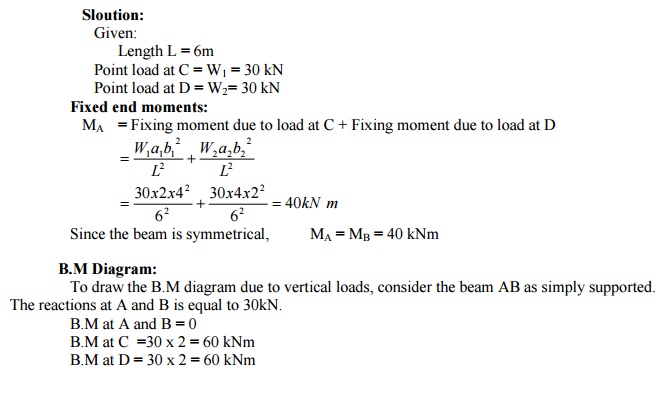
A
fixed beam AB of length 6m carries two point loads of 30 kN each at a distance
of 2m from the both ends. Determine the
fixed end moments and draw the B.M diagram.
Find the fixing moments and support reactions
of a fixed beam AB of length 6m, carrying a uniformly distributed load of 4kN/m over the left half
of the span.

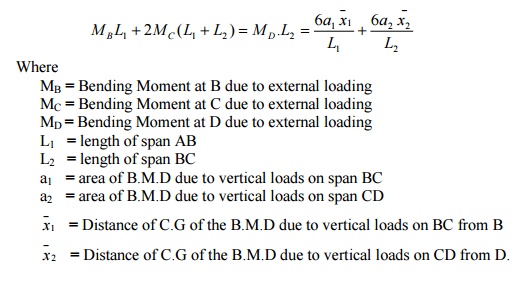
9 STATE THE THEOREM OF
THREE MOMENTS
Theorem of three
moments
It states that 'If BC and CD are only two
co an external loading, then the moments
MB, M C and M D at the supports B, C and D are
given by
What are the fixed end moments for a fixed
beam of length 'L load 'w' at a distance
'a' from left end?
Fixed End Moment:
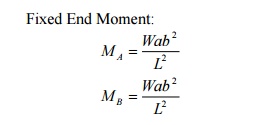
10 EXPLAIN THE EFFECT
OF SETTLEMENT OF SUPPORTS IN A CONTINUOUS BEAM
Due to the settlement of supports in a
continuous beam, the bending stresses will alters appreciably. The maximum bending moment in
case of continuous beam is less when compare to the simply supported beam.
ADVANTAGES OF CONTINUOUS BEAMS OVER SIMPLY SUPPORTED
BEAMS
(i)The maximum bending moment in case of a
continuous beam is much less than in case of a
simply supported beam of same span carrying same loads.
(ii) In case of a continuous beam, the average
B.M is lesser and hence lighter materials of
construction can be used it resist the bending moment.
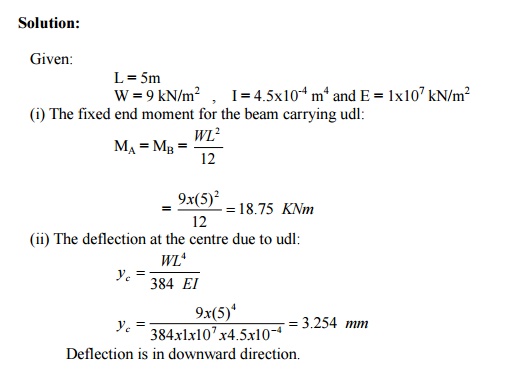
A
fixed beam of length 5m carries a uniformly distributed load of 9 kN/m run over
the entire span.
If I = 4.5x10-4 m4 and E = 1x107 kN/m2,
find the fixing moments at the ends and deflection at the centre.
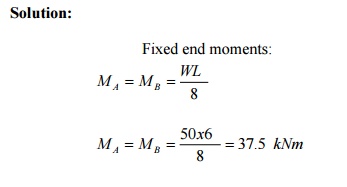
A
fixed beam AB, 6m long is carrying a point load of 40 kN at its center.
The M.O.I of the beam is 78 x 106
mm4 and value of E for beam material is 2.1x105 N/mm2.
Determine (i) Fixed end moments at A and
B.
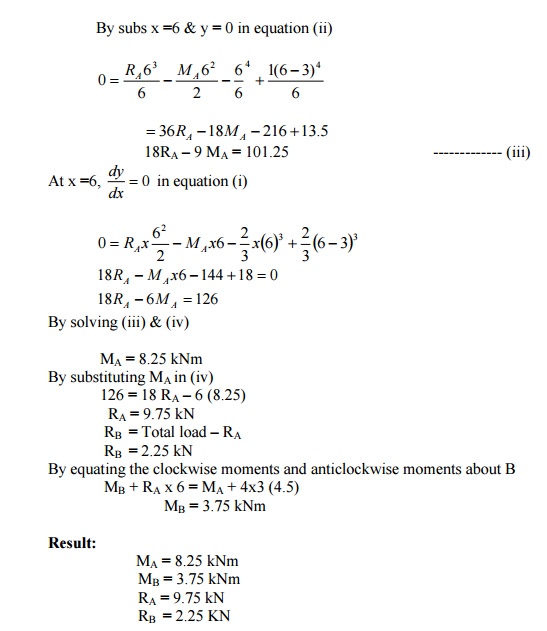
A
fixed beam AB of length 3m is having M.O.I I = 3 x 106 mm4
and value of E for beam material is 2x105
N/mm2. The support B sinks down by 3mm. Determine (i) fixed end moments at A and B.

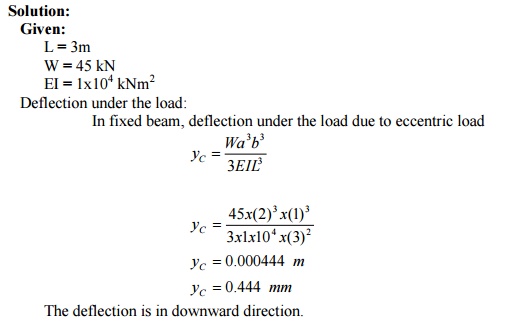
A
fixed beam AB, 3m long is carrying a point load of 45 kN at a distance of 2m
from A. If the
flexural rigidity (i.e) EI of the beam is 1x104kNm2.
Determine (i) Deflection under the Load.
A
fixed beam of 5m span carries a gradually varying load from zero at end A to 10
kN/m at end B. Find the fixing moment
and reaction at the fixed ends.
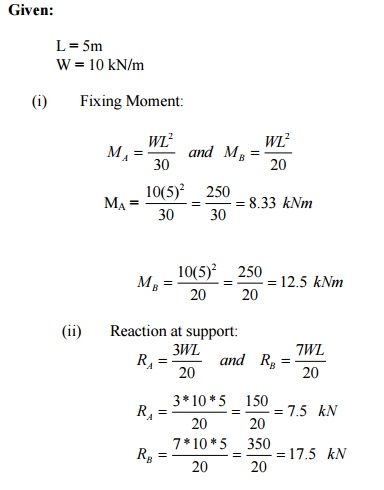
A
continuous beam ABC covers two consecutive span AB and BC of lengths 4m and
6m, carrying uniformly distributed loads
of 6kN/m and 10kN/m respectively. If the ends A and C are simply supported, find the support
moments at A,B and C. draw also B.M.D and S.F.D.


A
continuous beam ABCD of length 15m rests on four supports covering 3 equal
spans and carries a uniformly
distributed load of 1.5 kN/m length .Calculate the moments and reactions at
the supports. Draw The S.F.D and B.M.D.


A
continuous beam ABCD, simply supported at A,B, C and D is loaded as shown in
fig.
Find the moments over the beam and draw B.M.D
and S.F.D.
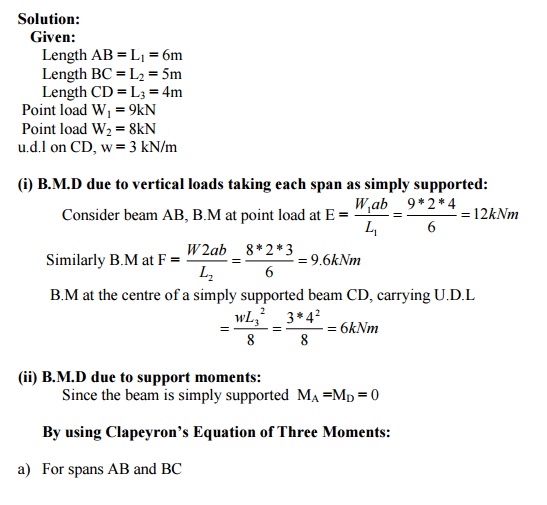
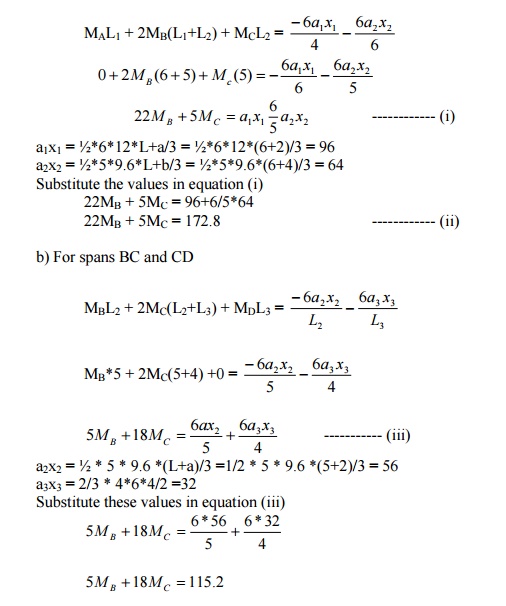
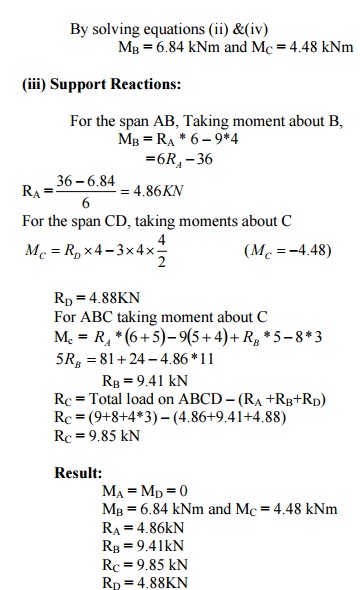
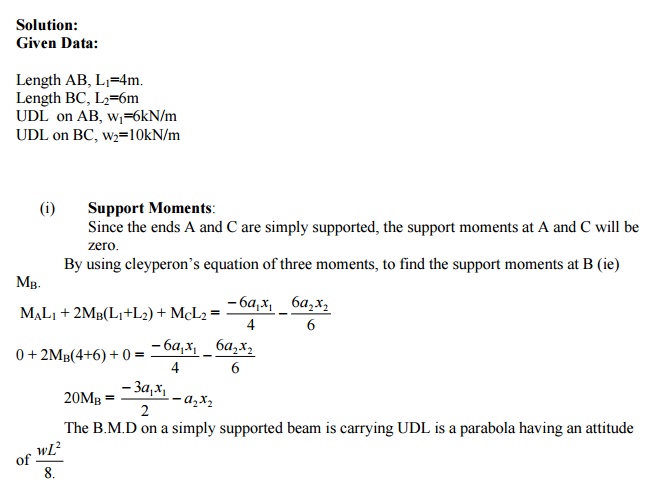
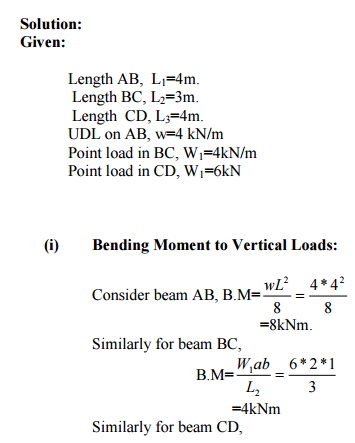
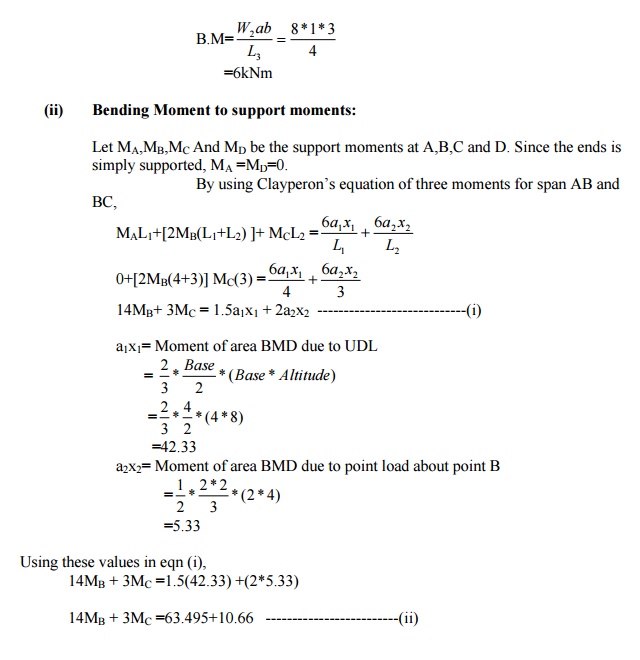
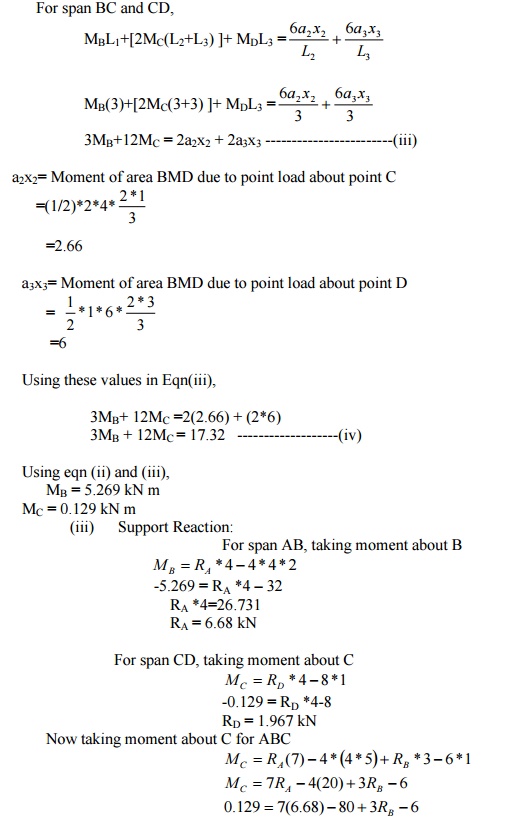
(i)
B.M.D due to vertical loads taking
each span as simply supported:
(ii) B.M.D due to support moments:
Since the beam is simply supported MA
=MD = 0
a) For
spans AB and BC
Using the theorem of three moments draw the
shear force and bending moment diagrams
for the following continuous beam.
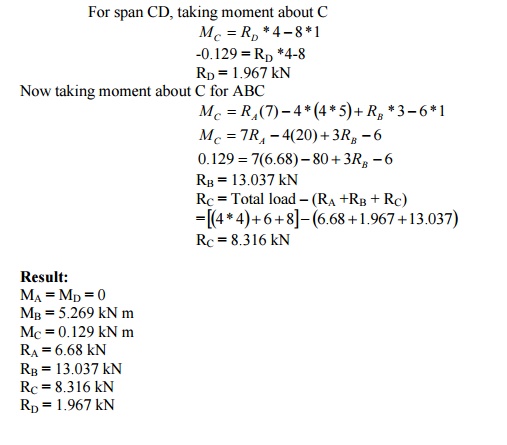
A
beam AB of 4m span is simply supported at the ends and is loaded as shown in
fig.
Determine (i) Deflection at C (ii) Maximum
deflection (iii) Slope at the end A.
E= 200 x 106 kN/m2 and I
= 20 x 10-6 m4

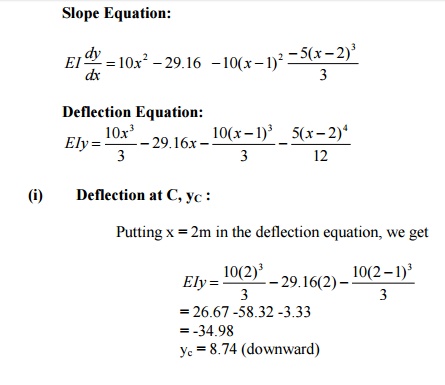

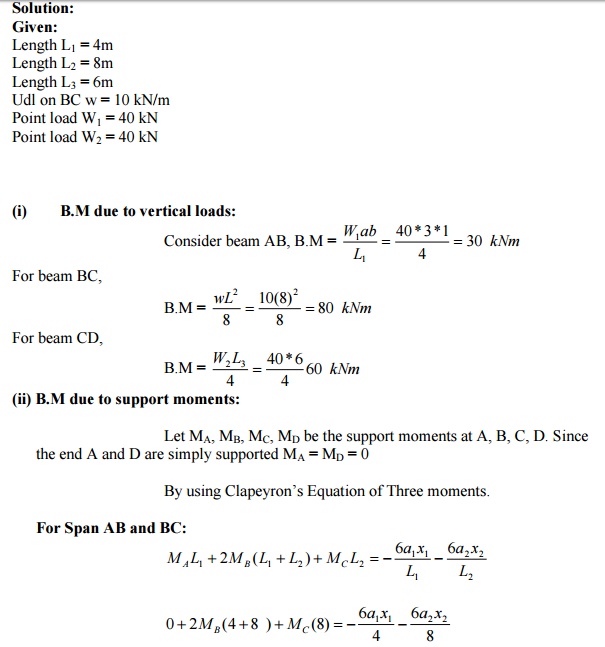
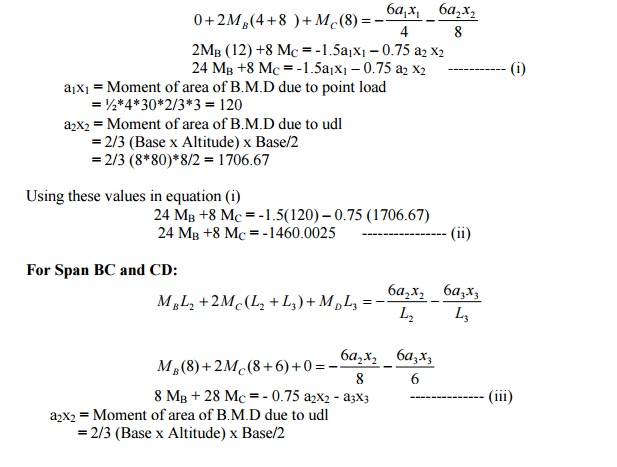
9. A continuous beam is shown in fig. Draw the
BMD indicating salient points.
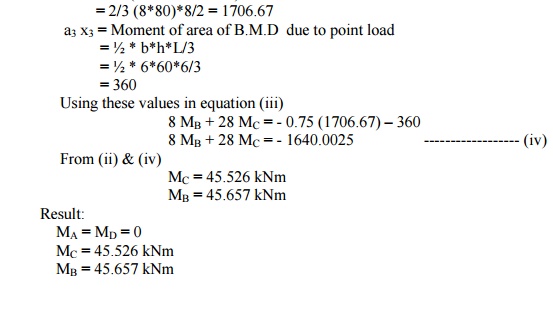
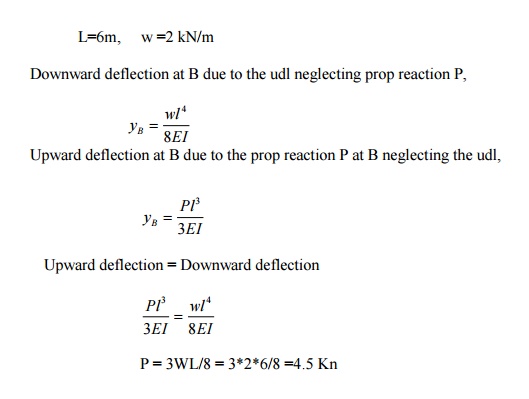
10. A cantilever beam AB of span 6m is
fixed at A and propped at B. The beam carries a udl of 2kN/m over its whole length. Find the
reaction at propped end.
Important
Questions and Answers
1. Explain with examples the statically
indeterminate structures.
If the forces on
the members of a structure cannot be determined by using conditions of
equilibrium (?Fx =0, ?Fy = 0, ?M = 0 ), it is
called statically indeterminate structures.
Example: Fixed beam, continuous beam.
2. Differentiate the statically determinate
structures and statically indeterminate structures?

3. Define: Continuous beam.
A Continuous beam
is one, which is supported on more than two supports. For usual loading on the
beam hogging ( - ive ) moments causing convexity upwards at the supports and
sagging ( + ve ) moments causing concavity upwards occur at mid span.
4.
What are the advantages of
Continuous beam over simply supported beam?
1.
The maximum bending moment in case
of continuous beam is much less than in case
of simply supported beam of same span carrying
same loads.
2. In case of
continuous beam, the average bending moment is lesser and hence lighter
materials of construction can be used to resist the bending moment.
5.Write down the general
form of Clapeyron's three moment equations for the continuous beam.
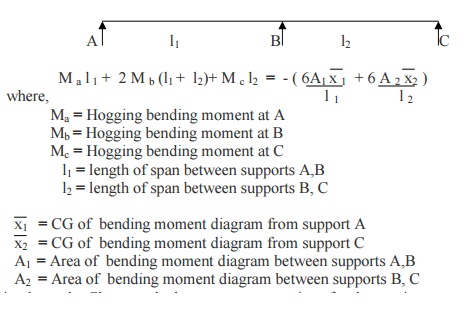
6. Write down the Clapeyron's three moment equations for the continuous beam with sinking
at the supports.
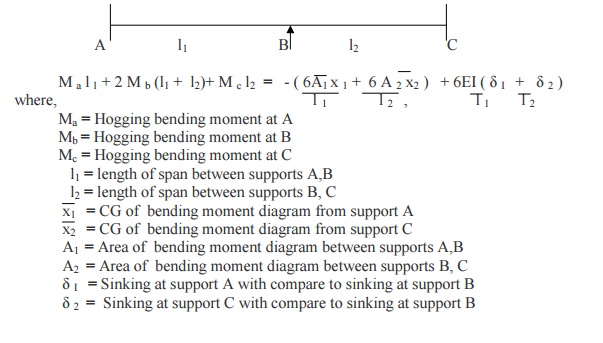
7.Write
down the Clapeyron's three moment equations for the fixed beam
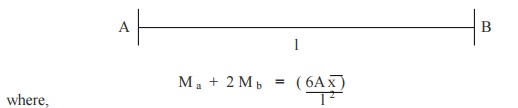
Ma =
Hogging bending moment at A Mb = Hogging bending moment at B
l = length of span between supports A,B
x = CG
of bending moment diagram from support A
![]() A = Area of bending moment diagram between supports A,B
A = Area of bending moment diagram between supports A,B
8. Write down the
Clapeyron's three moment equations for the continuous beam carrying UDL on both the spans.

9. Give the
values of ( 6A 1 x 1 / l 1 ),
( 6A 2 x 2 / l 2
) values for different type of loading.
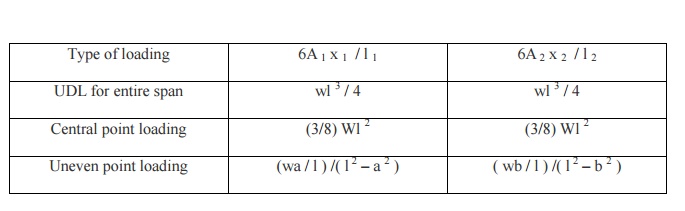
10.
Give the procedure for analyzing the
continuous beams with fixed ends using three moment equations?
The three moment
equations, for the fixed end of the beam, can be modified by imagining a span
of length l 0 and moment of inertia, beyond the support the and
applying the theorem of three moments as usual.
11.
Define Flexural Rigidity of Beams.
The product of young's modulus (E) and
moment of inertia (I) is called Flexural
Rigidity (EI) of Beams. The unit is N mm 2.
12. What is a fixed beam?
A beam whose both
ends are fixed is known as a fixed beam. Fixed beam is also called as built-in
or encaster beam. Incase of fixed beam both its ends are rigidly fixed and the
slope and deflection at the fixed ends are zero.
13.
What are the advantages of fixed
beams?
(i)
For the same loading, the maximum
deflection of a fixed beam is less than that of a simply supported beam.
(ii)
For the same loading, the fixed beam
is subjected to lesser maximum bending moment.
(iii)
The slope at both ends of a fixed
beam is zero.
(iv)
The beam is more stable and
stronger.
14.
What are the disadvantages of a
fixed beam?
(i)
Large stresses are set up by
temperature changes.
(ii)
Special care has to be taken in
aligning supports accurately at the same lavel.
(iii)
Large stresses are set if a little
sinking of one support takes place.
(iv)
Frequent fluctuations in
loadingrender the degree of fixity at the ends very uncertain.
15.Write the formula for deflection
of a fixed beam with point load at centre.
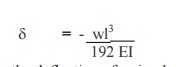
This defection is ¼ times the
deflection of a simply supported beam.
15.
Write the formula for deflection of
a fixed beam with uniformly distributed load..
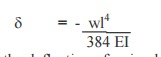
This defection is 5 times the deflection of a
simply supported beam.
17. Write the formula for deflection of a fixed
beam with eccentric point load..
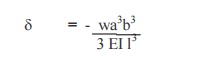
18.
What are the fixed end moments
for a fixed beam with the given loading conditions.
![]()
![]()
![]()
![]()
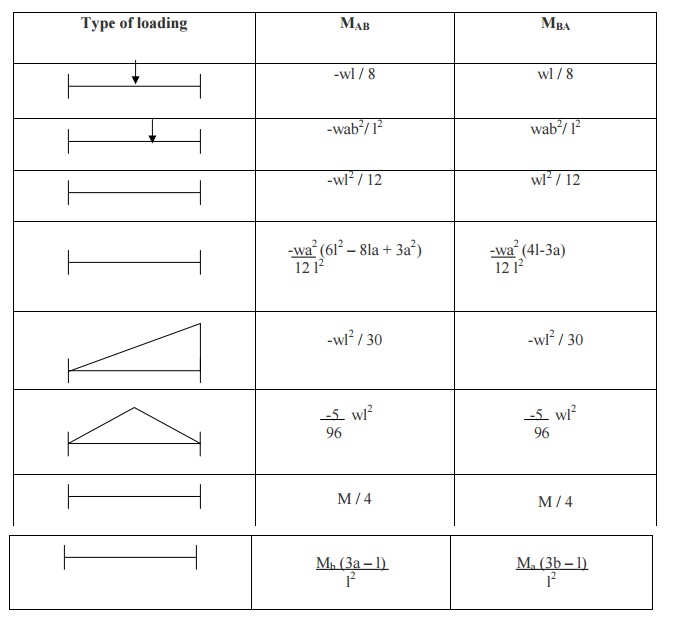
Related Topics