Age Distribution in Populations of Growing Cell

Age Distribution in Populations of Growing Cell

The cells in a population of freely growing cells are not all alike. A newly divided cell grows, doubles in volume, and divides into two daughter cells.

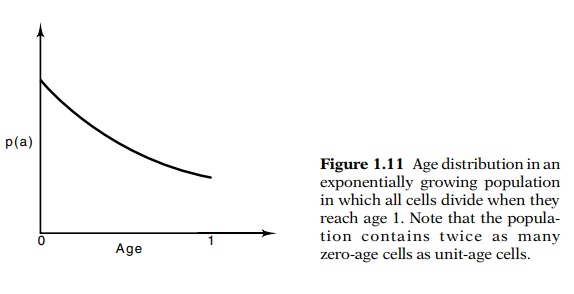
 Consequently, freely growing populations contain twice as many cells that have just divided as cells about to divide. The distribution of cell ages present in growing populations is an important consideration in a number of molecular biology experiments. Therefore we will derive the distribution of ages present in such populations.

Consider an idealized case where cells grow until they reach the age of 1, at which time they divide. In reality most cells do not divide at exactly this age, but the ages at which cell division occurs cluster around a peak. To derive the age distribution, let N(a,t)da be the number of cells with age between aand a + da at time t. For convenience, we omit writing the da. Since the number of cells of age a at time t must be the same as the number of zero-age cells at time t-a, N(a,t) = N(0,t -a). Since the numbers of cells at any age are growing exponentially, N(0,t) = N(0,0)e^µt, and N(a,t) = N(0,t-a) = N(0,t)e^-µa. Therefore the probability that a cell is of age a, p(a), is p(0)e^-µa= p(0)2^-a/Td (Fig. 1.11).