Vector Calculus

VECTOR CALCULUS

1        Gradient-Directional Derivative

2.       Divergence And Curl –Irrotational And Solenoidal Vector Fields Divergence 

3        Vector Integration

4        Green’s   Theorem   In   A   Plane;(Excluding proof)

5        Gauss Divergence Theorem:(Excluding proof)

6        Stoke’s   Theorem(Excluding   proof)

VECTOR CALCULUS

1Gradient-Directional Derivative

1.1. Gradient

1(a) The Vector Differential Operator

1(b) The Gradient (Or Slope Of A Scalar Point Function)

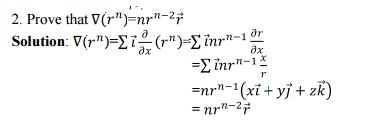
1.2.  Directional Derivative

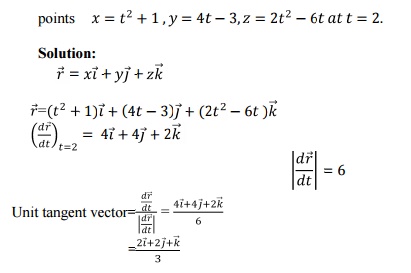
1.3. Unit Tangent Vector

1.Find  a unit tangent vector to the following surfaces at the specified

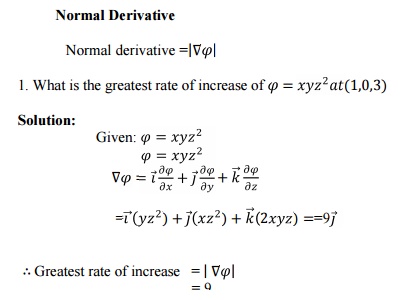
1.4 Normal Derivative

1.5 Unit Normal Vector

1.6 Angle Between The Suraces

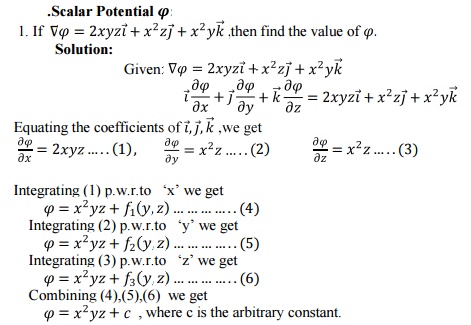
1.7 .Scalar Potential 

1. 8 The Vector Equation of the Tangent Plane And Normal Line to the Surface

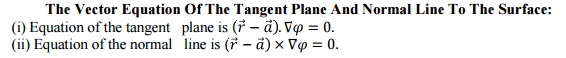
Tutorial Problems:

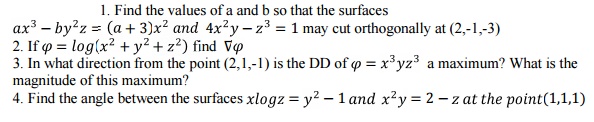
2 Divergence And Curl –Irotational And Solenoidal Vector Fields:

2.1 Divergence and curl

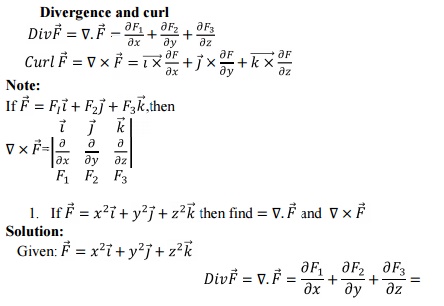
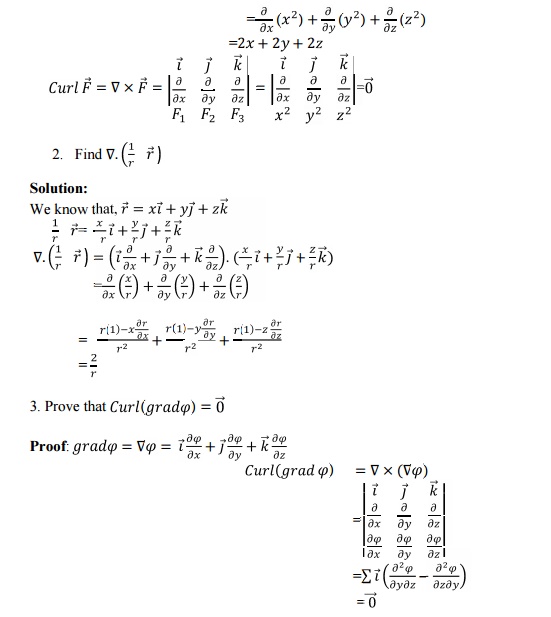
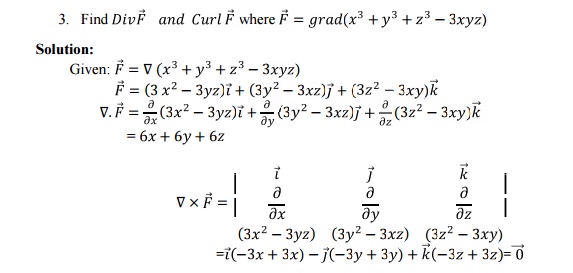
2.2   SOLENOIDAL VECTOR,IRROTATIONAL VECTOR:

Solenoidal vector formula:

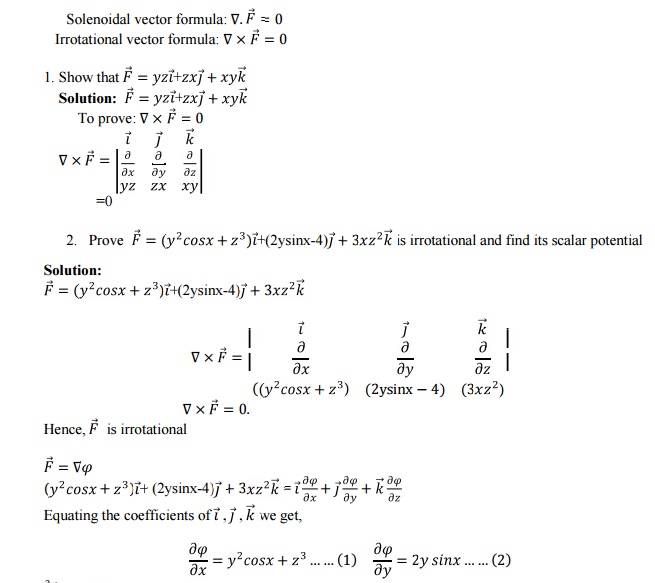
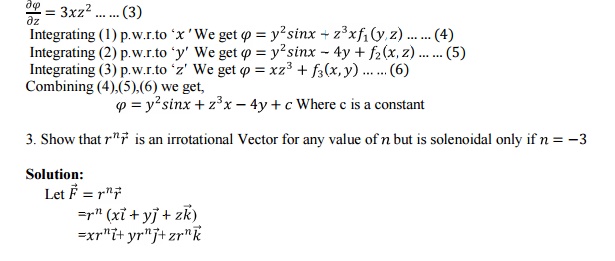
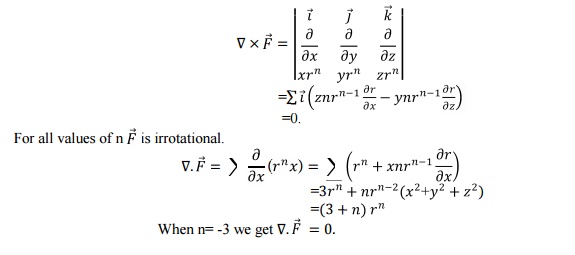
Laplace Operator:

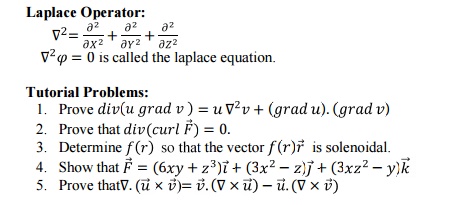
3 Vector Integration

Conservative Vector Field:

3.1. Line Integral:

3.2. Surface Integral:

Definition: Consider a surface S .Let n denote the unit outward normal to the surface S. Let R be the projection of the surface x on xy plane. Let Vec f be a vector function defined in some region  containing the surface S, then the surface integral of Vector f is defined to be 

3.3. Volume Integral:

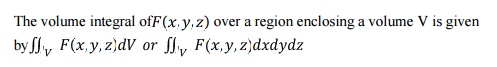
3.4 Tutorial Problems:

4 .Green’s Theorem In A Plane:

Statement:

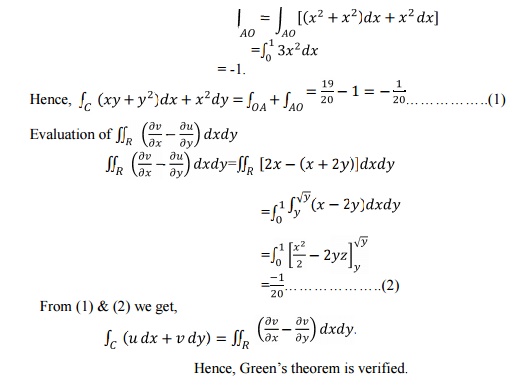
Tutorial Problems:

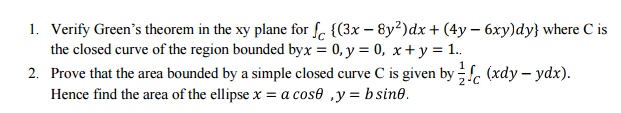
5 Gauss Divergence Theorem:

Statement:

          The surface integral of the normal component of a vector function F over a closed surface  S enclosing volume V is equal to the volume integral of the divergence of F taken throughout the

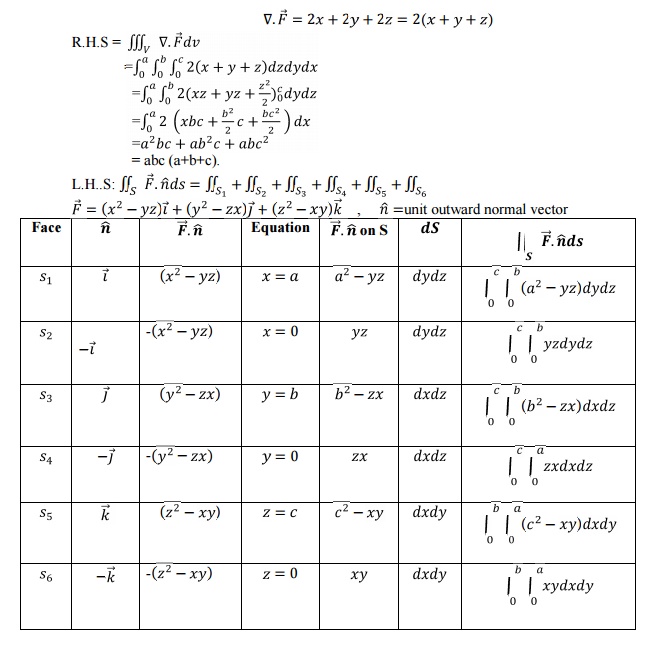
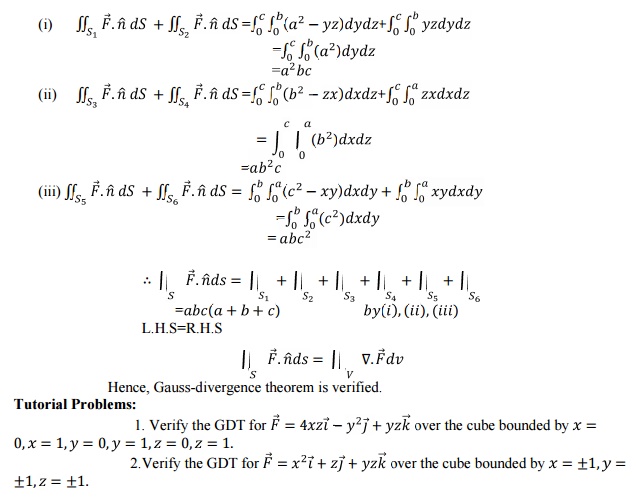
6. Stoke’s Theorem

Statement:

The surface integral of the normal component of the curl of a vector function F over an open surface S is equal to the line integral of the tangential component of F around the closed curve C bounding S.      

Hence, Stoke’s  theorem is verified.

6.1 Tutorial Problems: