Chapter: Civil : Structural Analysis : Archs
Solved Problems: Archs- Structural Analysis
1.A three hinged parabolic arch hinged at the crown and springing has a horizontal span of 12m and a central rise of 2.5m. it carries a udl of 30 kN/m run over the left hand half of the span. Calculate the resultant at the end hinges.
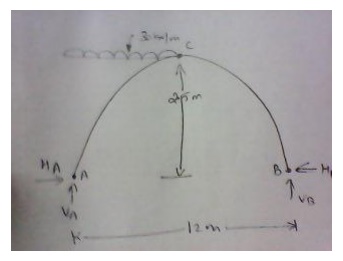
Let us take a section X of an arch. Let ?be the inclination of the tangent at X. if H is the horizontal thrust and V the net vertical shear at X, from theb free body of the RHS of the arch, it is clear that V and H will have normal and radial components given by,
N = H cos ?+ V sin ? R = V cos?-H sin ?
The normal thrust and radial shear in an arch rib.
Parabolic arches are preferable to carry distributed loads. Because, both, the shape of the arch and the shape of the bending moment diagram are parabolic. Hence the intercept between the theoretical arch and actual arch is zero everywhere. Hence, the bending moment at every section of the arch will be zero. The arch will be under pure compression that will be economical.
Difference between the basic action of an arch and a suspension cable
An arch is essentially a compression member, which can also take bending moments and shears. Bending moment and shears will be absent if the arch is parabolic and the loading uniformly distributed.
A cable can take only tension. A suspension bridge will therefore have a cable and a stiffening girder. The girder will take the bending moment and shears in the bridge and the cable, only tension.
Because of the thrust in cables and arches, the bending moments are considerably reduced.
If the load on the girder in uniform. The bridge will have only cable tension and no bending moment on the girder.
Under what conditions will the bending moment in an arch be zero throughout
The bending moment in an arch throughout the span will be zero, if
(i) The arch is parabolic and
(ii) The arch carries udl throughout the span
2.A three-hinged semicircular arch carries a point load of 100 kN at the crown. The radius of the arch is 4m. Find the horizontal reactions at the supports.
VA = VB = 50 kN
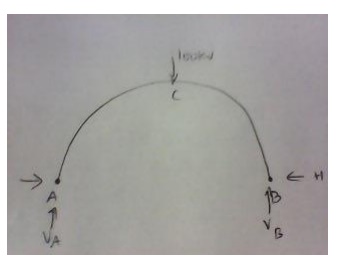
Equating the moment about C to Zero, VA * 4 -H*4 = 0
H = VA
Horizontal reaction, H = 50 kN
3.A three-hinged semicircular arch of radius 10m carries a udl of 2 kN/m over the span. Determine the horizontal and vertical reactions at the supports.
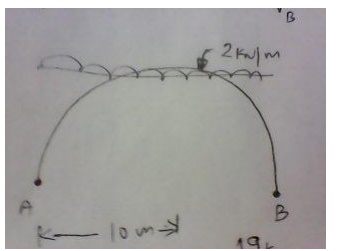
Determine H, VA and VB in the semicircular arch shown in fig
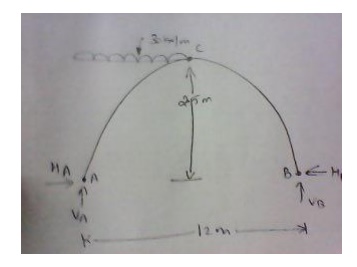
Equating moments about A to Zero,
VB * 12 -12 * 9 = 0;
VB = 9 kN and VA = 3 kN
Equating moments to the left of C to zero,
H = VA = 3 kN; H= 3 kN
Distinguish between two hinged and three hinged arches.
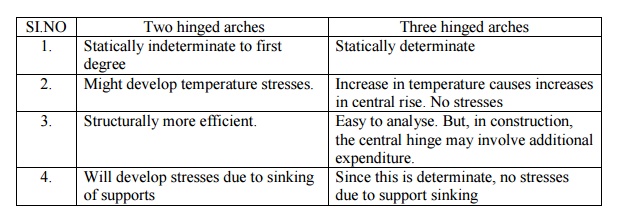
Two hinged arches
Statically indeterminate to first degree
Might develop temperature stresses.
Structurally more efficient.
Will develop stresses due to sinking of supports
Three hinged arches
Statically determinate
Increase in temperature causes increases in central rise. No stresses
Easy to analyse. But, in construction, the central hinge may involve additional expenditure.
Since this is determinate, no stresses due to support sinking
Rib -shorting in the case of arches.
In a 2-hinged arch, the normal thrust, which is a compressive force along the axis of the arch, will shorten then rib of the arch. This is turn will release part of the horizontal thrust.
Normally, this effect is not considered in the analysis (in the case of two hinged arches). Depending upon the important of the work we can either take into account or omit the effect of rib shortening. This will be done by considering (or omitting) strain energy due to axial compression along with the strain energy due to bending in evaluating H.
Effect of yielding of support in the case of an arch.
Yielding of supports has no effect in the case of a 3 hinged arch which is determinate. These displacements must be taken into account when we analyse 2 hinged or fixed arches as under

Here U is the strain energy of the arch ?H and ?VA are the displacements due to yielding of supports.
5.A three-hinged parabolic arch has a horizontal span of 36m with a central rise of 6m. A point load of 40 kN moves across the span from the left to the right. What is the absolute maximum positive bending moment that wills occur in the arch
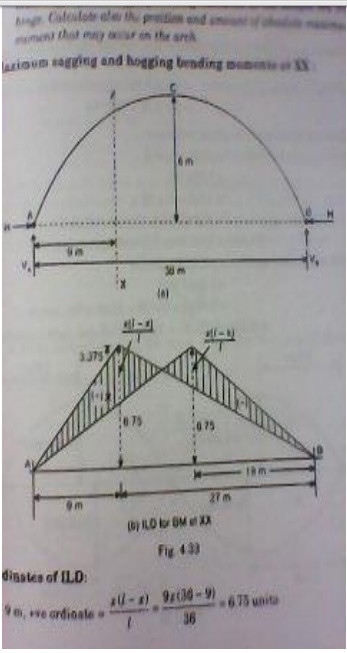
For a single concentrated load moving from one end to the other, Absolute maximum positive bending moment
= 0.096wl = 0.096*40 * 36=138.24 kNm This occurs at 0.211 l = 0.211 * 36 = 7.596 m from the ends.
Absolute maximum positive bending moment = 138.24 kNm at 7.596 m from the ends.
6.A 3 hinged arch of span 40m and rise 8m carries concentrated loads of 200 kN and 150 kN at a distance of 8m and 16m from the left end and an udl of 50 kN/m on the right half of the span. Find the horizontal thrust.
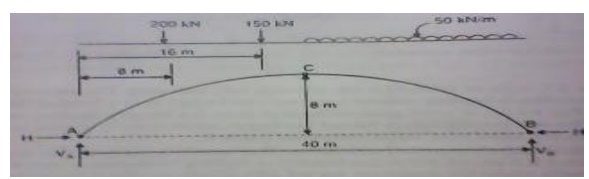
Solution:
(a) Vertical reactions VA and VB :
Taking moments about A,
200(8) + 150(16) + 50 * 20 * (20 + 20/2) -VB (40) = 0
1600 + 2400 + 30000 -40 VB = 0
VB= = 850 kN
VA = Total load -VB = 200 + 150 + 50 * 20 -850 = 500 kN
(b) Horizontal thrust (H)
Taking moments about C,
-H x 8 + VA (20) -200 (20 -8) -150 (20 -16) = 0 -8H + 500 * 20 -200 (12) -150 (4) = 0
H = 875 kN
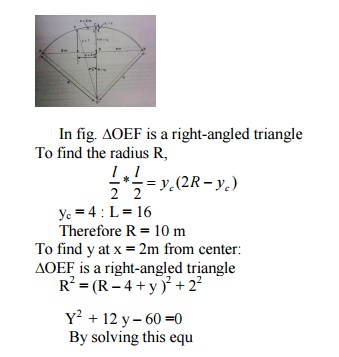
7.A parabolic 3-hinged arch carries a udl of 30kN/m on the left half of the span. It has a span of 16m and central rise of 3m. Determine the resultant reaction at supports. Find the bending moment, normal thrust and radial shear at xx, and 2m from left support.
Solution:
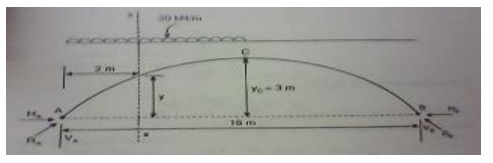
(1) Reaction at A nd B;
(i) Vertical components of reactions;
Taking moments about A,
-VB (16) + 30 x 82 /2 = 0
- VB (16) + 30 * 32 = 0 VB = 60 kN
VA = Total load -VB = 30 * 8 -60 kN VA = 180 kN
(ii) Horizontal components of reactions at A and
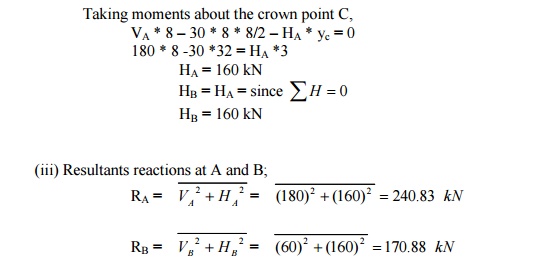
(2) Bending moment at x = 2m from A:
Bending moment = VA (2) -30 * 2 *1 -HA(y) ---- (1)
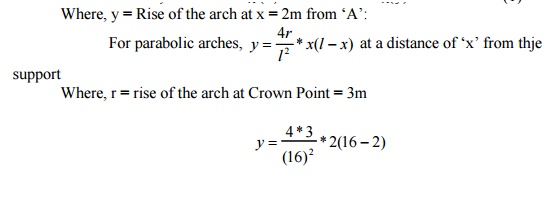
Substitute in (1)y = 1.3125 m at x = 2m fromA''.
Bending moment at x = 2m from A = 180 (2) -30 * 2 * 1 -160 * 1.3125
Bending moment at xx = 90 kNm
(3) Radial shear force at x = 2m from A
Shear force, RX = Vx cos ?-H sin ? Where, V = Net vertical shear force at x = 2m from A
= VA - w (2) = 180 -30 * 2 V = 120 kN
H = Horizontal shear force = 160 kN
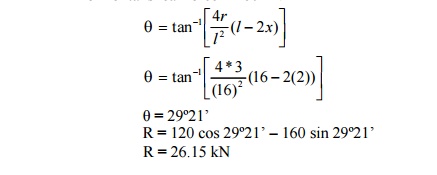
(4) Normal thrust at x = 2m from A:
Normal thrust PN = Vx sin?+ H cos ?= 120 sin 29º21'+160cos 29º21' PN = 198.28 kN.
8.A parabolic 3-hinged arch carries loads as shown in fig. Determine the resultant reactions at supports. Find the bending moment, normal thrust and radial shear at D, 5m from A. What is the maximum bending moment
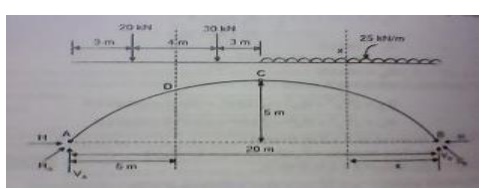
Solution:
(1)Reaction at supports: (RA and RB)
(i) Vertical components of RA and RB : (VA and VB) Taking moments about A,
20 * 3 + 30 (7) + 25 * 10 * (10 +10/2) -VB *(20) = 0 VB = 201 kN
VA = Total load -VB = 20 + 30 + 25 * 10 -201 VA = 99 kN
(ii) Horizontal thrust (H):
Taking moments about the crown point C, considering the right
side of 'C',
-VB (20/2) + H (5) + 25 * 10 *5 = 0 -201 * (20/2) + 5 H + 1250 + 0
H = 125 kN (iii) Resultant reactions RA and RB ;
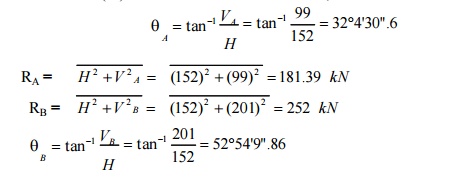
2. Bending moment, normal thrust and radial shear force (at D):

(iii) Normal thrust
P = V sin ?+ H cos ?
V = Net beam shear force = VA -20 V = 99 -20 = 79 kN
Substitute in (iii) P = 79 sin ?+ 152 cos ?= 179.28 kN (iv) Radial shear force
F = V cos ?-H sin ?
F = 79 cos ?-152 sin ?= 2.683 kN
3. Maximum Bending Moment in CB:
Considering a section xx at a distance of 'x' fromm B'' BMxx = 254KNM
9.A 3-hinged arch is circular, 25 m in span with a central rise of 5m. It is loaded with a concentrated load of 10 kN at 7.5m from the left hand hinge. Find the
(a) Horizontal thrust
(b) Reaction at each end hinge
(c) Bending moment under the load
Solution:
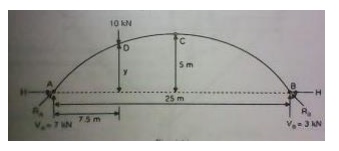
ertical reactions VA and VB:
Taking moments about A, 10(7.5) -VB (25) = 0
VB = 3 kN
VA = Total load -VB = 10 -3 = 7 kN
1.Horizontal thrust (H):
Taking moments about C

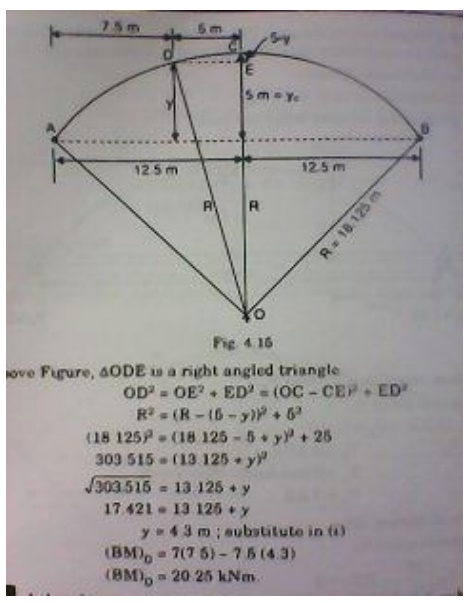
10.A three hinged circular arch of span 16m and rise 4m is subjected to two point loads of 100 kN and 80 kN at the left and right quarter span points respectively. Find the reactions at supports. Find also the bending moment, radial shear and normal thrust at 6m from left support.
Solution:

(a) Reaction at A and B:
(i) Vertical components of reactions at A and B:
Taking moment about A,
100 (4) + 80(12) -VB(16) = 0 VB = 85 kN.
VA = Total load - VB = (100+80)-85 VA = 95 kN.
b. Horizontal components of reactions at A and B; Taking moments about the crone points C
VA(8) -H(YC) - 100(4) = 0 95 (8) -H (yC ) -100 (4) = 0
H = 90 kN
(iii)Resultant reactions at A and B:
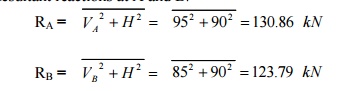
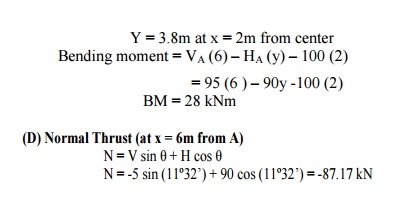
b) Bending moment at 6m from the left support:
(c) Radial shear force 'F' :
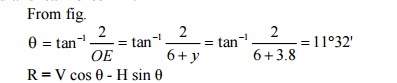
R = V cos ?- H sin ?
V = net shear force at x = 6m from A
= VA -100 = 95 -100 = -5 kN H = 90 kN
R = -5 cos (11º32')-90 sin (11º32')= - 22.895 R = -22.89 kN
11.A symmetrical three hinged parabolic arch of span 40m and rise 8m carries an udl of 30 kN/m over left of the span. The hinges are provided at these supports and at the center of the arch. Calculate the reactions at the supports. Also calculate the bending moment, radial shear, normal thrust at distance of 10 m from the left support.
Solution:
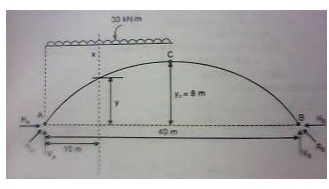
(1) Reactions at the supports:
(i)Vertical components;
Taking moments about A,
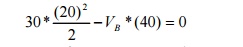
Vertical component of RB, VB = 150 kN
VA = Total load -VB = 30 * 20 -150 = 450 kN
(iii)Horizontal components
Taking moments about the crown, 'C',
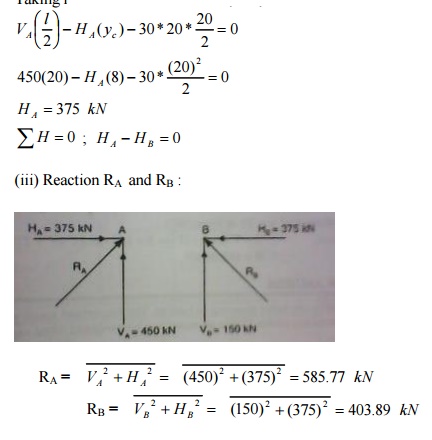
(22) Bending moment at 10 m from A:

(3)Radial shear force at x = 10m:
R = Radial shear force = V cos ?-H sin ? Where, V = Net vertical shear force at x = 10m from A
H = Horizontal thrust.
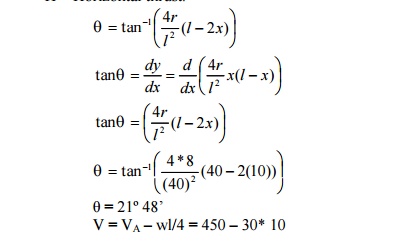
Radial shear force, R = V cos ?-H sin ?
R = 150 cos 21º-37548'sin 21º48'
R = 0
(4) Normal thrust at x = 10m from 'A':
Normal thrust, N = V sin ?+ H cos ?= 150 sin 21º48'375 cos+ 21?48' N = 403.89 kN
12.A parabolic 3-hinged arch of span 'l'issubjected to an u.d.l of w/m run over the entire span. Find the horizontal thrust and bending moment at any section XX.
Solution:
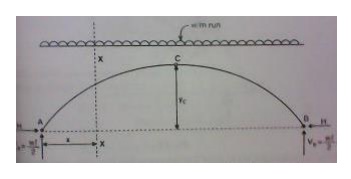
(a) Reactions (Vertical) at the supports:
As the loading is symmetrical, vertical reactions at A and B are equal VA = VB = Total load/2 = wl/2
(b) Horizontal thrust:
Taking moments about the crown point C,
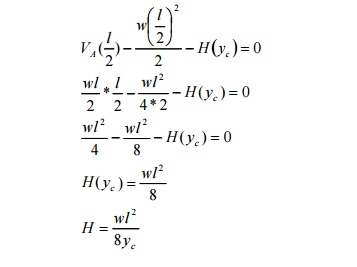
(c) Bending moment at xx;
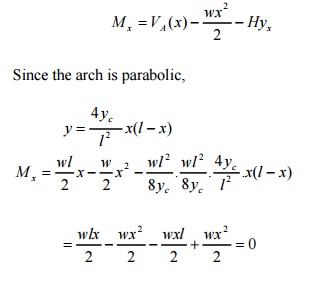
Related Topics