Chapter: Modern Pharmacology with Clinical Applications: Mechanisms of Drug Action
Dose–Response Relationship
DOSE–RESPONSE
RELATIONSHIP
To understand drug–receptor
interactions, it is neces-sary to quantify the relationship between the drug
and the biological effect it produces. Since the degree of ef-fect produced by
a drug is generally a function of the amount administered, we can express this
relationship in terms of a dose–response
curve. Because we cannot always quantify the concentration of drug in the
bio-phase in the intact individual, it is customary to corre-late effect with
dose administered.
In general, biological
responses to drugs are graded; that
is, the response continuously increases (up to the maximal responding capacity
of the given responding system) as the administered dose continuously
in-creases. Expressed in receptor theory terminology, this means that when a graded dose–response relationship
exists, the response to the drug is
directly related to the number of receptors with which the drug effectively
in-teracts. This is one of the tenets of pharmacology.
The principles derived from
dose–response curves are the same in animals and humans. However, obtain-ing
the data for complete dose–response curves in hu-mans is generally difficult or
dangerous. We shall there-fore use animal data to illustrate these principles.
Quantal Relationships
In addition to the
responsiveness of a given patient, one may be interested in the relationship
between dose and some specified quantum of response among all individ-uals taking that drug. Such information is obtained by
evaluating data obtained from a quantal
dose–response curve.
Anticonvulsants can be
suitably studied by use of quantal dose–response curves. For example, to assess
the potential of new anticonvulsants to control epileptic seizures in humans,
these drugs are initially tested for their ability to protect animals against
experimentally induced seizures. In the presence of a given dose of the drug,
the animal either has the seizure or does not; that is, it either is or is not
protected. Thus, in the design of this experiment, the effect of the drug
(protection) is all or none. This type of response, in contrast to a graded re-sponse, must
be described in a noncontinuous manner.
The construction of a quantal dose–response curve requires that data be obtained from many individuals. Although any given patient (or animal) either will or will not respond to a given dose, a comparison of indi-viduals within a population shows that members of that population are not identical in their ability to respond to a particular dose. This variability can be expressed as a type of dose–response curve, sometimes termed a quantal dose–response curve, in which the dose (plotted on the horizontal axis) is evaluated against the percent-age of animals in the experimental population that is protected by each dose (vertical axis). Such a dose– response curve for the anticonvulsant phenobarbital is illustrated in Figure 2.2A. Five groups of 10 rats per group were used. The animals in any one group received a particular dose of phenobarbital of 2, 3, 5, 7, or 10 mg/kg body weight. The percentage of animals in each group protected against convulsions was plotted against the dose of phenobarbital. As Figure 2.2A shows, the lowest dose protected none of the 10 rats to which it was given, whereas 10mg/kg protected 10 of 10. With the intermediate doses, some rats were protected and some were not; this indicates that the rats differ in their sensi-tivity to phenobarbital.
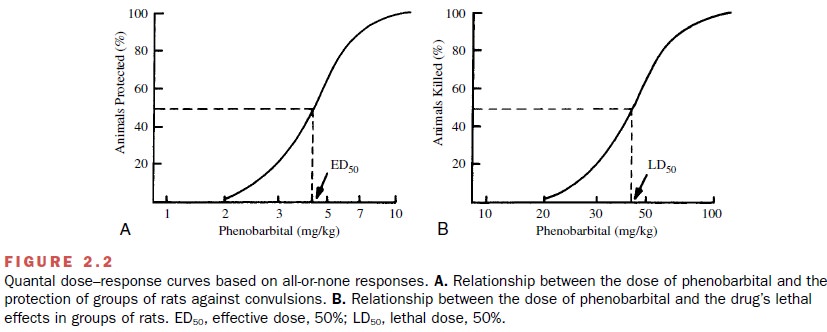
The quantal dose–response
curve is actually a cu-mulative plot of
the normal frequency distribution curve.
The frequency distribution curve, in this case re-lating the minimum protective
dose to the frequency with which it occurs in the population, generally is bell
shaped. If one graphs the cumulative frequency versus dose, one obtains the
sigmoid-shaped curve of Figure 2.2A. The
sigmoid shape is a characteristic of most dose–response curves when the dose is plotted on a geo-metric, or log,
scale.
Therapeutic Index
Effective Dose
The quantal dose–response
curve represents esti-mates of the frequency
with which each dose elicits the desired response in the population. In
addition to this information, it also would be useful to have some way to
express the average sensitivity of the entire population to phenobarbital. This
is done through the calculation of an ED50 (effective dose, 50%;
i.e., the dose that would protect 50% of the animals). This value can be
obtained from the dose–response curve in Figure 2.2A, as shown by the broken lines. The ED50 for
phenobarbital in this population is approximately 4mg/kg.
Lethal Dose
Another important
characteristic of a drug’s activity is its toxic
effect. Obviously, the ultimate toxic effect is death. A curve similar to
that already discussed can be constructed by plotting percent of animals killed
by phenobarbital against dose (Fig. 2.2B).
From this curve, one can calculate the LD50 (lethal dose, 50%).
Since the degree of safety associated with drug administration de-pends on an
adequate separation between doses produc-ing a therapeutic effect (e.g., ED50) and doses producing toxic effects (e.g.,
LD50), one can use a comparison of these two doses to estimate drug
safety. Thus, one esti-mate of a drug’s margin of safety is the ratio LD50/ED50;
this is the therapeutic index. The
therapeutic index for phenobarbital used as an anticonvulsant is approxi-mately
40/4, or 10.
As a general rule, a drug
should have a high thera-peutic index; however, some important therapeutic agents
have low indices. For example, although the ther-apeutic index of the cardiac
glycosides is only about 2 for the treatment and control of cardiac failure,
these drugs are important for many cases of cardiac failure. Therefore, in
spite of a low margin of safety, they are of-ten used for this condition. The
identification of a low margin of safety, however, dictates particular caution
in its use; the appropriate dose for each individual must be determined
separately.
It has been suggested that a
more realistic estimate of drug safety would include a comparison of the lowest
dose that produces toxicity (e.g., LD1) and the highest dose that
produces a maximal therapeutic response (e.g., ED99). A ratio less
than unity would indicate that a dose effective in 99% of the population will
be lethal in more than 1% of the individuals taking that dose. Figure 2.2
indicates that Phenobarbital’s ratio LD1/ED99 is
approximately 2.
Protective Index
The margin of safety is only one of several criteria to be used in determining
a drug’s clinical merit. Clearly, the
therapeutic index is a very rough measure
of safety and generally represents only the starting point in determin-ing
whether a drug is safe enough for human use. Usually, undesirable side effects
occur in doses lower than the lethal doses. For example, phenobarbital in-duces
drowsiness and an associated temporary neuro-logical impairment. Since
anticonvulsant drugs are in-tended to allow people with epilepsy to live normal
seizure-free lives, sedation is unacceptable. Thus, an im-portant measure of
safety for an anticonvulsant would be the ratio ED50 (neurological
impairment)/ED50 (seizure protection). This ratio is called a protective in-dex. The protective index
for phenobarbital is approxi-mately 3. It is easy to see that data derived from
dose–response curves can be used in a variety of ways to compare the clinical
usefulness of drugs. For instance, a drug with a protective index of 1 is
useless as an anti-convulsant, since the dose that protects against convul-sion
causes an unacceptable degree of drowsiness. A drug with a protective index of
5 would be a more promising anticonvulsant than one with an index of 2.
Graded Responses
More common than the quantal
dose–response rela-tionship is the situation in which a single animal (or
pa-tient) gives graded responses to graded doses; that is, as the dose is
increased, the response increases. With
graded responses, one can obtain a complete
dose– response curve in a single animal. A good example is the effect of the drug levarterenol (L-norepinephrine) on heart
rate.
Results of experiments with
levarterenol in guinea pigs are shown in Figure 2.3. The data are typical of
what one might obtain from constructing complete dose–response curves in each
of five different guinea pigs (a–e).
In animal a, a small increase in
heart rate oc-curs at a dose of 0.001 g/ kg body weight. As the dose is
increased, the response increases until at 1 ÎĽg/kg, the maximum increase of 80
beats per minute occurs. Further increases in dose do not produce greater
re-sponses. At the other extreme, in guinea pig e, doses be-low 0.3 ÎĽg/kg have no effect at all, and the maximum
re-sponse occurs only at about 100 ÎĽg/kg.
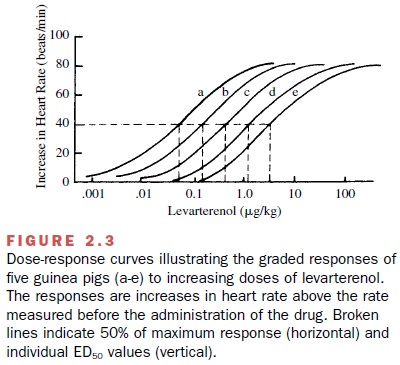
Since an entire dose–response
relationship is deter-mined from one animal, the curve cannot tell us about the
degree of biological variation inherent in a popula-tion of such animals.
Rather, variability is reflected by a family
of dose–response curves, such as those given in Figure 2.3. The ED50 in this type of dose–response
curve is the dose that produced 50% of the maximum re-sponse in one animal. In
guinea pig e, the maximum re-sponse
is an increase in heart rate of 80 beats per minute. Thus, 50% of the maximum
is 40 beats per minute. From Figure 2.3, it can be seen that the dose causing
this effect in guinea pig e is about
3 g/kg. The average sensitivity of all of the animals to levarterenol can be
estimated by combining the separate dose– response curves into a mean (average)
dose–response curve and then calculating the mean ED50. An estimate
of the variation within the population can be indicated by calculating a
statistical parameter, such as a confi-dence interval.
It is also possible to construct
quantal dose–response curves for drugs that produce graded responses. To do so,
one chooses a quantum of effect, for example, an in-crease in heart rate of 20
to 30 beats per minute above the control, or resting, rate. Doses of the drug
are then plotted against the frequency with which each dose pro-duces this
amount of effect. The resulting graph has the same characteristics as the graph
for the anticonvulsant activity of phenobarbital.
The doses in Figures 2.2 and
2.3 are on not an arith-metic but a logarithmic, or geometric, scale (i.e., the
doses are displayed as multiples). This is more apparent in Figure 2.3 because
of the greater range of doses. There are many reasons for the common practice
of us-ing geometric scales, some of which will become appar-ent later. One
important reason is that in most instances significant increases in response
gener-ally occur only when doses are increased in multiples. For example, in
Figure 2.3, curve e, if one increased
the dose from 10 to 11 or 12 ÎĽg/kg, the change in response would hardly be
measurable. However, if one increased it 3 times or 10 times (i.e., to 30 or
100 ÎĽg/kg), one could easily discern increased responses.
The concept of the
therapeutic index as a measure of the margin of safety has already been
discussed. In the ratio LD50/ED50, the ED50
can be obtained from either quantal (Fig. 2.2A) or graded (Fig. 2.3) dose–response curves. In the latter case,
it must be a mean ED50,
that is, the average ED50 obtained from several individuals.
Potency and Intrinsic Activity
Another drug characteristic that can be compared by use of ED50 values is potency. Figure 2.4 illustrates the mean dose–response curves of three hypothetical drugs that increase heart rate. Drugs a and b produce the same maximum response (an increase in heart rate of 80 beats per minute).
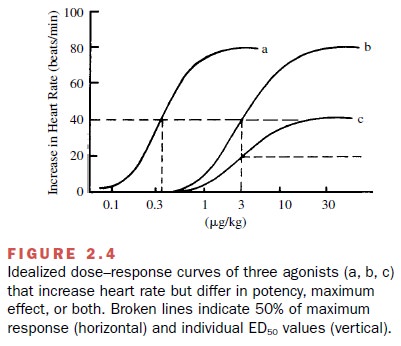
However, the fact that the dose–response curve for drug a lies to the left of the curve for drug b indicates that drug a
is more potent, that is, less of drug
a is needed to produce a given re-sponse.
The difference in potency is quantified by the ratio ED50b/ED50a: 3/0.3 10. Thus, drug a is 10 times as potent as drug b. In contrast, drug c has less maxi-mum effect than either
drug a or drug b. Drug c is said to have
a lower intrinsic activity than the
other two. Drugs a and b are full agonists with an intrinsic
activity of 1; drug c is called a partial agonist and has an intrin-sic
activity of 0.5 because its maximum effect is half the maximum effect of a or b.
The potency of drug c, how-ever, is
the same as that of drug b, because
both drugs have the same ED50 (3 ÎĽg/kg). The ED50 is the
dose pro-ducing a response that is one-half of the maximal re-sponse to that same drug.
It is important not to equate greater potency of a drug with
therapeutic superiority, since one might simply in-crease the dose of a less potent drug and
thereby obtain an identical therapeutic response. Such factors as the severity
and frequency of undesirable effects associated with each drug and their cost
to the patient are more relevant factors in the choice between two similar
drugs.
Related Topics