Design Problem, Important Question And Answer: Civil - Limit State Design Of Columns

Design Problems

1.Determine the load carrying capacity of a column of size 300 x 400 mm reinforced with six rods of 20 mm diameter i.e, 6-#20. The grade of concrete and steel are M20 and Fe 415 respectively. Assume that the column is short.

f_ck = 20 MPa, f_y= 415 MPa

Area of steel A_SC = 6 x ? x 20²/4 = 6 x 314 = 1884 mm² Percentage of steel = 100Asc/bD = 100x1884/300x400 = 1.57 % Area of concrete A_c = A_g - A_sc = 300 x 400 - 1884 = 118116 mm² Ultimate load carried by the column

P_u     = 0.4 f_ck A_c + 0.67 f_y A_sc

0.4x20x118116 + 0.67x415x1884

944928 + 523846 = 1468774 N = 1468. 8 kN

Therefore the safe load on the column = 1468.8 /1.5 = 979.2 kN

2.Determine the steel required to carry a load of 980kN on a rectangular column of size 300 x 400 mm. The grade of concrete and steel are M20 and Fe 415 respectively. Assume that the column is short.

f_ck = 20 MPa, f_y= 415 MPa, P = 980 kN Area of steel A_SC = ?

Area of concrete A_c = A_g - A_sc = (300 x 400 -  A_SC)

Ultimate load carried by the column

P_u     = 0.4 f_ck A_c + 0.67 f_y A_sc

980 x 1.5 x 1000 = 0.4x20x (300 x 400 -  A_SC) + 0.67x415 A_SC

= 960000 - 8 A_SC + 278.06 A_SC

A_SC  =1888.5 mm²,

Percentage of steel = 100Asc/bD = 100x1888.5 /300x400 = 1.57 % which is more than 0.8% and less than 6% and therefore ok.

Use 20 mm dia. bas, No. of bars = 1888.5/314 = 6.01 say 6

3.Design a square or circular column to carry a working load of 980kN. The grade of concrete and steel are M20 and Fe 415 respectively. Assume that the column is short.

Let us assume 1.0% steel (1 to 2%)

Say A_SC = 1.0% A_g =1/100 A_g = 0.01A_g f_ck = 20 MPa, f_y= 415 MPa, P = 980 kN

Area of concrete A_c = A_g - A_sc =  A_g -0.01A_g = 0.99 A_g

Ultimate load carried by the column P_u = 0.4 f_ck A_c + 0.67 f_y A_sc

980 x 1.5 x 1000 = 0.4x20x 0.99 A_g + 0.67x415 x 0.01A_g = 7.92 A_g + 2.78 A_g =10.7A_g

A_g  =  137383 mm²

Let us design  a square column:

B = D = ? A_g =370.6 mm say 375 x 375 mm

This is ok. However this size cannot take the minimum eccentricity of 20 mm as e_min/D = 20/375 =0.053 > 0.05. To restrict the eccentricity to 20 mm, the required size is 400x 400 mm.

Area of steel required is A_g = 1373.8 mm². Provide 4 bar of 22 mm diameter. Steel provided is 380 x 4 = 1520 mm²

Actual percentage of steel = 100A_sc/bD = 100x1520 /400x400 = 0.95 % which is more than 0.8% and less than 6% and therefore ok.

Design of Transverse steel:

Diameter of tie = ¼ diameter of main steel = 22/4 =5.5mm or 6 mm, whichever is greater. Provide 6 mm.

Spacing: < 300 mm, < 16 x22 = 352mm, < LLD = 400mm. Say 300mm c/c

Design of circular column:

Here A_g = 137383 mm²

? x D²/4 = A_g, D= 418.2 mm say 420 mm. This satisfy the minimum eccentricity of 20m Also provide 7 bars of 16 mm, 7 x 201 = 1407 mm²

Design of Transverse steel:

Dia of tie = ¼ dia of main steel = 16/4 = 4 mm or 6 mm, whichever is greater. Provide 6 mm.

Spacing: < 300 mm, < 16 x16 = 256 mm, < LLD = 420mm. Say 250 mm c/c

4.Design a rectangular column to carry an ultimate load of 2500kN. The unsupported length of the column is 3m. The ends of the column are effectively held in position and also restrained against rotation. The grade of concrete and steel are M20 and Fe 415 respectively.

Given:

f_ck = 20 MPa, f_y= 415 MPa,  P_u = 2500kN

Let us assume 1.0% steel (1 to 2%)

Say  A_SC = 1.0% A_g =1/100 A_g = 0.01A_g

Area of concrete A_c = A_g - A_sc =  A_g -0.01A_g = 0.99 A_g

Ultimate load carried by the column P_u = 0.4 f_ck A_c + 0.67 f_y A_sc

2500 x 1000 = 0.4x20x 0.99 A_g + 0.67x415 x 0.01A_g = 7.92 A_g + 2.78 A_g =10.7A_g

A_g  =  233645 mm²

If it is a square column:

B = D = ? A_g =483 mm. However provide rectangular column of size 425 x 550mm. The area provided=333750 mm²

Area of steel = 2336 mm², Also provide 8 bars of 20 mm, 6 x 314 = 2512 mm²

Check for shortness: Ends are fixed. l_ex = l_ey = 0.65 l = 0.65 x 3000 = 1950 mm

l_ex /D= 1950/550 < 12, and  l_ey /b = 1950/425 < 12, Column is short

Check for minimum eccentricity:

In the direction of longer direction

e_{min, x} = l_ux/500 + D/30 = 3000/500 + 550/30 = 24.22mm or 20mm whichever is greater.

e_{min, x} =  24.22 mm < 0.05D = 0.05 x 550 =27.5 mm. O.K

In the direction of shorter direction

e_{min, y}= l_uy/500 + b/30 = 3000/500 + 425/30 = 20.17 mm or 20mm whichever is greater.

e_{min, x} =  20.17 mm < 0.05b = 0.05 x 425 =21.25 mm. O.K

Design of Transverse steel:

Dia of tie = ¼ dia of main steel = 20/4 = 5 mm or 6 mm, whichever is greater. Provide 6 mm or 8 mm.

Spacing: < 300 mm, < 16 x20 = 320 mm, < LLD = 425mm. Say 300 mm c/c

5.Design a circular column with ties to carry an ultimate load of 2500kN. The unsupported length of the column is 3m. The ends of the column are effectively held in position but not against rotation. The grade of concrete and steel are M20 and Fe 415 respectively.

Given:

f_ck = 20 MPa, f_y= 415 MPa,  P_u = 2500kN

Let us assume 1.0% steel (1 to 2%)

Say  A_SC = 1.0% A_g =1/100 A_g = 0.01A_g

Area of concrete A_c = A_g - A_sc =  A_g -0.01A_g = 0.99 A_g

Ultimate load carried by the column P_u = 0.4 f_ck A_c + 0.67 f_y A_sc

2500 x 1000 = 0.4x20x 0.99 A_g + 0.67x415 x 0.01A_g = 7.92 A_g + 2.78 A_g =10.7A_g

A_g  =  233645 mm²

? x D²/4  = A_g, D = 545.4 mm say 550 mm.

Area of steel = 2336 mm², Also provide 8 bars of 20 mm, 6 x 314 = 2512 mm²

Check for shortness: Ends are hinged l_ex = l_ey =  l = 3000 mm

l_ex /D= 3000/550 < 12, and  l_ey /b = 3000/425 < 12, Column is short

Check for minimum eccentricity:

Here, e_{min, x} = e_{min, y} = l_ux/500 + D/30 = 3000/500 + 550/30 = 24.22mm or 20mm whichever is greater.

e_min  =  24.22 mm < 0.05D = 0.05 x 550 =27.5 mm. O.K

Design of Transverse steel:

Diameter of tie = ¼ dia of main steel = 20/4 = 5 mm or 6 mm, whichever is greater. Provide 6 mm or 8 mm.

Spacing: < 300 mm, < 16 x20 = 320 mm, < LLD = 550mm. Say 300 mm c/c

Similarly square column can be designed.

If the size of the column provided is less than that provided above, then the minimum eccentricity criteria are not satisfied. Then e_min is more and the column is to be designed as uni axial bending case or bi axial bending case as the case may be. This situation arises when more steel is provided ( say 2% in this case).

Try to solve these problems by using SP 16 charts, though not mentioned in the syllabus.

6.Design the reinforcement in a column of size 450 mm × 600 mm, subject to an axial load of 2000 kN under service dead and live loads. The column has an unsupported length of 3.0m and its ends are held in position but not in direction. Use M 20 concrete and Fe 415 steel.

Solution:

Given:  l_u= 3000 mm, b = 450 mm, D = 600 mm, P =2000kN, M20, Fe415

Check for shortness: Ends are fixed. l_ex = l_ey = l =  3000 mm

l_ex /D= 3000/600 < 12, and  l_ey /b = 3000/450< 12, Column is short

Check for minimum eccentricity:

In the direction of longer direction

e_{min, x} = l_ux/500 + D/30 = 3000/500 + 600/30 = 26 mm or 20mm whichever is greater. e_{min, x} = 26 mm < 0.05D = 0.05 x 600 =30 mm. O.K

In the direction of shorter direction

e_{min, y}= l_uy/500 + b/30 = 3000/500 + 450/30 = 21 mm or 20mm whichever is greater. e_{min, x} = 21 mm < 0.05b = 0.05 x 450 =22.5 mm. O.K

Minimum eccentricities are within the limits and hence code formula for axially loaded short columns can be used.

Factored Load

3

3000 × 10  = 0.4 × 20 × (450 × 600) + (0.67 × 415-0.4 × 20)A_sc

3

= 2160×10  + 270.05A_sc

? A_sc = (3000-2160) × 10 /270.05 = 3111 mm

In view of the column dimensions (450 mm, 600 mm), it is necessary to place intermediate

bars, in addition to the 4 corner bars:

2

Provide 4-25? at corners ie, 4 × 491 = 1964 mm

2

and 4-20? additional ie, 4 × 314 = 1256 mm

2             2

? A_sc = 3220 mm  > 3111 mm

? p = (100×3220) / (450×600) = 1.192 > 0.8 (minimum steel), OK.

Design of transverse steel

Diameter of tie = ¼ diameter of main steel = 25/4 =6.25 mm or 6 mm, whichever is greater. Provide 6 mm.

Spacing: < 300 mm, < 16 x 20 = 320 mm, < LLD = 450mm. Say 300 mm c/c Thus provide ties 8mm @ 300 mm c/c

Sketch:

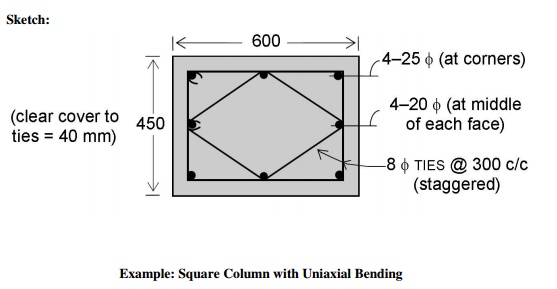
7.Determine the reinforcement to be provided in a square column subjected to uniaxial bending with the following data:

Size of column 450 x 450 mm

Concrete mix M 25

Characteristic strength of steel 415 N/mm²

Factored load 2500 kN

Factored moment 200 kN.m

Arrangement of reinforcement:

(a)On two sides

(b)             On four sides

Assume moment due to minimum eccentricity to be less than the actual moment Assuming 25 mm bars with 40 mm cover, d = 40 + 12.5 = 52.5 mm d¹/D = 52.5/450- 0.12

Charts for d¹/D = 0.15 will be used

P_u/f_ckbD = (2500 x 1000)/ (25 x 450 x 450) = 0.494

M_u/f_ckbD² =200 x 10⁶ /(25 x 450 x 450²) = 0.088

a) Reinforcement on two sides,

Referring to Chart 33, p/f_ck = 0.09

Percentage of reinforcement, p = 0.09 x 25 = 2.25 %

As = p bD/100 = 2.25 x 450 x 450/100 = 4556 mm²

b) Reinforcement on four sides from Chart 45,

p/f_ck = 0.10

p = 0.10 x 25 = 2.5 %

A_s = 2.5 x 450 x 450/100 = 5063 mm²

8.Example:  Circular Column with Uniaxial Bending

Determine the reinforcement to be provided in a circular column with the following data:

Diameter of column 500 mm Grade of concrete M20 Characteristic strength 250 N/mm² Factored load 1600 kN

Factored moment 125 kN.m

Lateral reinforcement :

(a)Hoop reinforcement

(b)             Helical reinforcement

(Assume moment due to minimum eccentricity to be less than the actual moment). Assuming 25 mm bars with 40 mm cover,

d¹ = 40 + 12.5 = 52.5 mm d¹/D - 52.5/50 = 0.105

Charts for d'/D = 0.10 will be used.

(a) Column with hoop reinforcement

P_u/f_ck D D = (1600 x 1000)/ (20 x 500 x 500) = 0.32

M_u/f_ck D x D² =125 x 10⁶ /(20 x 500 x 500²) = 0.05

Referring to Chart 52, for f_y = 250 N/mm² p/f_ck = 0.87

Percentage of reinforcement, p = 0.87 x 20 = 1.74 %

As = 1.74 x (? x 500²/4)/100 = 3416 mm²

(b) Column with Helical Reinforcement

According to 38.4 of the Code, the strength of a compression member with helical reinforcement is 1.05 times the strength of a similar member with lateral ties. Therefore, the, given load and moment should be divided by 1.05 before referring to the chart.

P_u/f_ck D D = (1600/1.05 x 1000)/ (20 x 500 x 500) = 0.31

M_u/f_ck D x D² =125/1.05 x 10⁶ /(20 x 500 x 500²) = 0.048

Hence, From Chart 52, for f_y = 250 N/mm²,

p/f_ck = 0.078

p = 0.078 x 20 = 1.56 %

As = 1.56 x( ? x 500 x 500/4 )/100 = 3063 cm²

According to 38.4.1 of the Code the ratio of the volume of helical reinforcement to the volume of the core shall not be less than

0.36 (A_g/A_c - 1) x f_ck /f_y

where A_g is the gross area of the section and A_c is the area of the core measured to the outside diameter of the helix. Assuming 8 mm dia bars for the helix,

Core diameter = 500 - 2 (40 - 8) = 436 mm

A_g/A_C  = 500/436  = 1.315

0.36 (A_g/A_c - 1) x f_ck /f_y = 0.36(0.315) 20/250 =0.0091

Volume of helical reinforcement / Volume of core

= A_sh ? x 428 /( ?/4 x 436²) s_h

0.9     A_sh / s_h

where, A_sh is the area of the bar forming the helix and s_h is the pitch of the helix. In order to satisfy the coda1 requirement,

0.09  A_sh / s_h  ? 0.0091

For 8 mm dia bar,

s_h ? 0.09 x 50 / 0.0091 = 49.7 mm. Thus provide 48 mm pitch

Example:  Rectangular column with Biaxial Bending

9.Determine the reinforcement to be provided in a short column subjected to biaxial bending, with the following data:

size of column = 400 x 600 mm Concrete mix = M15

Characteristic strength of reinforcement = 415 N/mm² Factored load, P_u = 1600 kN

Factored moment acting parallel to the larger dimension, M_ux =120 kNm Factored moment acting parallel to the shorter dimension, M_uy = 90 kNm Moments due to minimum eccentricity are less than the values given above.

Reinforcement is distributed equally on four sides.

As a first trial assume the reinforcement percentage, p = 1.2% p/f_ck = 1.2/15 = 0.08

Uniaxial moment capacity of the section about xx-axis :

d¹/D = 52.5 /600 = 0.088

Chart for d'/D = 0.1 will be used.

P_u/f_ck b D = (1600 x 1000)/ (15 x 400 x 600) = 0.444

Referring to chart 44

M_u/f_ck b x D² = 0.09

M_ux1 = 0.09 x 15 x 400 x 600²) = 194.4 kN.m

Uni-axial moment capacity of the section about yy axis :

d¹/D = 52.5 /400 = 0.131

Chart for d¹/D =0.15 will be used. Referring to Chart 45,

M_u/f_ck b x D² = 0.083

M_ux1 = 0.083 x 15 x 600 x 400²) = 119.52 kN.m

Calculation of P_uz :

Referring to Chart 63 corresponding to p = 1.2, f_y = 415 and f_ck = 15,

P_uz/A_g = 10.3

P_uz = 10.3 x 400 x 600 = 2472 kN

M_ux/M_ux1 = 120/194.4 =0.62

M_uy/M_uy1=90/119.52 = 0.75

P_u /P_uz =1600/2472 = 0.65       Referring to Churn 64, the permissible value of M_ux/M_ux1 corresponding to M_uy/M_uy1 and P_u /P_uz is equal to 0.58

The actual value of 0.62 is only slightly higher than the value read from the Chart.

This can be made up by slight increase in reinforcement.

Using Boris load contour equation as per IS:456-2000

P_u /P_uz = 0.65 thus, ?_n = 1 + [(2-1) / (0.8 - 0.2)]  (0.65-0.2) = 1.75

[0.62 ]^1.75 + [0.75]^1.75 = 1.04 slightly greater than 1 and slightly unsafe. This can be made up by slight increase in reinforcement say 1.3%

Thus provide As = 1.3x400x600/100 = 3120 mm²

Provide 1.3 % of steel p/f_ck = 1.3/15 = 0.086

d¹/D = 52.5 /600 = 0.088 = 0.1

From chart 44

M_u/f_ck b x D² = 0.095

M_ux1 = 0.095 x 15 x 400 x 600²) = 205.2  kN.m

Referring to Chart 45,

M_u/f_ck b x D² = 0.085

M_ux1 = 0.085 x 15 x 600 x 400²) = 122.4 kN.m

Chart 63 : P_uz/A_g = 10.4

P_uz = 10.4 x 400 x 600 = 2496 kN

M_ux/M_ux1 = 120/205.2 =0.585

M_uy/M_uy1=90/122.4  = 0.735

P_u /P_uz =1600/2496 = 0.641

Referring to Chart 64, the permissible value of M_ux/M_ux1 corresponding to M_uy/M_uy1 and P_u /P_uz is equal to 0.60

Hence the section is O.K.

Using Boris load contour equation as per IS:456-2000

P_u /P_uz = 0.641 thus, ?_n = 1 + [(2-1) / (0.8 - 0.2)]  (0.641-0.2) = 1.735

[120/205.2]^1.735 + [90/122.4]^1.735 = 0.981 ? 1 Thus OK

As = 3120 mm². Provide 10 bars of 20 mm dia. Steel provided is 314 x 10 = 3140 mm²

Design of transverse steel: Provide 8 mm dia stirrups at 300 mm c/c as shown satisfying the requirements of IS: 456-2000

10.Verify the adequacy of the short column section 500 mm x 300 mm under the following load conditions:

P_u = 1400 kN, M_ux = 125 kNm, M_uy = 75 kNm. The design interaction curves of SP 16

should be used. Assume that the column is a 'short column' and the eccentricity due to moments is greater than the minimum eccentricity.

Solution:

2

Given: D  = 500 mm, b = 300 mm, A = 2946 mm  M   = 125 kNm, M   = 75 kNm, f  = 25

x                                s                  ux                 uy              ck

MPa, f  = 415 MPa

y

Applied eccentricities

3

e_x = M_ux/P_u = 125 × 10 /1400 = 89.3 mm ? e_x/D_x = 0.179

3

e_y = M_uy/P_u = 75 × 10 /1400 = 53.6 mm ? e_y/D_y = 0.179

These eccentricities for the short column are clearly not less than the minimum eccentricities specified by the Code.

Uniaxial moment capacities: M_ux1, M_uy1

As determined in the earlier example, corresponding to P  = 1400 kN,

u

M       = 187 kNm

ux1

M_uy1 = 110 kNm Values of P_uz and ?_n

P_uz = 0.45f_ck A_g + (0.75f_y - 0.45f_ck)A_sc

= (0.45 × 25 × 300 × 500) + (0.75 × 415 - 0.45 × 25)×2946

= (1687500 + 883800)N = 2571 kN

? P_u/P_uz = 1400/2571 = 0.545 (which lies between 0.2 and 0.8)

? ?_n = 1.575

Check safety under biaxial bending

[125/187]^1.575 + [75/110]¹

= 0.530 + 0.547

1.077 > 1.0 Hence, almost ok.