Transitions between sub and super critical flow

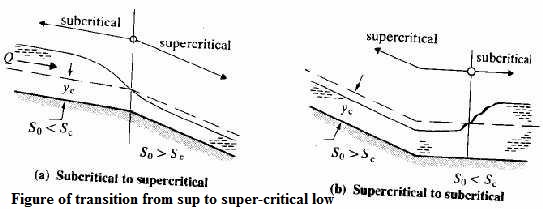
Transitions between sub and super critical flow

If sub critical flow exists in a channel of a mild slope and this channel meets with a steep channel in which the normal depth is super-critical there must be some change of surface level between the two. In this situation the surface changes gradually between the two. The flow in the joining region is known as gradually varied flow.

This situation can be clearly seen in the figure on the left below. Note how at the point of joining of the two channels the depth passes through the critical depth.

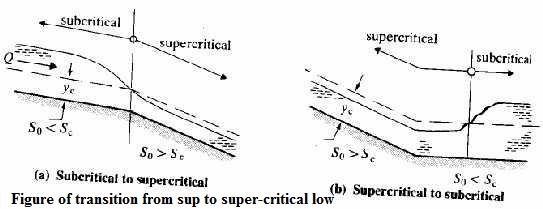
If the situation is reversed and the upstream slope is steep, super critical flow, and the down stream mild, sub-critical, then there must occur a hydraulic jump to join the two. There may occur a short length of gradually varied flow between the channel junction and the jump. The figure above right shows this situation:

Analysis of gradually varied flow can identify the type of profile for the transition as well as the position hydraulic jumps. The equations of gradually varied flow. The basic assumption in the derivation of this equation is that the change in energy with distance is equal to the friction loses.

This is the basic equation of gradually varied flow. It describes how the depth, y , changes with distance x , in terms of the bed slope S o , friction S f and the discharge, Q , and channels shape (encompassed in Fr and S f ).

Equations 1.25 and 1.26 are differential equations equating relating depth to distance. There is no explicit solution (except for a few special cases in prismatic channels). Numerical integration is the only practical method of solution. This is normally done on computers, however it is not too cumbersome to be done by hand.