Mechanical Properties and Deformation Mechanism

MECHANICAL PROPERTIES AND DEFORMATION MECHANISM

1 Plastic deformation

2 Brinell hardness est

3 Vickers hardness test

4 Rockwell hardness test

5 Charpy impact test

6 Fatigue test

7 Creep test

1.PLASTIC DEFORMATION

Plastic deformation is a change of the material dimensions remaining after removal of the load caused the deformation. Plastic deformations in metals occur by “slip” mechanism, illustrated in the picture:

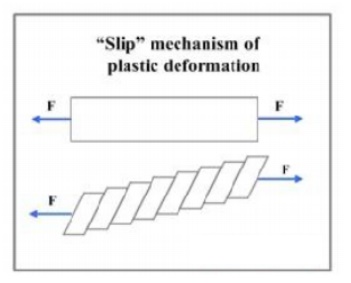
When the yield stress is achieved one plane of atoms in crystal lattice glides over another. Few parallel slip planes form a block, neighboring with another block. Thus movement of the crystal planes is resulted in a series of steps, forming slip bands - black lines viewed under optical microscope.

Slip occurs when the share resolved stress along the gliding planes reaches a critical value. This critical resolved shear stress is a characteristic of the material.

Certain metals (Zn and Sn) deform by a process of twinning, differing from the normal slip mechanism, where all atoms in a block move the same distance. In the deformation by twinning atoms of each slip plane in a block move different distance, causing half of the crystal lattice to become a mirror image of another half.

 In polycrystalline material directions of slips are different in different crystals. If a grain is oriented unfavorably to the stress direction its deformation is impeded. In addition to this grain boundaries are obstacles for the Slip movement as the slip direction should be changed when it crosses the boundary. As a result of the above strength of polycrystalline materials is higher, than that of mono-crystals.

 Slip and twinning processes, occurring during plastic deformation result in formation of preferred orientation of the grains. If the stress value required for a slip is higher than cohesion strength, metal fracture occurs. Stress- strain relations are considered in Tensile test and Stress-Strain Diagram.

Microscopically, plastic deformation is a result of permanent distortion of lattice by extensive rearrangement of atoms within it. There is an irreversible shear displacement of one part of the crystal relative to another in a definite crystallographic direction. This process is known as slip. Slip follows the path of least energy. It coincides to the direction in which atoms are most closely packed.

In a lattice, crystalline array of atoms are having linear imperfection, called dislocation. Slip is considered as step-by-step movement Of dislocation within a crystal. In well-annealed metals, density of dislocation is Not high enough to cause such macroscopic deformation. Therefore, there must be some mechanism that causes dislocations to multiply to a large number. Slip is one of such mechanisms in which dislocations reproduce themselves.

There are two types of dislocations: edge dislocation and screw dislocation. The edge dislocation moves across the slip plane in the direction of applied shear force. The direction of movement of screw dislocation is normal to the direction of slip step. When slip occurs by combination of the two types of dislocations, it results in a curved dislocation.

 Another mechanism of plastic deformation that occurs in Certain metals under certain circumstances is by twinning. In this process, atoms In each successive plane within a block move different distances. As a result The direction of the lattice is altered so that each half of the crystal becomes A mirror image of the other half along a twinning plane. In case of BCC structure, twinning occurs after some plastic deformation or when stress is applied quickly.

2.THE BRINELL HARDNESS TEST

 The Brinell hardness test method consists of indenting the test material with a 10 mm diameter hardened steel or carbide ball subjected to a load of 3000 kg. For softer materials the load can be reduced to 1500 kg or 500 kg to avoid excessive indentation. The full load is normally applied for 10 to 15 seconds in the case of iron and steel and for at least 30 seconds in the case of other metals. The diameter of the indentation left in the test material is measured with a low powered microscope. The Brinell harness number is calculated by dividing the load applied by the surface area of the indentation.

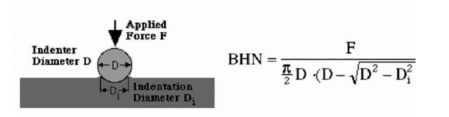
 The diameter of the impression is the average of two readings at right angles and the use of a Brinell hardness number table can simplify the determination of the Brinell hardness. A well structured Brinell hardness number reveals the test conditions, and looks like this, "75 HB 10/500/30" which means that a Brinell Hardness of 75 was obtained using a 10mm diameter hardened steel with a 500 kilogram load applied for a period of 30 seconds. On tests of extremely hard metals a tungsten carbide ball is substituted for the steel ball. Compared to the other hardness test methods, the Brinell ball makes the deepest and widest indentation, so the test averages the hardness over a wider amount of material, which will more accurately account for multiple grain structures and any irregularities in the uniformity o f the material. This method is the best for achieving the bulk or macro-hardness of a material, particularly those materials with heterogeneous structures.

3 VICKERS HARDNESS TEST

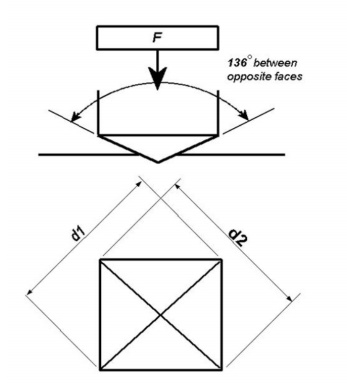
 The Vickers hardness test method consists of indenting the test material with a diamond indenter, in the form of a right pyramid with a square base and an angle of 136 degrees between opposite faces subjected to a load of 1 to 100kgf. The full load is normally applied for 10 to 15 seconds. The two diagonals of the indentation left in the surface of the material after removal of the load are measured using a microscope and their average calculated. The area of the sloping surface of the indentation is calculated. The Vickers hardness is the quotient obtained by dividing the kgf load by the square mm area of indentation.

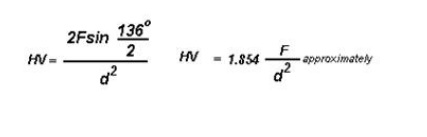
F= Load in kgf

d = Arithmetic mean of the two diagonals, d1 and d2 in mm HV = Vickers hardness

When the mean diagonal of the indentation has been determined the Vickers hardness may be calculated from the formula, but is more convenient to use conversion tables. The Vickers hardness should be reported like 800 HV/10, which means a Vickers hardness of 800, was obtained using a 10 kgf force. Several different loading settings give practically identical hardness numbers on uniform material, which is much better than the arbitrary changing of scale with the other hardness testing methods. The advantages of the Vickers hardness test are that extremely accurate readings can be taken, and just one type of indenter is used for all types of metals and surface treatments. Although thoroughly adaptable and very precise for testing the softest and hardest of materials, under varying loads, the Vickers machine is a floor standing unit that is more expensive than the Brinell or Rockwell machines.

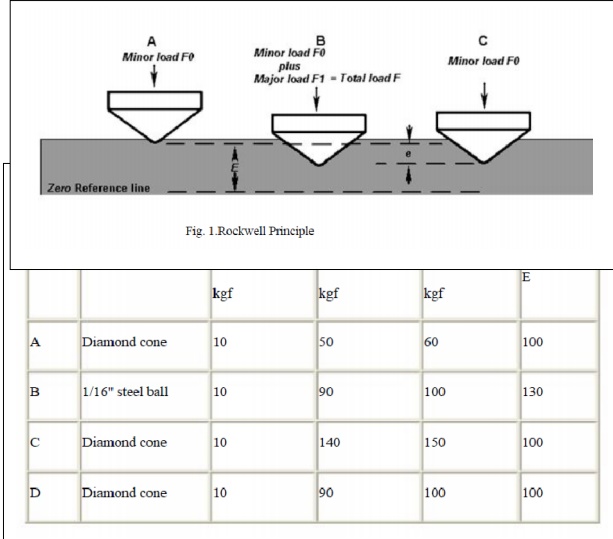
4.ROCKWELL HARDNESS TEST

The Rockwell hardness test method consists of indenting the test material with a diamond cone or hardened steel ball indenter. The indenter is forced into the test material under a preliminary minor load F0 (Fig. 1A) usually 10 kgf. When equilibrium has been reached, an indicating device, which follows the movements of the indenter and so responds to changes in depth of penetration of the indenter is set to a datum position. While the preliminary minor load is still applied an additional major load is applied with resulting increase in penetration (Fig. 1B). When equilibrium has again been reach, the additional major load is removed but the preliminary minor load is still maintained. Removal of the additional major load allows a partial recovery, so reducing the depth of penetration (Fig. 1C). The permanent increase in depth of penetration, resulting from the application and removal of the additional major load is used to calculate the Rockwell hardness number.

e = permanent increase in depth of penetration due to major load F1 measured in units of 0.002 mm

E = a constant depending on form of indenter: 100 units for diamond indenter, 130 units for steel ball indenter

HR = Rockwell hardness number

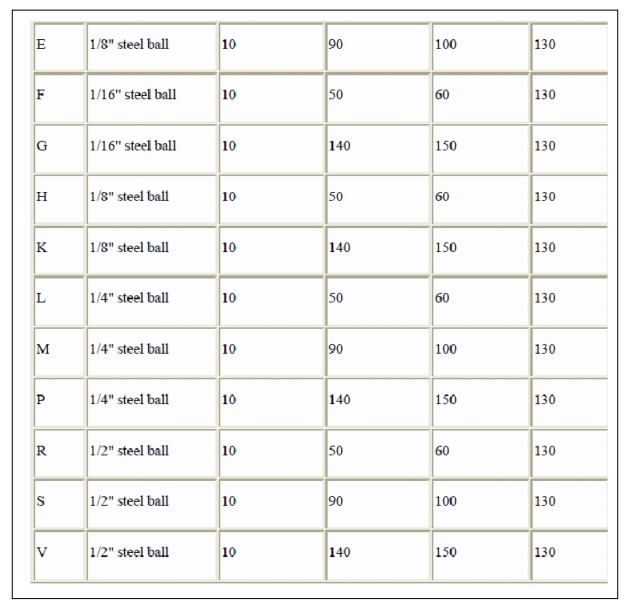
TYPICAL APPLICATION OF ROCKWELL HARDNESS SCALES HRA . . .      .

Cemented carbides, thin steel and shallow case hardened steel

HRB . . .         . Copper alloys, soft steels, aluminium alloys, malleable irons, etc.

HRC . . . . Steel, hard cast irons, case hardened steel and other materials harder than 100 HRB HRD . . . . Thin steel and medium case hardened steel and pearlitic malleable iron

HRE . . . . Cast iron, aluminium and magnesium alloys, bearing metals

HRF . . . . Annealed copper alloys, thin soft sheet metals

HRG . . . . Phosphor bronze, beryllium copper, malleable irons HRH . . . Aluminium,

zinc, lead

HRK . . . . } HRL . . . . }

HRM . . . .} . . . . Soft bearing metals, plastics and other very soft materials

HRP . . . . }

HRR . . . . }

HRS . . . . }

HRV . . . . }

 Advantages of the Rockwell hardness method include the direct Rockwell hardness number readout and rapid testing time. Disadvantages include many arbitrary non-related scales And possible effects from the specimen support anvil (try putting a cigarette paper under a test block and take note of the effect on the Hardness reading! Vickers and Brinell methods don't suffer from this effect).

5.CHARPY IMPACT TEST

 The Charpy impact test, also known as the Charpy v-notch test, is a standardized high strain-rate test which determines the amount of energy absorbed by a material during fracture. This absorbed energy is a measure of a given material's toughness and acts as a tool to study temperature- dependent brittle-ductile transition. It is widely applied in industry, since it is easy to prepare and conduct and results can be obtained quickly and cheaply. But a major disadvantage is that all results are only comparative.

 The apparatus consists of a pendulum axe swinging at a notched sample of material. The energy transferred to the material can be inferred by comparing the difference in the height of the hammer before and after a big fracture.

 The notch in the sample affects the results of the impact test, thus it is necessary for the notch to be of regular dimensions and geometry. The size of the sample can also affect results, since the dimensions determine whether or not the material is in plane strain.

This difference can greatly affect conclusions made.

 where all the aspects of the test and equipment used are described in detail.

Quantitative results

The quantitative result of the impact tests the energy needed to fracture a material and can be used to measure the toughness of the material and the yield strength. Also, the strain rate may be studied and analyzed for its effect on fracture.

The ductile-brittle transition temperature (DBTT) may be derived from the temperature where the energy needed to fracture the material drastically changes. However, in practice there is no sharp transition and so it is difficult to obtain a precise transition temperature. An exact DBTT may be empirically derived in many ways: a specific absorbed energy, change in aspect of fracture (such as 50% of the area is cleavage), etc.

Qualitative results

The qualitative results of the impact test can be used to determine the ductility of a material. If the material breaks on a flat plane, the fracture was brittle, and if the material breaks with jagged edges or shear lips, then the fracture was ductile. Usually a material does not break in just one way or the other, and thus comparing the jagged of the to flat surface areas fracture will give an estimate of the percentage of ductile and brittle fracture.

Sample sizes

According to ASTM A370,the standard specimen size for Charpy impact testing

 Subsize specimen sizes are:

is 10mm×10mm×55mm.

10mm×7.5mm×55mm, 10mm×6.7mm×55mm,

10mm×5mm×55mm,

10mm×3.3mm×55mm, 10mm×2.5mm×55mm.

Details of specimens as per ASTM A370 (Standard Test Method and Definitions for Mechanical Testing of Steel Products).

6.FATIGUE TEST

In materials science, fatigue is the progressive and localized structural damage that occurs when a material is subjected to cyclic loading. The nominal maximum stress values are less than the ultimate tensile stress limit, and may be below the yield stress limit of the material.

Fatigue occurs when a material is subjected to repeated loading and unloading. If the loads are above a certain threshold, microscopic cracks will begin to form at the surface. Eventually a crack will reach a critical size, and the structure will suddenly fracture. The shape of the structure will significantly affect the fatigue life; square holes or sharp corners will lead to elevated local stresses where fatigue cracks can initiate. Round holes and smooth transitions or fillets are therefore important to increase the fatigue strength of the structure.

Characteristics of fatigue

Fracture of an aluminium crank arm. Dark area of striation s: slow crack growth. Bright granular are a: sudden fracture.

• In metals and alloys, the process starts w ith dislocation movements,eventually forming persistent slip bands that nucleate short cracks.

• Fatigue is a st ochastic process, often showing considerable scatter even in controlled environments.

• The greate r the applied stress range, the shorter the life. F atigue life scatter tends to increase for longer fatigue lives. Damage is cumulative. Materials do not Recover when rested. Fatigue life is influence d by a variety of factors, su ch as temperature, surface finish, microstructure, presence of oxidizing or inert chemicals, residual stresses, contact (fretting), etc.

• Some materials (e.g.,some steel and titanium alloys) exhibit a theoretical fatigue limit below which continued loading does not lead to structural failure.

• In recent years, researchers have found that failures o ccur below the theoretical fatigue limit at very high fatigue lives (109 t o 1010 cycles). An ultrasoni c resonance technique is used in these experiments w ith frequencies around 10-20 kHz

• High cycle fatigue strength (about 103 to 108 cycles) can be described by load-controlled servo-hydraulic test rig is stress-basedpar ameters. A commonly us ed in these tests, with frequencies of aro und 20-50 Hz. Other sorts o f machines like resonant magnetic machines can also be used, achieving frequencies up to 250 Hz.

• Low cycle fatigue (typically less than 103 cycles) is associated with wide spread plasticity in metals; thus, a strain-based param eter should be used for fatig ue life prediction in metals and alloys . Testing is conducted with constant strain amplitudes typically at 0.01 -5 Hz.

FACTORS THAT AFFECT FATIGUE-LIFE

Cyclic stress state: Depending on the compl exity of the geometry and the loading, one or more properties of the stress state need t o be considered, such as stress am plitude, mean stress, biaxial ity, in- phase or out-of-phase shear stress, and load sequence,

 Geomet ry: Notches and variation in cross section throughout a part lead to stress concentrations where fatigue cracks initiate.

Surface quality: Surface roughness cause micro scopic stress concentrations that lower the fatigu e strength.

Compressive residual stresses can be introduced in the surface by e.g. shot peening to increase fatigue life. Such techniques for producing surface stress are often referred to as peening, whatever the mechanism used to produce the stress. Low Plasticity Burnishing, Laser peening, and ultrasonic i mpact treatment can also produce this surface compressive stress and can increase the fatigu e life of the component. This improvement is normally observed only for high-cycle f atigue.

aterial Type: Fatigue life, as well as the behavior during cyclic loading, varies widely for different m aterials, e.g. composites and polymers differ markedly from metals.

Residual stresses: Welding, cutting, ca sting, and other manufac turing processes involving heat or deformation can produce high levels of tensile residual stress, which decrea ses the fatigue strength.

Size and distribution of internal defects: Ca sting defects such as gas porosity, non-metallic inclusions and shrinkage voids can significantly reduce fatigue strength.

Direction of loading: For non-isotropic materials, fatigue strength depe nds on the direction of the principal stress.

Grain size: For most metals, smaller grains yield longer fatigue lives, however, the presence of surface defects or scratches w ill have a greater influence th an in a coarse grain ed alloy.

Environment: Environmental conditions can cause erosion, corros ion, or gas-phase embrittlement, which all affect fatigue life. Corrosion fatigue is a problem encountered in many aggres sive environments.

Temperature: Extreme high or low tempe ratures can decrease fatigu e strength.

DESIGN AGAINST FAT IGUE

Dependable design against fatigue-failure requires thorough Education and supervised experience in structural engineering,

mechanical engineering, or materials science. There are three principal

approaches to life assurance for mechanical parts that display increasing degrees

of sophistication:

 1. Design to keep stress below threshold of fatigue limit (infinite lifetime concept);

 2. Design (conservatively) for a fixed life after which the user is instructed to replace the part with a new one (a so-called Lifed part, finite lifetime concept, or "safe-life" design practice);

 3. Instruct the user to inspect the part periodically for cracks And to replace the part once a crack exceeds a critical length. This approach usually uses the technologies of nondestructive testing and requires an accurate prediction of the rate of crack-growth between inspections.

 This is often referred to as damage tolerant design or "retirement-for- cause".

STOPPING FATIGUE

 Fatigue cracks that have begun to propagate can sometimes be stopped by drilling holes, called drill stops, in the path of the fatigue crack This is not recommended as a general practice because the hole Represents a stress concentration factor which depends on the size of the Hole and geometry. There is thus the possibility of a new crack starting in the side of the hole. It is always far better to replace the cracked part entirely.

MATERIAL CHANGE

 Changes in the materials used in parts can also improve fatigue life. For example, parts can be made from better fatigue rated metals. Complete replacement and redesign of parts can also reduce if not eliminate fatigue problems. Thus helicopter rotor blades and propellers in metal are being replaced by composite equivalents. They are not only lighter, but also much more resistant to fatigue. They are more expensive, but the extra cost is amply repaid by their greater integrity, since loss of a rotor blade usually leads to total loss of the aircraft. A similar argument has been made for replacement of metal fuselages, wings and tails of aircraft.

7.CREEP TEST

Method for determining creep or stress relaxation behavior. To determine creep properties, material is subjected to prolonged constant tension or compression loading at constant temperature. Deformation is recorded at specified time intervals and a creep vs. time diagram is plotted. Slope of curve at any point is creep rate. If failure occurs, it terminates test and time for rupture is recorded. If specimen does not fracture within test period, creep recovery may be measured. To determine stress relaxation of material, specimen is deformed a given amount and decrease in stress over prolonged period of exposure at constant temperature is recorded Viscoplasticity is a theory in continuum mechanics that describes the rate-dependent inelastic behavior of solids. Rate-dependence in this context means that the deformation of the material depends on the rate at which loads are applied. The inelastic behavior that is the subject of viscoplasticity is plastic deformation which means that the material undergoes unrecoverable deformations when a load level is reached. Rate-dependent plasticity is important for transie nt plasticity calculations. The main difference between rate- independ ent plastic and viscoplastic material models is that the latter exhibit not only permanent deformations after the application of loads but continue to undergo a creep flow as a function of time under the influence of the applied load.

The elastic respon se of viscoplastic materials can be represented in one- dimension by Hookean spring elements. Rate- dependence can be represented by nonlinear dashpot elemen ts in a manner similar to viscoelasticity. Plasticity can be accounted for by adding sliding frictional elements as shown in Figure 1.In the figure E is the modulus of elasticity, λ is the viscosity parameter and N is a power-law type parameter that represents non-linear dashpot σ(dε/dt)= σ = λ(dε/dt)(1/ N)]. The sliding element can have a yield stress (σy) that is strain rate dependent, or e ven constant, Viscoplasticity is usually modeled in three -dimensions using overstress models of the Perzyna or Duvaut-Lions types. In these models, the stress is allo wed to increase beyond the rate-independent yield surface upon application of a load and then allowed to relax back to the yield surface over time. The yield surrface is usually assumed not to be rate-dependent in such models. An alternativ e approach is to add a strain rate depen dence to the yield stress and use the techhniques of rate independent plasticity to calculate the response of a material For metals and alloys, viscoplasticity is the macroscopic behavior caused by a mechanism linked to the movement of dislocation s in grains, with superposed effects of inter-crystalline gliding. The mechanism usually becomes dominant at temperatur es greater than approximately one third o f the absolute melting temperature. However, certain alloys exhibit viscoplasticity at room temperature (300K). For polymer s, wood, and bitumen, the theory of viscoplasticity is required to describe beha vior beyond the limit of elasticity or viscoelasticity.

In general, viscoplasticity theories are useful in areas such as the calculation of permanent deformations, the prediction of the plastic collapse of structures, the investigation of stability, crash simulations, systems exposed to hig h temperatures such as turbines in engines, e.g. a power plant, dynamic problem s and systems exposed to high strain rates. dels of rate-independent plasticity that have a rate-dependent yield stress.

Creep is the tendency of a solid material to slowly move or defor m permanently under constant stresses. Creep tests measure the strain response due to a constant stress as shown in Figure 3. The classical creep curve represents the evolution of strain as a function of time in a material subjected to uniaxial stre ss at a constant temperature. The creep test, for instance, is performed by apply ing a constant force/stress and analyzing the strain response of the system. In gener al, as shown in Figure 3b this curve usually shows three phases or periods of behavior A primary creep stage, also known as transient creep, is the starti ng stage during which hardening of the material leads to a decrease in the rate of flow which is initially very high.

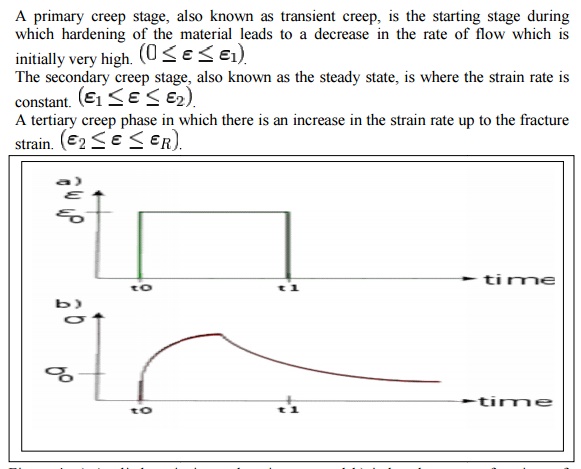
Figure 4. a) Applied strai n in a relaxation test and b) induced stress as functions of time over a short period for a viscoplastic material.

As shown in Figure 4, th e relaxation test is defined as the stress res ponse due to a constant strain for a p eriod of time. In viscoplastic materials, relaxation tests demonstrate the stress rel axation in uniaxial loading at a constant strain. In fact, these tests characterize the viscosity and can be used to determine the relation which exists between the stress and th e rate of viscoplastic strain. The decomposit on of strain rate is

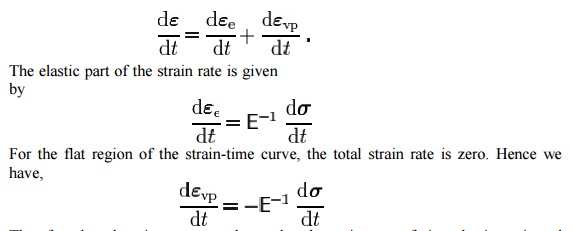
Therefore the relaxation curve can be used to determine rate of viscoplastic strain and hence the viscosity of the dashpot in a one-dimensional viscoplastic material model. The residual value that is reached when the stress has plateaued a t the end of a relaxation test corresponds to the upper limit of elasticity. For some m aterials such as rock salt such an upper limit of elasticity occurs at a very small val ue of stress and relaxation tests can be co ntinued for more than a year without any ob servable plateau in the stress.

It is important to note that relaxation tests are extremely difficult to perform because

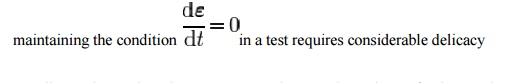
Tensile testing, also known as  tension  testing, is a fundamental materials science test in which a sa mple is subjected to uniaxial tension until fail ure. The results from the test are commo nly used to select a material for an application, for quality control, and to predict how a material will react under other t ypes of forces. Properties that are directly measured via a tensile test are ultimate t ensile strength, maximum elongation and reduction in area. From these m easurements the following properties can also be determined: Young's modulus, Poisson's ratio, yield strength, and strain-harde ning characteristics.

Tensile specimens made from an aluminum alloy. The left two sp ecimens have a round cross-section and threaded shoulders. The right two are flat specimen designed to be used with s errated grips.

A tensile specimen is a standardized sample cross-section. It has two shoulders and a gage section in between. The shoulders are large so they can be readily gripped, where as the gage section has a smaller cross-section so that the deformation and failure can occur in this area.

The shoulders of the test specimen can be manufactured in various ways to mate to various grips in the testing machine (see the image below). Each system has advantages and disadvantages; for example, shoulders designed for se rrated grips are easy and cheap to manuufacture, but the alignment of the specim en is dependent on the skill of the tech nician. On the other hand, a pinned gri p assures good alignment. Threaded sh oulders and grips also assure good alig nment, but the technician must know to thread each shoulder into the grip at least one diameter's length, otherwise the threads can strip before the specimen fractures.

In large castings and forgi ngs it is common to add extra material, which is designed to be removed from the casting so that test specimens can be made from it. These specimen not be exact representation of the whole workpiece be cause the grain structure may be different throughout. In smaller workpieces or when critical parts of the casting must be te sted, a workpiece may be sacrificed to make the test specimens.For workpieces that are machined from bar stock, the test specimen can be made from the same piece as the bar stock.

The repeatability of a testing machine can be found by using special test specimens meticulously made to be a s similar as possible.