Book Back Questions with Answers

Laws of Motion - Science

I. Choose the correct answer

1) Inertia of a body depends on

a) weight of the object

b) acceleration due to gravity of the planet

c) mass of the object

d) Both a & b

2) Impulse is equals to

a) rate of change of momentum

b) rate of force and time

c) change of momentum

d) rate of change of mass

3) Newton’s III law is applicable

a) for a body is at rest

b) for a body in motion

c) both a & b

d) only for bodies with equal masses

4) Plotting a graph for momentum on the X-axis and time on Y-axis. slope of momen-tum-time graph gives

a) Impulsive force

b) Acceleration

c) Force

d) Rate of force

5) In which of the following sport the turning of effect of force used

a) swimming

b) tennis

c) cycling

d) hockey

6) The unit of ‘g’ is m s-2. It can be also expressed as

a) cm s-1

b) N kg-1

c) N m2 kg-1

d) cm2 s-2

7) One kilogram force equals to

a) 9.8 dyne

b) 9.8 × 104 N

c) 98 × 104 dyne

d) 980 dyne

8) The mass of a body is measured on planet Earth as M kg. When it is taken to a planet of radius half that of the Earth then its value will be____kg

a) 4 M

b) 2M

c) M/4

d) M

9) If the Earth shrinks to 50% of its real radius its mass remaining the same, the weight of a body on the Earth will

a) decrease by 50%

b) increase by 50%

 decrease by 25%

d) increase by 300%

10) To project the rockets which of the follow-ing principle(s) is /(are) required?

a)              Newton’s third law of motion

b)             Newton’s law of gravitation

c)              law of conservation of linear momentum

d)             both a and c

II. Fill in the blanks

1. To produce a displacement force is required

2. Passengers lean forward when sudden brake is applied in a moving vehicle. This can be explained by inertia of motion

3. By convention, the clockwise moments are taken as negative and the anticlockwise moments are taken as positive

4. Gear is used to change the speed of car.

5. A man of mass 100 kg has a weight of 980 N at the surface of the Earth

III. State whether the following statements are true or false. Correct the statement if it is false:

1. The linear momentum of a system of particles is always conserved. - False

In the absence of external force, the linear momentum of a system of particle is always conserved.

2. Apparent weight of a person is always equal to his actual weight - False

Both apparent weight and actual weight can be greater or lesser according to the movement of the person inside the lift.

3. Weight of a body is greater at the equator and less at the polar region. - False

Weight of the body is less at equator, more at polar region.

4. Turning a nut with a spanner having a short handle is so easy than one with a long handle.  - False

Turning effect (i.e torque) depends on perpendicular distance of the line of action of the applied force N = F x d

5. There is no gravity in the orbiting space station around the Earth. So the astronauts feel weightlessness. - False

When space station and astronauts have equal acceleration, they are under free fall condition, so both astronaut and space station are in the state of weightlessness.

IV. Match the following

 Column I Column II

 a. Newton’s I law - propulsion of a rocket

 b. Newton’s II law - Stable equilibrium of a body

 c. Newton’s III law - Law of force

 d. Law of conservation of Linear momentum - Flying nature of bird

Answer:

(a) Newton's law - stable equilibrium of a body

(b) Newton's II law - Law of force

(c) Newton's III law - Flying nature of bird

(d) Law of conservation of linear momentum - propulsion of a rocket

V. Assertion & Reasoning

Mark the correct choice as

a) If both the assertion and the reason are true and the reason is the correct explanation of assertion.

b) If both the assertion and the reason are true, but the reason is not the correct ex-planation of the assertion.

c) Assertion is true, but the reason is false.

d) Assertion is false, but the reason is true.

1. Assertion: The sum of the clockwise moments is equal to the sum of the anticlockwise moments.

Reason: The principle of conservation of momentum is valid if the external force on the system is zero.

Answer: b) If both the assertion and the reason are true, but the reason is not the correct explanation of the assertion.

2. Assertion: The value of ‘g’ decreases as height and depth increases from the surface of the Earth.

Reason: ‘g’ depends on the mass of the object and the Earth.

Answer: c) Assertion is true, but the reason is false.

VI. Answer briefly.

1. Define inertia. Give its classification.

The inherent property of the objects to resist any change in its state of rest or the state of uniform motion unless it is influenced upon by an external unbalanced force is known as ’’inertia”.

2. Classify the types of force based on their application.

They are two types of force

(i) Contact force : A force which is applied by means of direct physical contact between two objects is known as " contact force". Examples : pushing or pulling an object, muscular force, frictional force, compressing a spring, kicking a football, etc.

(ii) Non - contact force i A force which is applied without any physical contact between the objects is known as "non- contact force". Examples : gravitational force, magnetic force electromagnetic force, etc.

3. If a 5 N and a 15 N forces are acting opposite to one another. Find the resultant force and the direction of action of the resultant force

F1 = 5 N ;

F2 = 15N

R = F1 + (-F2)

= F1-F2

= 5-15

Resultant force = -10N

Resultant force of -10 N is acting in the direction of 15 N.

4. Differentiate mass and weight.

Mass

1. It is the quantity of matter contained in the body

2. Mass is a scalar quantity

3. It's unit is kg (kilogram)

4. Mass of a body remains the same at any point on the earth

5. Mass can be measured using a physical balance

Weight

1. It is the gravitational force exerted on a body due to the earth's gravity alone.

2. Weight is a vector quantity

3. It's unit is N (newton)

4. Weight of a body varies from one place to another place on the earth

5. Weight can be measured using a spring balance

5. Define moment of a couple.

Couple: Two equal and unlike parallel forces applied simultaneously at two distinct points constitute a couple. The line of action of the two forces does not coincide. It does not produce any translatory motion since the resultant is zero. But, a couple results in causes the rotation of the body. Rotating effect of a couple is known as moment of a couple.

Examples: Turning a tap, winding or unwinding a screw, spinning of a top, etc.

6. State the principle of moments.

Principle of moments states that when a number of like or unlike parallel forces act on a rigid body and the body is in equilibrium then the algebraic sum of moments in clockwise direction is equals to the algebraic sum of moments in anticlockwise direction.

Moment in clockwise direction

= Moment in anticlockwise direction

F1 x d1 = F2 x d2

7. State Newton’s second law.

The force acting on a body is directly proportional to the rate of change of linear momentum of the body and the change in momentum takes place in the direction of force.

F = m x a

Force = mass x acceleration

8. Why a spanner with a long handle is preferred to tighten screws in heavy vehicles?

The turning effect of a body depends upon the distance of the line of action of the applied force from the axis of rotation. Larger the perpendicular distance, lesser is the force required to turn the body. So spanner with long handle is preferred.

9. While catching a cricket ball the fielder lowers his hands backwards. Why?

When the fielder pulls back his hands he experiences a smaller force for a longer interval of time leading to less damage to his hands.

10. How does an astronaut float in a space shuttle?

(i) Both the astronauts and the space craft experience the same gravitational force of the earth.

(ii) There is no weight of the astronauts exerted on the surface of the space craft.

(iii) In turn, there is no reaction force exerted by the surface of the space craft on the astronauts.

(R = 0). So they float. 

VII. Solve the given problems

1. Two bodies have a mass ratio of 3:4 The force applied on the bigger mass produces an acceleration of 12 ms-2.What could be the acceleration of the other body, if the same force acts on it.

Given

Mass ratio of two bodies is 3 : 4

So let's assume

Mass of smaller body = m1 = 3 kg

Mass of bigger body = m2 = 4 kg

Acceleration due to force applied by bigger body = a2 = 12 ms-2

To Find: Acceleration due to the same force on the smaller body = a1 = ?

Solution

According to Newton's second law of motion.

F = m x a

F1 = m1a1

F2 = m2a2

F1 = 3a1,

F2 = 4 X 12 = 48N

As the force is the equal

F1 = F2

3a1 = 48

a1 = 48 /3 = 16 ms-2

So acceleration due to the same force on the smaller body a1  = 16 ms-2

2. A ball of mass 1 kg moving with a speed of 10 ms-1 rebounds after a perfect elastic collision with the floor. Calculate the change in linear momentum of the ball.

Given

Mass, m = 1 kg

Initial velocity, u =10 ms-1

Final velocity, v = -10ms-1

To find

Change in linear momentum

= m(v-u) = mv - mu

Solution

Momentum before collision = mu

= (1x10)

= 10 kg ms-1

Momentum after collision = mv

= - (1x10)

= - 10 kg ms-1

Change in momentum = mv - mu

= - 10 - 10

= -20 kg ms-1

3. A mechanic unscrew a nut by applying a force of 140 N with a spanner of length 40 cm. What should be the length of the spanner if a force of 40 N is applied to unscrew the same nut?

Given

Force F1 = 140 N

Length L1 = 40 cm

= 40 x 10-2 m

Force, F2 = 40 N

Length, L2 = ?

To find: F1 x L1 = F2 x L2

Length of the spanner,

 L2 = [ F1 x L1 ] / F2

Solution

L2 = [ 140 x 40 x 10-2 ] / 40 = 140 x 10-2

Length, L2 = 1.4m

4. The ratio of masses of two planets is 2:3 and the ratio of their radii is 4:7 Find the ratio of their accelerations due to gravity.

The radio of masses of two bodies is m1: m2  i.e., 2:3

Mass of the smaller body, m1 = 2 kg

Mass of the bigger body m2 = 3 kg

Radius of the smaller body, R1 = 4 km

Radius of the bigger body, R2 = 7 km

i.e., r1 : r2 = 4:7

Solution

We know that GM/R2

The ratio is, g1 : g2 = 49 : 24

VIII. Answer in detail.

1. What are the types of inertia? Give an example for each type.

Answer:

(i) Inertia of rest : The resistance of a body to change its state of rest is called inertia of rest.

Ex : When you vigorously shake the branches of a tree some of the leaves and fruit are detached and they fall down.

(ii) Inertia of motion : The resistance of a body to change its state of motion is called inertia of motion.

Ex : An athlete runs some distance before jumping. Because, this will help him jump longer and higher.

(iii) Inertia of direction : The resistance of a body to change its direction of motion is called inertia of direction.

Ex : When you make a sharp turn while driving a car, you tend to lean sideways.

2. State Newton’s laws of motion?

Answer:

(i) Newton's First law: Every object continues to be in its state of rest or the state of uniform motion along a straight line unless it is acted upon by some external force.

(ii) Newton's second law of motion: The force acting on an object is directly proportional to the rate of change of linear momentum of the object and the change in momentum takes place in the direction of force.

This law helps us to measure the amount of force. So it is called as "law of force".

(iii) Newton's third law of motion : Newton's third law states that "for every action there is an equal and opposite reaction. They always act on two different bodies".

3. Deduce the equation of a force using Newton’s second law of motion.

Answer:

(i) Let "m" be the mass of a moving body, moving along a straight line with an initial speed 'u' ms^-1.

(ii) After a time interval of 't'second, the velocity of the body changes to 'v' ms^-1 due to the impact of unbalanced external force F.

(iii) Initial momentum of the body → P_i = mu

(iv) final momentum of the body → P_f = mv

 (v) Change in momentum → Δp

 = P_f - P_i

 = mv - mu

By Newton's second law of motion,

Force, F α rate of change of momentum

F α change in momentum / time

F α (mv - mu) /t

F = k m (v - u) t

Here, k is the proportionality constant.

k = 1 in all system of units. Hence,

F = m (v - u) / t

Since acceleration = change in velocity / time,

a = (v - u) / t.

(vi) Hence, we have F = m × a

Force = mass × acceleration

4. State and prove the law of conservation of linear momentum.

Answer:

The principle of conservation of linear momentum states that "There is no change in the linear momentum of a system of bodies as long as no net external force acts on them"

Let us prove the law of conservation of linear momentum with the following illustration:

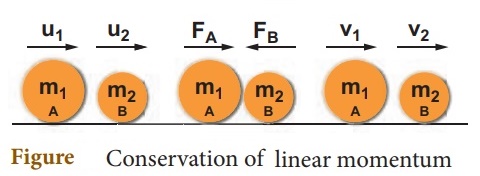
Conservation of linear momentum

Proof:

Let two bodies A and B having masses m₁ and m₂ move with initial velocity u₁ and u₂ in a straight line. Let the velocity of the first body be higher than that of the second body. i.e., u₁>u₂. During an interval of time t second, they tend to have a collision. After the impact, both of them move along the same straight line with a velocity v₁ and v₂ respectively.

Force on body B due to A,

F_B = m₂(v₂ - u₂) / t

Force on body A due to B,

F_A = m₁ (v₁ – u₁) / t

By Newton’s III law of motion,

Action force = Reaction force

F_A = –F_B

m₁ (v₁ – u₁) / t = - m₂ (v₂ - u₂) / t

m₁ v₁ + m₂v₂ = m₁u₁ + m₂u₂      ……. (A)

The above equation confirms in the absence of an external force, the algebraic sum of the momentum after collision is numerically equal to the algebraic sum of the momentum before collision.

Hence the law of conservation of linear momentum is proved.

5. Describe rocket propulsion.

Answer:

(i) Propulsion of rockets is based on law of conservation of linear momentum as well as Newton's III law of motion.

(ii) Rockets are filled with a fuel (either liquid or solid) in the propeller.

(iii) When the rocket is fired, this fuel is burnt and a hot gas is ejected with high speed from the back nozzle producing a huge momentum.

(iv) To balance this momentum, an equal and opposite reaction force is produced which makes the rocket project forward.

(v) While in motion, mass of the rocket gradually decreases until the fuel is completely burnt out. Since there is no net external force acting on it, the linear momentum of the system is conserved.

(vi) The mass of the rocket decreases by altitude that results gradual increase in velocity of the rocket.

(vii) At one stage, it reaches a velocity which is sufficient to just escape from the gravitational pull of the Earth. This velocity is called escape velocity.

6. State the universal law of gravitation and derive its mathematical expression

Answer:

Newton’s Universal law of gravitation : This law states that every particle of matter in this universe attracts every other particle with a force. This force is directly proportional to the product of their masses and inversely proportional to the square of the distance between centers of these masses. The direction of the force acts along the line joining the masses

Deviation: Let m₁ and m₂ be the masses of two bodies A and B placed at r meter apart in space

Force F ∝ m₁ × m₂

F ∝ 1/r²

On combining the above two expressions,

F ∝ [ m₁ × m₂ ] / r²

F = [G m₁ × m₂ ]/r² or ( G m₁m₂ ) / r²

Where G is universal gravitational constant. Its value in SI unit is 6.674 x 10^-11 Nm² kg^-2.

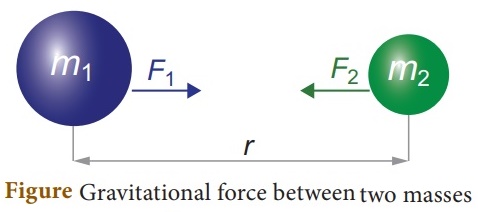
Gravitational force between two masses

7. Give the applications of universal law gravitation.

Answer:

Application of Newton’s law of gravitation

(i) Dimensions of the heavenly objects can be measured using gravitation law. Mass of the earth, radius of the earth, acceleration due to gravity etc. can be calculated with a higher accuracy.

(ii) Helps in discovering new stars and planets. Mass of the double stars can be calculated.

(iii) One of the irregularities in the motion of stars is called "Wobble" which leads to the disturbance in the motion of planet nearby. In this condition mass of the star can be calculated using law of gravitation.

(iv) Helps to explain germination of roots due to the property of geotropism, which is the property of root responding to the gravity.

(v) Helps to predict the path of the astronomical bodies.

IX. HOT Questions

1. Two blocks of masses 8 kg and 2 kg respectively lie on a smooth horizontal surface in contact with one other. They are pushed by a horizontally applied force of 15 N. Calculate the force exerted on the 2 kg mass.

Given

Mass of block 1, m1 = 8

Mass of block 2, m2 = 2kg

Total mass, m = m1 + m2

m = 8 +2 = 10

Force, F1 = 15N

To find:

Force exerted on 2 kg, F2= ?

Solution

Force, F1 = mass x acceleration

F1 = ma

F1 = 10 x a

a = F1/10 = 15/10 = 1.5 ms-2

Force exerted on m2 (2 kg) is,

F2 = m2a

= 2 x 1.5

F2 =3N

2. A heavy truck and bike are moving with the same kinetic energy. If the mass of the truck is four times that of the bike, then calculate the ratio of their momenta. (Ratio of momenta = 1:2

Given

Kinetic energy of the truck =Kinetic energy of the bike

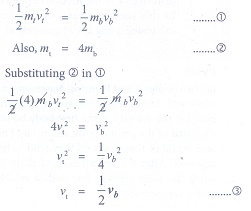
To find:

Ratio of the momenta, i.e mtvt : mbvb

Solution:

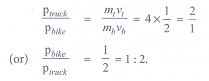
3. “Wearing helmet and fastening the seat belt is highly recommended for safe journey” Justify your answer using Newton’s laws of motion.

(i) According to Newton’s second law, when you fall from a bike on the ground with a force equal to your mass and acceleration of the bike and according to Newton’s third law, an equal and opposite reacting force on the ground is exerted on you body or hear. When you do not wear a helmet, this reacting force can cause fatal head injuries. So it is important to wear helmet for a safe journey.

(ii) Consider for instance of an unfortunate collision of a car with another car (or any obstacles in its path). Upon contact with an obstacle, an unbalanced force acts upon the car to abruptly decelerate it to rest. The passengers in the car would also be decelerated to rest if strapped to the seats by seat belts.

(iii) If not strapped to the seats, they no longer share the same state of rest as the car. According to Newton’s first law, the passenger in the car are more likely to maintain the same state of motion, which will result in the banging the glass (wind shield) or thrown away in the front by breaking the glass windshield. In case of the driver, he might bang himself in the steering. So wearing seatbelts is highly recommended for a safe journey.