Chapter: Mechanical : Engineering Thermodynamics : Ideal And Real Gases, Thermodynamic Relations
Ideal and Real Gases, Thermodynamic Relations
IDEAL AND REAL GASES, THERMODYNAMIC
RELATIONS
IDEAL GAS
An ideal gas is
a theoretical gas composed of a set of randomly-moving point particles that
interact only through elastic collisions. The ideal gas concept is useful
because it obeys the ideal gas law, a simplified equation of state, and is
amenable to analysis under statistical mechanics.
At normal ambient
conditions such as standard temperature and pressure, most real gases behave
qualitatively like an ideal gas. Generally, deviation from an ideal gas tends
to decrease with higher temperature and lower density, as the work performed by
intermolecular forces becomes less significant compared with the particles'
kinetic energy, and the size of the molecules becomes less significant compared
to the empty space between them.
EQUATION
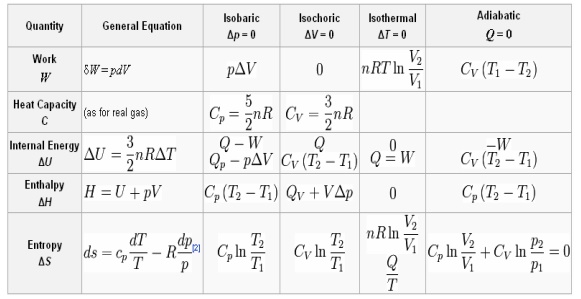
TABLE FOR AN IDEAL GAS
REAL GAS
Real
gas,
as opposed to a Perfect or Ideal Gas, effects refers to an assumption
base where the following are taken into account:
•Compressibility effects
•Variable heat capacity
•Van der Waals forces
•Non-equilibrium thermodynamic effects
•Issues with molecular
dissociation and elementary reactions with variable composition.
For most applications,
such a detailed analysis is "over-kill" and the ideal gas
approximation is used. Real-gas models have to be used near condensation point
of gases, near critical point, at very high pressures, and in several other
less usual cases.
VAN DER WAALS MODELISATION
Real gases are often
modeled by taking into account their molar weight and molar volume
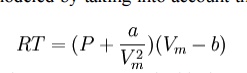
Where
P is the pressure, T is the temperature, R the ideal gas constant, and Vm the
molar volume. a and b are parameters that are determined empirically for each
gas, but are sometimes estimated from their critical temperature (Tc) and
critical pressure (Pc) using these relations:
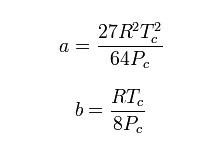
REDLICH–KWONG MODELISATION
The
Redlich–Kwong equation is another two-parameters equation that is used to
modelize real gases. It is almost always more accurate than the Van der Waals
equation, and often more accurate than some equation with more than two
parameters. The equation is
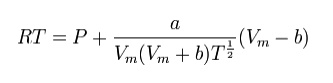
THERMODYNAMICS
RELATIONS
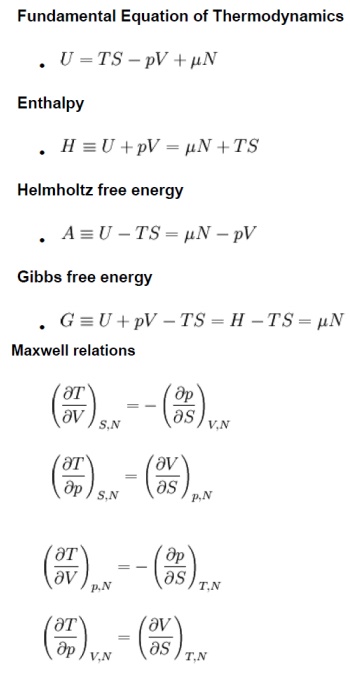
Maxwell relations.
The Maxwell’s equations relate entro properties p,v
and T for pure simple compressible substances.
From first law of thermodynamics,
Q = W + U
Rearranging the parameters
Q = U + W since [ds = ,
W = pdv ]
Tds = du +pdv
du = Tds –pdv -----------
(1)
We know that, h = u + pv
dh = du + d(pv)
= du + vdp + pdv -----------
(2)
Substituting the value du in equation (2),
dh = Tds + pdv + vdp –pdv
dh = Tds + vdp -----------
(3)
By
Helmotz’s function,
a = u –Ts
da = du –d(Ts)
= du –Tds –sdT -----------
(4)
Substituting the values of du in equation (4),
da = Tds –pdv –Tds –sdT
T = –pdv –sdT -----------
(5)
By Gibbs functions,
G = h –Ts
dg = dh –d(Ts)
dg = dh –Tds –sdT -----------
(6)
Substituting the value of dh in equation (6),
So, dg becomes
dg = Tds + vdp –Tds –sdT
dg = vdp –sdT -----------
(7)
By inverse exact differential we can write equation
(1) as, du = Tds –pdv
----------- (8)
Similarly, equation (3) can be written as, dh = Tds
+ vdp
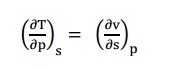 ----------- (9)
----------- (9)
Similarly, equation (5) can be written as,

These
equations 8, 9, 10 and
11 are Maxwell’s
e

Tds relations in
terms of temperature and pressure changes and temperature and volume changes.
The entropy (s) of pure substance can be expressed
as a function of temperature (T) and pressure (p).
s
= f(T,p)
We
know that,
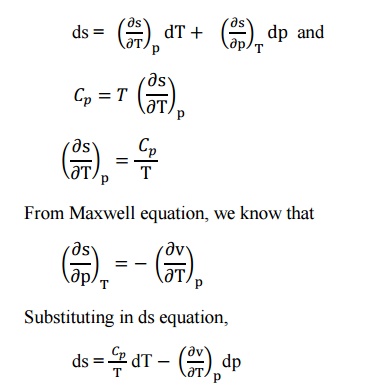
This
is known as the first form of entropy equation or the first Tds equation. By
considering the entropy of a pure substance as a function of temperature and
specific volume,
i.e. s = f(T,v)

From
the Maxwell Equations, we know that

This is known as the second form of entropy equation
or the second Tds equation
THE JOULE-THOMSON COEFFICIENT OF AN
IDEAL GAS IS ZERO
The
Joule-Thomson coefficient is defined as the change in temperature with change
in pressure, keeping the enthalpy remains constant. It is denoted by,
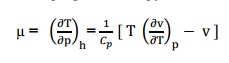
We
know that the equation of state as,
pV=RT
Differentiating
the above equation of state with respect to T by keeping pressure, p constant.
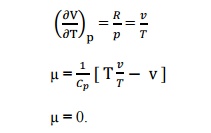
It
implies that the Joule-Thomson coefficient is zero for ideal gas.
CLAUSIUS–CLAPEYRON RELATION
The
Clausius–Clapeyron relation, named after Rudolf Clausius and Émile
Clapeyron, who defined it sometime after 1834, is a way of characterizing the
phase transition between two phases of matter, such as solid and liquid. On a
pressure–temperature (P–T) diagram, the line separating the two phases is known
as the coexistence curve. The Clausius–Clapeyron relation gives the slope of
this curve. Mathematically,
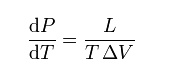
where dP / dT
is the slope of the coexistence curve, L is the latent heat, T is
the temperature,Visthevolumeandchange of the phase transition.
Pressure
Temperature Relations
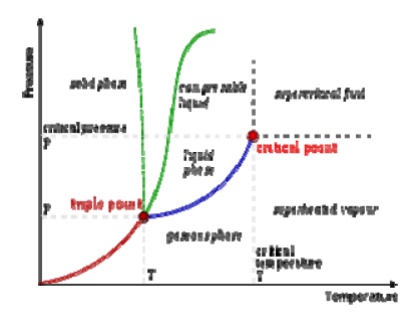
A typical phase
diagram. The dotted line gives the anomalous behavior of water. The
Clausius–Clapeyron relation can be used to (numerically) find the relationships
between pressure and temperature for the phase change boundaries. Entropy and
volume changes (due to phase change) are orthogonal to the plane of this
drawing
Derivation
Using
the state postulate, take the specific entropy, s, for a homogeneous
substance to be a function of specific volume, v, and temperature, T.
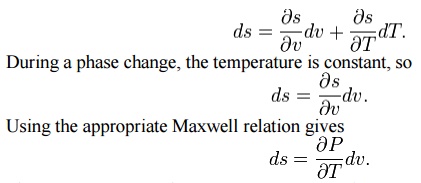
Since
temperature and pressure are constant during a phase change, the
derivative of pressure with respect to temperature is not a function of the
specific volume. Thus the partial derivative may be changed into a total
derivative and be factored out when taking an integral from one phase to
another,

Del is
used as an operator to represent— final (2) minus initial (1) For a closed
system undergoing an internally reversible process, the first law is
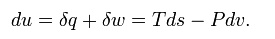
This
leads to a version of the Clausius–Clapeyron equation that is simpler to
integrate:
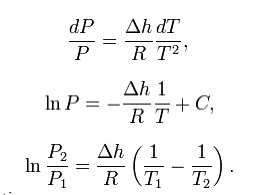
C is a constant of
integration
These
last equations are useful because they relate saturation pressure and
saturation temperature to the enthalpy of phase change, without requiring
specific volume data. Note that in this last equation, the subscripts 1 and 2
correspond to different locations on the pressure versus temperature phase
lines. In earlier equations, they corresponded to different specific volumes
and entropies at the same saturation pressure and temperature.
The Joule–Thomson (Kelvin) coefficient
The rate of change of
temperature T with respect to pressure P in a Joule– Thomson process (that is,
at constant enthalpy H) is the Joule–Thomson (Kelvin) coefficient μJT. Thisin
termscoefficientofthegas'svolumeV, can
its
heat capacity at constant pressure Cp, a as
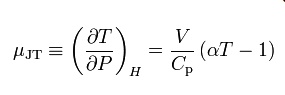
See the Appendix for
the proof of thi expressed in °C/bar (SI units: K/Pa) and depends on the type
of gas and on the temperature and pressure of the gas before expansion.
All real gases have an
inversion point at which the value of sign. The temperature of this point, the
Joule–Thomson inversion temperature,
depends
on the pressure of the gas before expansion. In a gas expansion the pressure
decreases, so the sign of is always negative. With that in mind, the following
table explains when the Joule–Thomson effect cools or warms a real gas:

Helium and hydrogen are two gases whose
Joule–Thomson inversion temperatures
at a pressure of one
atmosphere forare v helium). Thus, helium and hydrogen warm up when expanded at
constant enthalpy
at
typical room temperatures. On the other hand nitrogen and oxygen, the two most
abundant gases in air, have inversion temperatures of 621 K (348 °C) and 764 K
(491 °C) respectively: these gases can be cooled from room temperature by the
Joule–Thomson effect.
Derivation of the Joule–Thomson (Kelvin)
coefficent
A
derivation of the formula
for the Joule–Thomson (Kelvin) coefficient.
The
partial derivative of T with respect to P at constant H can be computed by
expressing the differential of the enthalpy dH in terms of dT and dP, and
equating the resulting expression to zero and solving for the ratio of dT and
dP. It follows from the fundamental thermodynamic relation that the
differential of the enthalpy is given by:
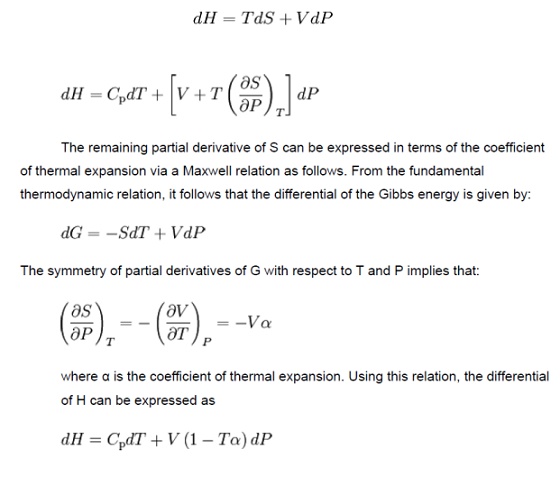
SOLVED PROBLEMS
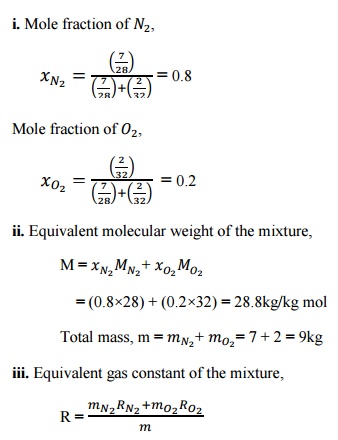
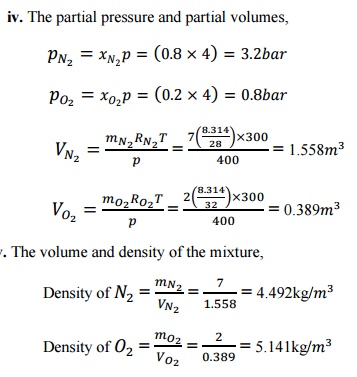
1. A
mixture of ideal gases consists of 7kg ofand 2kg ofat a pressure of 4bar and a temperature of 27°C. Determine:
i.
Mole fraction of each constituent,
ii.
Equivalent molecular weight of the
mixture,
iii.
Equivalent gas constant of the
mixture,
iv.
The partial pressure and partial
volumes,
v.
The volume and density of the
mixture
Given
data:
= 7kg
= 2kg
p = 4bar T = 27°C
Solution:
i.
Mole
fraction of ,
Describe
Joule Kelvin effect with the help of T-p diagram
The Joule Kelvin effect or Joule Thomson effect is
an efficient way of cooling gases. In this, a gas is made to undergo a
continuous throttling process. A constant pressure is maintained at one side of
a porous plug and a constant lower pressure at the other side. The apparatus is
thermally insulated so that the heat loss can be measured.
Joule
–Thomson co –efficient is defined as the change in temperature with change in
pressure, keeping the enthalpy remains constant. It is denoted by,
Throttling
process:
It is defined as the
fluid expansion through a minute orifice or slightly opened valve. During this
process, pressure and velocity are reduced. But there is no heat transfer and
no work done by the system. In this process enthalpy remains constant.
Joule
Thomson Experiment:
The
figure shows the arrangement of porous plug experiment. In this experiment, a
stream of gas at a pressure and temperature is allowed to flow continuously
through a porous pig. The gas comes out from the other side of the porous pig
at a pressure and temperature .
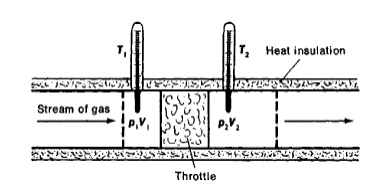
The whole apparatus is insulated. Therefore no heat
transfer takes place. Q = 0.
The system does not exchange work with the
surroundings.
So,
W=0 from steady flow energy equation we know that
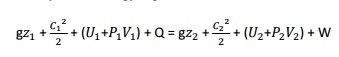
Since
there is no considerable change velocity,
and , Q=0,W=0, are applied in steady
flow energy equation. Therefore, It indicates that the enthalpy is
constant for throttling process.
It
is assumed that a series of experiments performed on a real gas keeping the
initial pressure p1 and temperature T1 constant with various down steam
pressures ( p2,p3..... ). It is found that the down steam temperature also
changes. The results from these experiments can be plotted as enthalpy curve on
T-p plane.
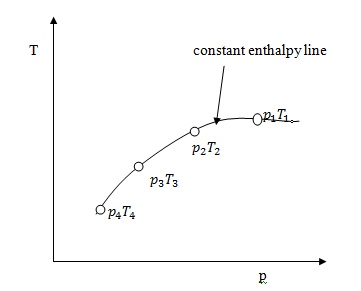
The
slope of a constant enthalpy is known as Joule Thomson Coefficient. It is
denoted by µ.
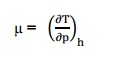
For
real gas, µ may be either positive or negative depending upon the thermodynamic
state of the gas.
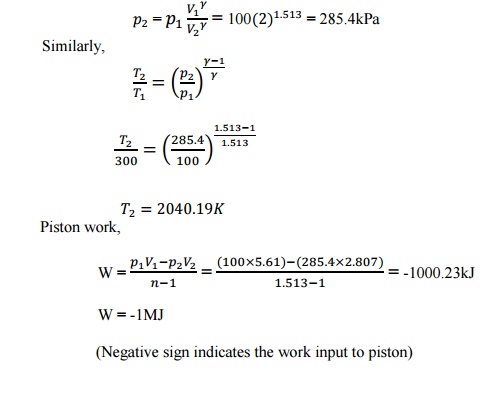
2. A
mixture of 2kg oxygen and 2kg Argon is in an insulated piston cylinder
arrangement at 100kPa, 300K. The piston now compresses the mixture to half its
initial volume. Molecular weight of oxygen is 40. Ratio of specific heats for
oxygen is 1.39 and for argon is 1.667.
Given
data:
=2kg =2kg
= 100kPa
= 300K
= 32
= 40
= =1.39
=1.667
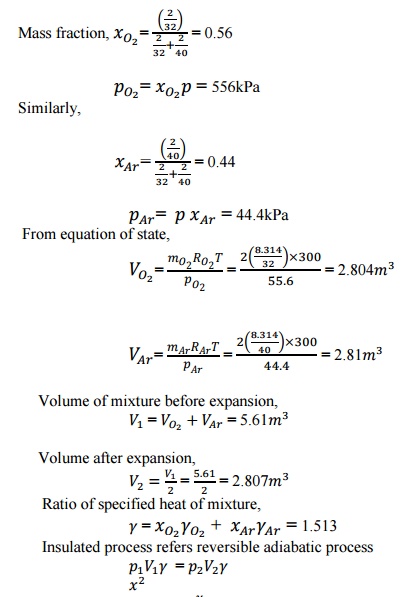
![]()
Related Topics