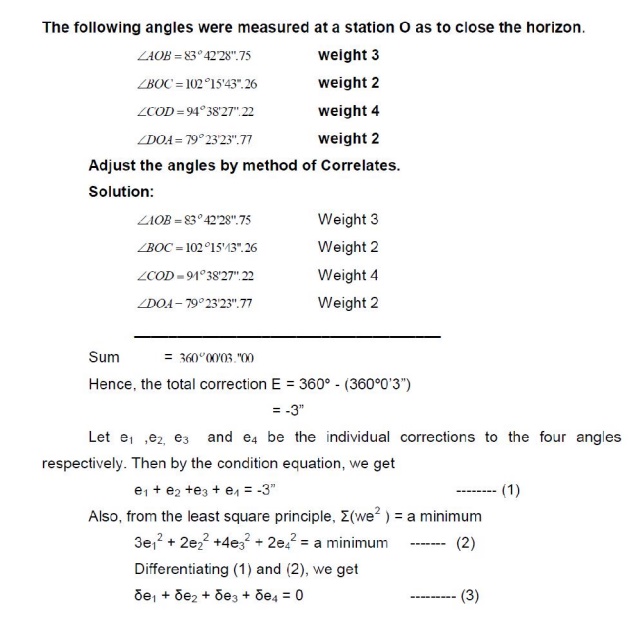
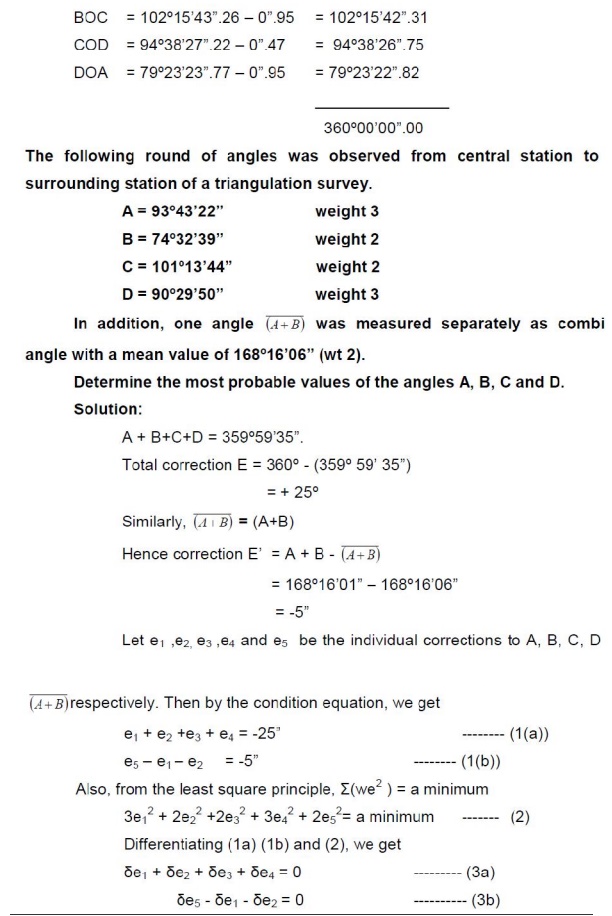
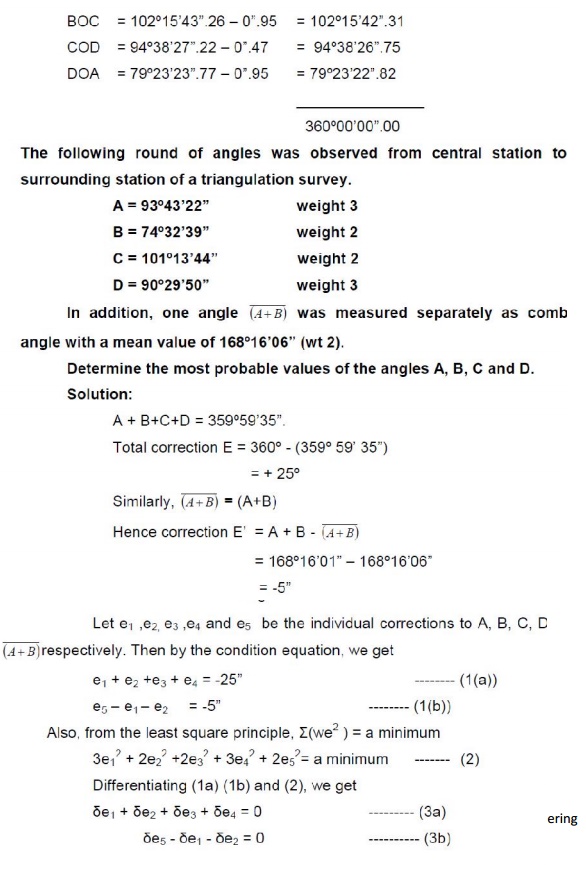
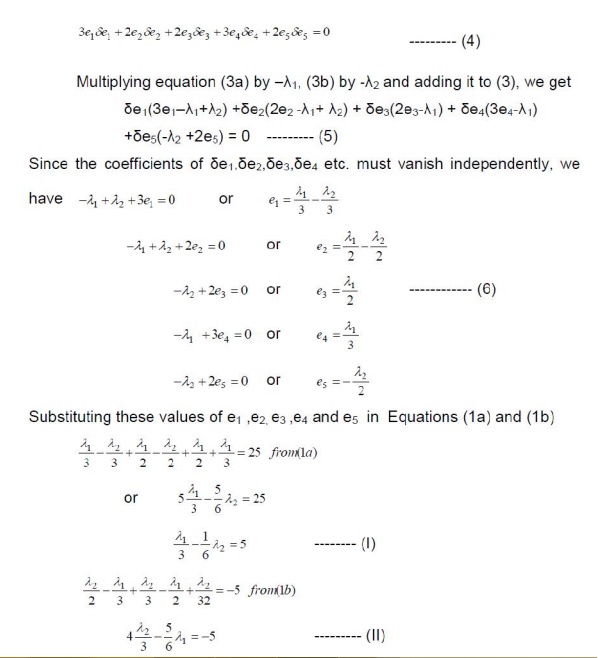
Chapter: Civil Surveying : Survey Adjustments
Distribution of Error of The Field Measurement
DISTRIBUTION OF ERROR OF THE FIELD MEASUREMENT
Whenever observations are made in
the field, it is always necessary to check for the closing error, if any. The
closing error should be distributed to the observed quantities. For examples,
the sum of the angles measured at a central angle should be 360 o , the error
should be distributed to the observed angles after giving proper weight age to
the observations. The following rules should be applied for the distribution of
errors:
(i).The correction to be applied to an observation
is inversely proportional to the weight of the observation.
(2) The
correction to be applied to an observation is directly proportional to the
square of the probable error.
(3) In case
of line of
levels, the correction
to be applied
is proportional to the
length.
The following are the three angles ?, ? and y
observed at a station P closing
the horizon, along with their probable errors of measurement.
Determine their corrected values.
Solution.
? = 78 o 12? 12'
±
2'
? = 136 o 48? 30' ± 4'
y = 144 o 59? 08' ± 5'
Sum of the three angles = 359 o 59? 50'
Discrepancy =
10'
Hence
each angle is to be increased, and the error of 10'
is to
be distributed in
proportion
to the square of the probable error.
Let c1, c2 and c3 be the correction to be applied
to the angles ?, ? and y respectively.
c1
: c2 : c3 = (2)² : (4)² : (5)² = 4 : 16 : 25 … (1)
Also, c1 + c2
+ c3 = 10' … (2)
From (1), c2
= 16 /4 c1 = 4c1
And c3
= 25/4 c1
Substituting these values of c2 and c3 in (2), we
get c1 +
4c1
+ 25/4 c1 = 10'
or c1 (
1 + 4 + 25/4 )= 10'
c1
= 10 x 4/45 = 0'.89
c2
= 4c1 = 3'.36
And c3 = 25 /4 c1
= 5'.55
Check: c1 + c2 + c3 = 0.'89 + 3'.56 + 5'.55 = 10'
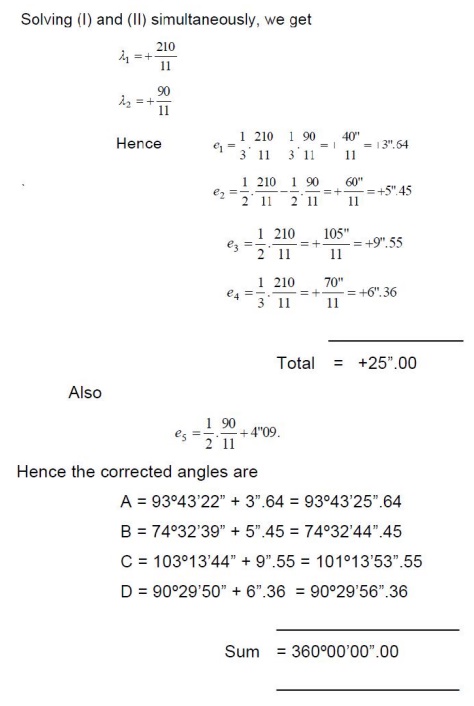
Hence the corrected angles are
?
= 78 o 12? 12'
+ 0'.89 = 78 o 12? 12'.89
?
=
136 o 48?
30'
+ 3'.56 = 136 o
48?
33'.56
and y = 144 o 59? 08'
+
5'.55 =
144 o 59? 13'.55
Sum = 360 o 00? 00'+ 00
An angle A was measured by different persons and
the following are the values
:
Angle Number of measurements
65 o
30? 10' … 2
65 o
29? 50' … 3
65 o
30? 00' … 3
65 o
30? 20' … 4
65 o
30? 10' … 3
Find the most probable value of the angle. Solution.
As stated
earlier, the most probable value of an angle is equal to its weighted
arithmetic mean.
65 o 30? 10'
x
2 = 131 o 00? 20'
65 o 29? 50'
x
3 = 196 o 29? 30'
65 o 30? 00'
x
3 = 196 o 30? 00'
65 o 30? 20'
x
4 = 262 o 01? 20'
65 o 30? 10'
x
3 = 196 o 30? 30'
Sum = 982 o 31? 40' ?
weight
= 2 + 3 + 3 + 4 + 3 = 15
Weighted
arithmetic mean
= 982 o 31? 40'
= 65 o 30? 6'.67
Hence most probable value of the angle = 65 o 30? 6'.67
The telescope of a theodilite is fitted with stadia wires. It
is required to find the most probable values of the constants C and K of
tacheometer. The staff was kept vertical at three points in the field and with
of sight horizontal the staff
intercepts observed was as follows.
Distance
of staff Staff intercept S(m)
from
tacheometer D( m)
150 1.495
200 2.000
250 2.505
Solution:
The
distance equation is
D = KS +
C
The
observation equations are
150 =
1.495 K + C
200 =
2.000 K + C
250 =
2.505 K + C
If K and
C are the most probable values, then the error of observations are:
150 -
1.495 K - C
200 -
2.000 K - C
250 -
2.505 K - C
By the theory of least squares
(150 -1.495 K -C)2
+(200 - 2.000 K- C )2 +(250 - 505 K - C )2
= minimum---(i)
For normal equation in K,
Differentiating
equation (i) w.r.t. K,
2(-1.495)(150
-1.495 K -C) +2(-20.)(200 - 2.000 K- C)
+2(-2.505)(250 - 505 K - C ) = 0
208.41667 - 2.085 K - C = 0 --------
(2)
Normal equation in C
Differentiating equation (i) w.r.t. C,
2(-1.0)(150 -1.495 K -C) +2(-1.0)(200 -
2.000 K- C)
+2(-1.0)(250 - 505 K - C ) = 0
200 - 2 K - C = 0 --------- (3)
On solving Equations (2) and (3)
K = 99.0196
C = 1.9608
The
distance equation is:
D = 99.0196 S + 1.9608
Related Topics