Oscillations | Physics - Linear Simple Harmonic Oscillator (LHO) | 11th Physics : UNIT 10 : Oscillations
Chapter: 11th Physics : UNIT 10 : Oscillations
Linear Simple Harmonic Oscillator (LHO)
LINEAR SIMPLE HARMONIC OSCILLATOR (LHO)
Horizontal oscillations of a spring-mass system
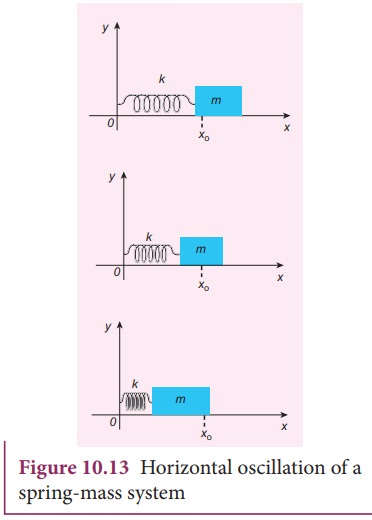
Consider a system containing a block of mass m attached to a massless spring with
stiffness constant or force constant or spring constant k placed on a smooth horizontal surface (frictionless surface) as
shown in Figure 10.13. Let x0
be the equilibrium position or mean position of mass m when it is left
undisturbed. Suppose the mass is displaced through a small displacement x towards right from its equilibrium
position and then released, it will oscillate back and forth about its mean
position x0. Let F be the restoring force (due to
stretching of the spring) which is
proportional to the amount of displacement of block. For one dimensional
motion, mathematically, we have
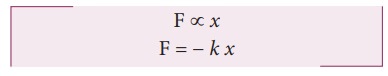
where negative sign implies that the restoring force
will always act opposite to the direction of the displacement. This equation is
called Hooke’s law. Notice that, the restoring force is linear with the
displacement (i.e., the exponent of force and displacement are unity). This is
not always true; in case if we apply a very large stretching force, then the
amplitude of oscillations becomes very large (which means, force is
proportional to displacement containing higher powers of x) and therefore, the
oscillation of the system is not linear and hence, it is called non-linear
oscillation. We restrict ourselves only to linear oscillations throughout our
discussions, which means Hooke’s law is valid (force and displacement have a
linear relationship).
From Newton’s second law, we can write the equation
for the particle executing simple harmonic motion
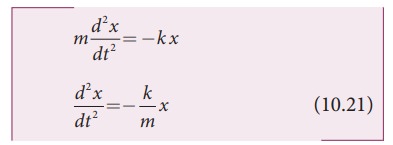
Comparing the equation (10.21) with simple harmonic
motion equation (10.10), we get

which means the angular frequency or natural frequency
of the oscillator is
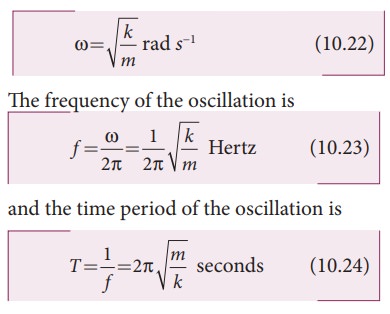
Notice
that in
simple harmonic motion, the time period of oscillation is independent of
amplitude. This is valid only if the amplitude of oscillation is small.
The
solution of the differential equation of a SHM may be written as
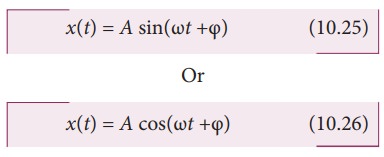
where A, ω
and ϕ are constants. General solution for differential equation 10.21 is x(t)
= A sin(ωt +φ)+ B cos(ωt +φ) where A and B are contants.
Vertical oscillations of a spring
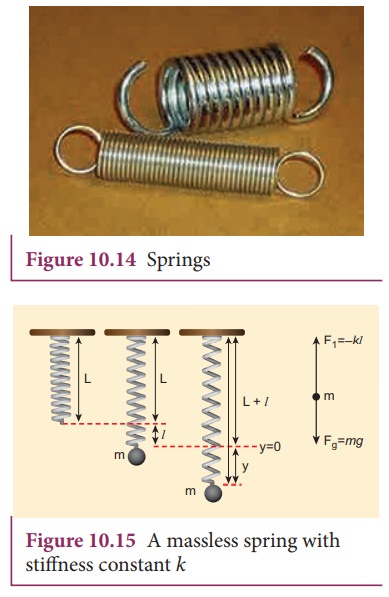
Let us consider a massless spring with stiffness
constant or force constant k attached
to a ceiling as shown in Figure 10.15. Let the length of the spring before
loading mass m be L. If the block of mass m is attached to the other end of
spring, then the spring elongates by a length l. Let F1 be the restoring force due to stretching of
spring. Due to mass m, the
gravitational force acts vertically downward. We can draw free-body diagram for
this system as shown in Figure 10.15. When the system is under equilibrium,

But the spring elongates by small displacement l, therefore,
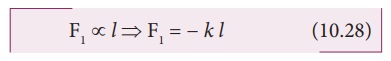
Substituting equation (10.28) in equation (10.27), we
get
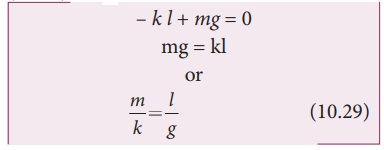
Suppose we apply a very small external force on the
mass such that the mass further displaces downward by a displacement y, then it will oscillate up and down.
Now, the restoring force due to this stretching of spring (total extension of
spring is y + l ) is
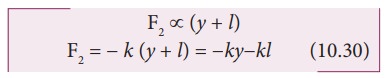
Since, the mass moves up and down with acceleration d2y/dt2,
by drawing the free body, diagram for this case, we get
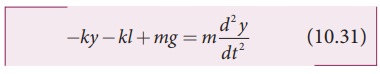
The net force acting on the mass due to this
stretching is
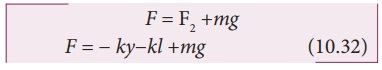
The
gravitational force opposes the restoring force. Substituting equation (10.29)
in equation (10.32), we get
F = −ky − kl +
kl = −ky
Applying
Newton’s law, we get
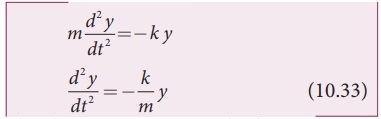
The above equation is in the form of simple harmonic
differential equation. Therefore, we get the time period as

The time period can be rewritten using equation
(10.29)
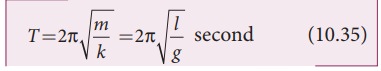
The acceleration due to gravity g can be computed from the formula
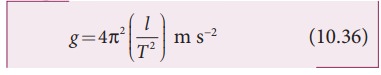
EXAMPLE 10.8
A
spring balance has a scale which ranges from 0 to 25 kg and the length of the
scale is 0.25m. It is taken to an unknown planet X where the acceleration due
to gravity is 11.5 m s−1. Suppose a body of mass M kg is suspended
in this spring and made to oscillate with a period of 0.50 s. Compute the
gravitational force acting on the body.
Solution
Let us first calculate the stiffness constant of the
spring balance by using equation (10.29),
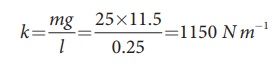
The time period of oscillations is given by T=2π√M/√k, , where M is the mass of the body.
Since, M is unknown, rearranging, we get

The gravitational force acting on the body is W = Mg =
7.3 × 11.5 = 83.95 N ≈ 84 N
Combinations of springs

Spring
constant or force constant, also called as stiffness constant, is a measure of
the stiffness of the spring. Larger the value of the spring constant, stiffer
is the spring. This implies that we need to apply more force to compress or
elongate the spring. Similarly, smaller the value of spring constant, the
spring can be stretched (elongated) or compressed with lesser force. Springs
can be connected in two ways. Either the springs can be connected end to end,
also known as series connection, or alternatively, connected in parallel. In
the following subsection, we compute the effective spring constant when
a.
Springs are connected in series
b.
Springs are connected in parallel
a. Springs connected in series
When two or more springs are connected in series, we
can replace (by removing) all the springs in series with an equivalent spring
(effective spring) whose net effect is the same as if all the springs are in
series connection. Given the value of individual spring constants k1, k2, k3,...
(known quantity), we can establish a
mathematical relationship to find out an effective (or equivalent) spring
constant ks (unknown
quantity). For simplicity, let us consider only two springs whose spring
constant are k1 and
k2 and which can be
attached to a mass m as shown in Figure 10.17. The results
thus obtained can be generalized for any number of springs in series.
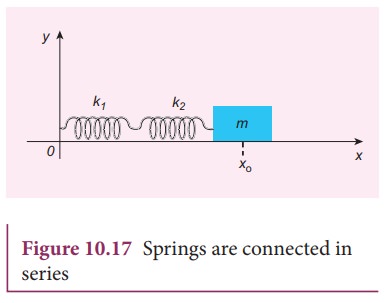
Let F be the
applied force towards right as shown in Figure 10.18. Since the spring
constants for different spring are different and the connection points between
them is not rigidly fixed, the strings can stretch in different lengths. Let x1 and x2 be the elongation of springs from their equilibrium
position (un-stretched position) due to the applied force F. Then, the net displacement of the mass point is

From Hooke’s law, the net force
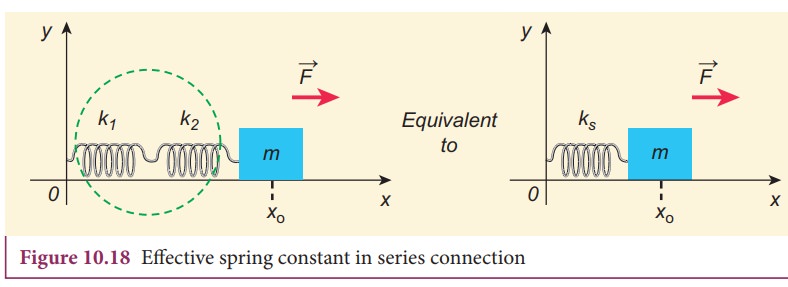
For springs in series connection
−k1x1 = −k2x2 = F
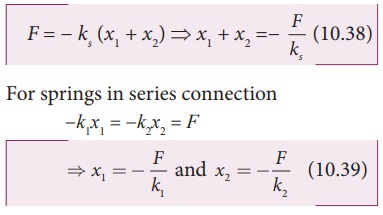
Therefore, substituting equation (10.39) in equation
(10.38), the effective spring constant
can be calculated as
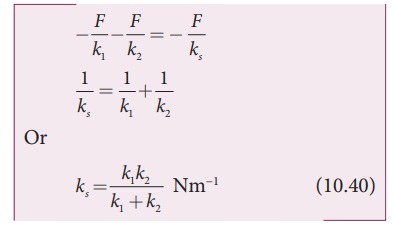
Suppose we have n
springs connected in series, the effective spring constant in series is
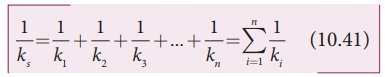
If all spring constants are identical i.e., k1 = k2 =... = kn = k then

This means that the effective spring constant reduces
by the factor n. Hence, for springs in series connection, the effective
spring constant is lesser than the individual spring constants.
From
equation (10.39), we have,
k1x1 = k2x2
Then
the ratio of compressed distance or elongated distance x1 and x2
is

The
elastic potential energy stored in first and second springs are V1=1/2
k1x12 and V2=1/2 k2x22
respectively. Then, their ratio is
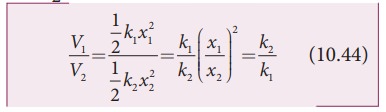
EXAMPLE 10.9
Consider two springs whose force constants are 1 N m−1
and 2 N m−1 which are connected in series. Calculate the effective
spring constant (ks ) and
comment on ks .
Solution
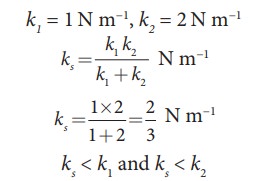
ks < k1 and ks < k
Therefore,
the effective spring constant is lesser than both k1 and k2.
b. Springs connected in parallel
When two or more springs are connected in parallel, we
can replace (by removing) all these springs with an equivalent spring
(effective spring) whose net effect is same as if all the springs are in
parallel connection. Given the values of individual spring constants to be k1,k2,k3,
... (known quantities), we can establish a mathematical relationship to find out
an effective (or equivalent) spring constant kp (unknown
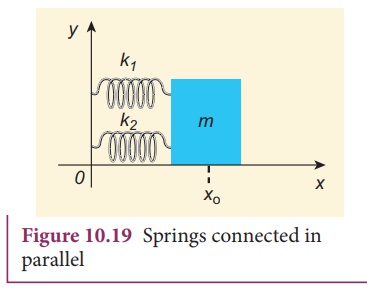
quantity). For simplicity, let us consider
only two springs of spring constants k1and k2 attached to a mass m as
shown in Figure 10.19. The results
can be generalized to any number of springs in parallel.
Let the force F
be applied towards right as shown in Figure 10.20. In this case, both the
springs elongate or compress by the same amount of displacement. Therefore, net
force for the displacement of mass m
is

where kp
is called effective spring constant. Let the first spring be elongated by
a displacement x due to force F1 and second spring be
elongated by the same displacement x due
to force F2, then the net
force
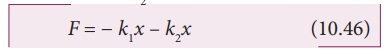
Equating equations (10.46) and (10.45), we get

Generalizing, for n springs connected in parallel,

If all spring constants are identical i.e., k1 = k2= ... = kn = k then

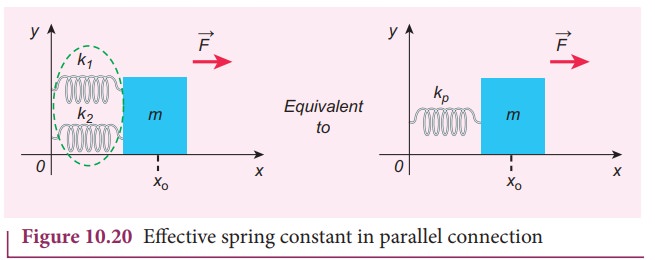
This implies that the effective spring constant increases by a factor n. Hence, for the springs in parallel connection, the effective spring constant is greater than individual spring constant.
EXAMPLE 10.10
Consider
two springs with force constants 1 N m−1 and 2 N m−1
connected in parallel. Calculate the effective spring constant (kp ) and comment on kp.
Solution
k1 = 1 N m−1, k2 = 2 N m−1
kp =
k1 + k2 N m−1
kp = 1 + 2 = 3 N m−1
kp > k1 and kp > k2
Therefore,
the effective spring constant is greater than both k1 and k2.
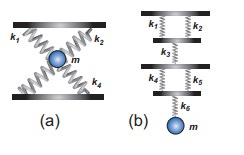
EXAMPLE 10.11
Calculate the equivalent spring constant for the
following systems and also compute if all the spring constants are equal:
Solution
a.
Since k1 and k2 are parallel, ku = k1 + k2
Similarly, k3 and k4 are parallel, therefore, kd = k3 + k4
But
ku and kd are in series,
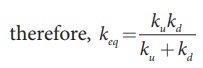
If
all the spring constants are equal then, k1
= k2 = k3 = k4 = k
Which
means, ku = 2k and kd = 2k
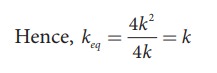
b.
Since k1 and k2 are parallel, kA = k1 + k2
Similarly, k4 and k5 are parallel, therefore, kB = k4 + k5
But
kA, k3, kB,
and k6 are in series,
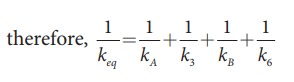
If all the spring constants are equal then, k1 = k2 = k3
= k4 = k5 = k6 = k which
means, kA = 2k and kB = 2k
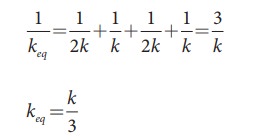
keq
= k/3
EXAMPLE 10.12
A
mass m moves with a speed v on a horizontal smooth surface and
collides with a nearly massless spring whose spring constant is k. If the mass stops after collision,
compute the maximum compression of the spring.
Solution
When
the mass collides with the spring, from the law of conservation of energy “the
loss in kinetic energy of mass is gain in elastic potential energy by spring”.
Let
x be the distance of compression of
spring, then the law of conservation of energy
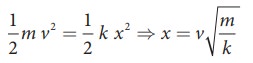
Oscillations of a simple pendulum in SHM and laws of simple pendulum
Simple pendulum
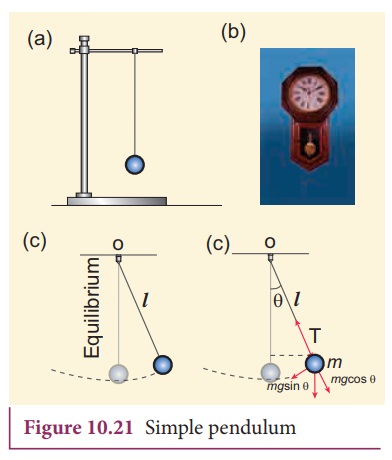
A
pendulum is a mechanical system which exhibits periodic motion. It has a bob
with mass m suspended by a long string (assumed to be massless and inextensible
string) and the other end is fixed on a stand as shown in Figure 10.21 (a). At
equilibrium, the pendulum does not oscillate and hangs vertically downward.
Such a position is known as mean position or equilibrium position. When a
pendulum is displaced through a small displacement from its equilibrium position
and released, the bob of the pendulum executes to and fro motion. Let l be the length of the pendulum which is
taken as the distance between the point of suspension and the centre of gravity
of the bob. Two forces act on the bob of the pendulum at any displaced
position, as shown in the Figure 10.21 (d),
![]()
![]() (i) The gravitational force acting on the body (
(i) The gravitational force acting on the body (  ) which acts vertically downwards.
) which acts vertically downwards.
(ii)
The tension in the string ![]() which acts along the string to the point of suspension.
which acts along the string to the point of suspension.
Resolving
the gravitational force into its components:
a. Normal component: The component along the string but in opposition to the direction of tension, Fas = mg cosθ.
b. Tangential component: The component perpendicular to the string i.e., along tangential
direction of arc of swing, Fps = mg sinθ.
Therefore,
The normal component of the force is, along the string,

From the Figure 10.21, we can observe that the
tangential component Wps
of the gravitational force always points towards the equilibrium position i.e.,
the direction in which it always points opposite to the direction of
displacement of the bob from the mean position. Hence, in this case, the
tangential force is nothing but the restoring force. Applying Newton’s second
law along tangential direction, we have
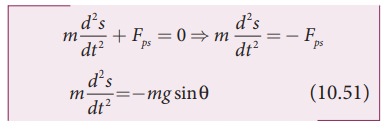
where, s is
the position of bob which is measured along the arc. Expressing arc length in
terms of angular displacement i.e.,

Substituting equation (10.53) in equation (10.51), we
get

Because of the presence of sin θ in the above differential equation, it is a non-linear
differential equation (Here, homogeneous second order). Assume “the small
oscillation approximation”, sin θ ≈ θ, the above differential equation
becomes linear differential equation.

This is the well known oscillatory differential
equation. Therefore, the angular frequency of this oscillator (natural
frequency of this system) is
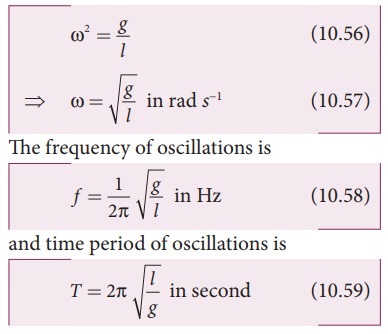
Laws of simple pendulum
The
time period of a simple pendulum
a. Depends on the following laws
(i) Law of length
For
a given value of acceleration due to gravity, the time period of a simple
pendulum is directly proportional to the square root of length of the pendulum.

(ii) Law of acceleration
For a fixed length, the time period of a simple
pendulum is inversely proportional to square root of acceleration due to
gravity.

b. Independent of the following factors
(i) Mass of the bob
The
time period of oscillation is independent of mass of the simple pendulum.
This is similar to free fall. Therefore, in a pendulum of fixed length, it does
not matter whether an elephant swings or an ant swings. Both of them will swing
with the same time period.
(ii) Amplitude of the oscillations
For a pendulum with small angle approximation (angular
displacement is very small), the time period is independent of amplitude of the
oscillation.
EXAMPLE 10.13
In simple pendulum experiment, we have used small
angle approximation . Discuss the small angle approximation.
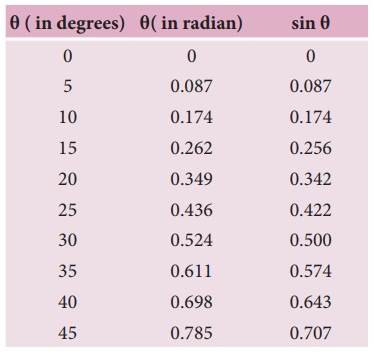
For θ in radian, sin θ ≈ θ for very small angles
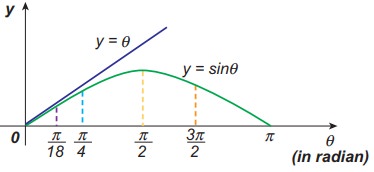
This
means that “for θ as large as 10
degrees, sin θ is nearly the same as θ when θ is expressed in radians”.
As θ increases in value sinθ gradually becomes different from θ
Pendulum length due to effect of temperature
Suppose
the suspended wire is affected due to change in temperature. The rise in
temperature affects length by
l = lo (1 +
α ∆t)
where
lo is the original length
of the wire and l is final length of
the wire when the temperature is raised. Let ∆t is the change in temperature and α is the co-efficient of linear expansion.
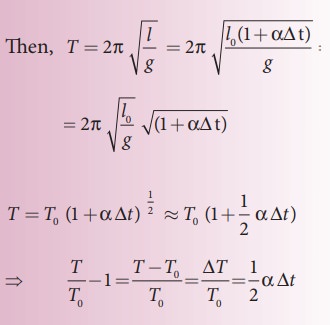
where ∆T is the change in time period due to the
effect of temperature and T0
is the time period of the simple pendulum with original length l0.
EXAMPLE 10.14
If
the length of the simple pendulum is increased by 44% from its original length,
calculate the percentage increase in time period of the pendulum.
Solution
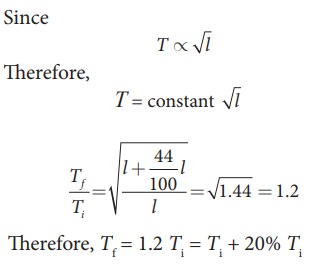
Oscillation
of liquid in a U-tube:
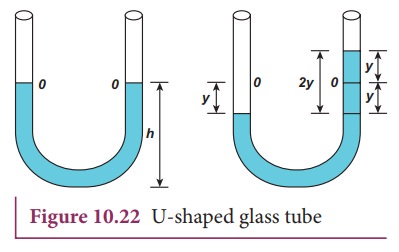
Consider a U-shaped glass tube which consists of two
open arms with uniform cross-sectional area A. Let us pour a non-viscous
uniform incompressible liquid of density ρ in the U-shaped tube to a height h as shown in the Figure 10.22. If the liquid and tube are not
disturbed then the liquid surface will be in equilibrium position O. It means the pressure as measured at
any point on the liquid is the same and also at the surface on the arm (edge of
the tube on either side), which balances with the atmospheric pressure. Due to
this the level of liquid in each arm will be the same. By blowing air one can
provide sufficient force in one arm, and the liquid gets disturbed from
equilibrium position O, which means,
the pressure at blown arm is higher than the other arm. This creates difference
in pressure which will cause the liquid to oscillate for a very short duration
of time about the mean or equilibrium position and finally comes to rest.
Time period of the oscillation is
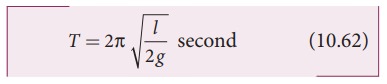
Related Topics